Calculate packing efficiency in body-centred cubic structure.

Text Solution

Verified by Experts

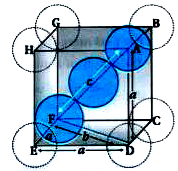
In a body-centred unit cell, the spheres located at the corners donot touch each other but they are in contact with body centred atom.
In $△ E F D$
$b^{2} = a^{2} + a^{2}$
$2 a^{2}$
$b = \sqrt{2} a$ .
In $△ A F D$
$c^{2} = a^{2} + b^{2}$
$= a^{2} + 2 a^{2}$
$= 3 a^{2}$
$c = \sqrt{3} a$
The length of the body diagonal c is equal to 4r, where r is the radius of sphere, as all three spheres along the diagonal touch each other. Therefore, $\sqrt{3} a = 4 r$
$a = \frac{4 r}{\sqrt{3}}$
Or $r = \frac{\sqrt{3}}{4} a$
In BCC, the total number of atoms per unit cell are two. Hence the total volume of two atoms is $(2 \times \frac{4}{3} π r^{3})$
Volume of the cube = $a^{3} = {(\frac{4}{\sqrt{3}} r)}^{3}$
% Packing efficiency = $\frac{Volume occupied by four spheres in unit cell \times 100}{Volume of the unit cell}$
$= \frac{2 \times (\frac{4}{3}) π r^{3} \times 100}{{(\frac{4}{\sqrt{4}} r)}^{3}} = 68 %$ .

Updated on:21/07/2023

The packing efficiency for a body centred cubic structure is
A $0.42$
B $0.53$
C $0.68$
D $0.82$
Percentage of packing fraction in cubic close packed structure and in body centered packed structure are respectively.
A $0.42$
B $0.53$
C $0.68$
D $0.82$
The packing fraction for a body-centred cube is
A0.42
B $0.53$
C $0.68$
D $0.82$