For radial probability curves. Which of the following is/are correct ?
The number of maxima in 2s orbital are two
The number of spherical or radial nodes is equal to n −l−1
The number of angular nodes are 'l'
3dz2has 3 angular nodes
The correct Answer is:A,B,C
To solve the question regarding the radial probability curves and determine which statements are correct, we will analyze each statement step by step.
Step 1: Understand the 2s Orbital
- The 2s orbital has a principal quantum number (n) of 2.
- The formula for the total number of nodes in an orbital is n−1.
- For the 2s orbital, the total number of nodes is 2−1=1.
Hint: Remember that the total number of nodes is derived from the principal quantum number.
Step 2: Analyze the Number of Maxima in the 2s Orbital
- The radial probability curve for the 2s orbital shows that there are two maxima.
- This means that there are two positions where the probability of finding an electron is highest.
Hint: Visualizing the radial probability curve can help in understanding the maxima.
Step 3: Check the Formula for Radial Nodes
- The formula for the number of radial nodes is n−l−1, where l is the azimuthal quantum number.
- For the 2s orbital, l=0 (since s orbitals have l=0).
- Thus, the number of radial nodes is 2−0−1=1.
Hint: The azimuthal quantum number l varies with the type of orbital (s, p, d, f).
Step 4: Evaluate Angular Nodes
- The number of angular nodes is equal to l.
- For the s orbital, l=0, so there are 0 angular nodes.
- For p orbitals, l=1 (1 angular node), and for d orbitals, l=2 (2 angular nodes).
Hint: The number of angular nodes is directly related to the type of orbital.
Step 5: Analyze the Statement about 3d2
- The statement claims that the 3d2 orbital has 3 angular nodes.
- For d orbitals, l=2, which means there are 2 angular nodes, not 3.
- Therefore, this statement is incorrect.
Hint: Remember that the number of angular nodes is equal to the azimuthal quantum number l.
Conclusion
Based on the analysis:
- The first statement about the 2s orbital having 2 maxima is true.
- The second statement regarding the formula for radial nodes n−l−1 is true.
- The third statement about the number of angular nodes being l is true.
- The last statement about 3d2 having 3 angular nodes is false.
Thus, the correct statements are A, B, and C.
Final Answer
The correct statements regarding the radial probability curves are:
- A: The number of maxima in a 2s orbital are 2.
- B: The number of spherical or radial nodes is equal to n−l−1.
- C: The number of angular nodes are l.
Incorrect Statement:
- D: 3d2 has 3 angular nodes (this is false; it has 2 angular nodes).
- The 2s orbital has a principal quantum number (n) of 2.
- The formula for the total number of nodes in an orbital is n−1.
- For the 2s orbital, the total number of nodes is 2−1=1.
- The radial probability curve for the 2s orbital shows that there are two maxima.
- This means that there are two positions where the probability of finding an electron is highest.
- The formula for the number of radial nodes is n−l−1, where l is the azimuthal quantum number.
- For the 2s orbital, l=0 (since s orbitals have l=0).
- Thus, the number of radial nodes is 2−0−1=1.
- The number of angular nodes is equal to l.
- For the s orbital, l=0, so there are 0 angular nodes.
- For p orbitals, l=1 (1 angular node), and for d orbitals, l=2 (2 angular nodes).
- The statement claims that the 3d2 orbital has 3 angular nodes.
- For d orbitals, l=2, which means there are 2 angular nodes, not 3.
- Therefore, this statement is incorrect.
Based on the analysis:
- The first statement about the 2s orbital having 2 maxima is true.
- The second statement regarding the formula for radial nodes n−l−1 is true.
- The third statement about the number of angular nodes being l is true.
- The last statement about 3d2 having 3 angular nodes is false.
The correct statements regarding the radial probability curves are:
- A: The number of maxima in a 2s orbital are 2.
- B: The number of spherical or radial nodes is equal to n−l−1.
- C: The number of angular nodes are l.
- D: 3d2 has 3 angular nodes (this is false; it has 2 angular nodes).
Similar Questions
Which of the following curves is correct?
View SolutionV vs T curves at constant pressure P1 and P2 for an ideal gas are shown in the figure. Which of the following is correct?

View SolutionThe temperature change versus heat supplied curve is given for 1 kg of a solid block. Then, which of the following statement is/are correct ?
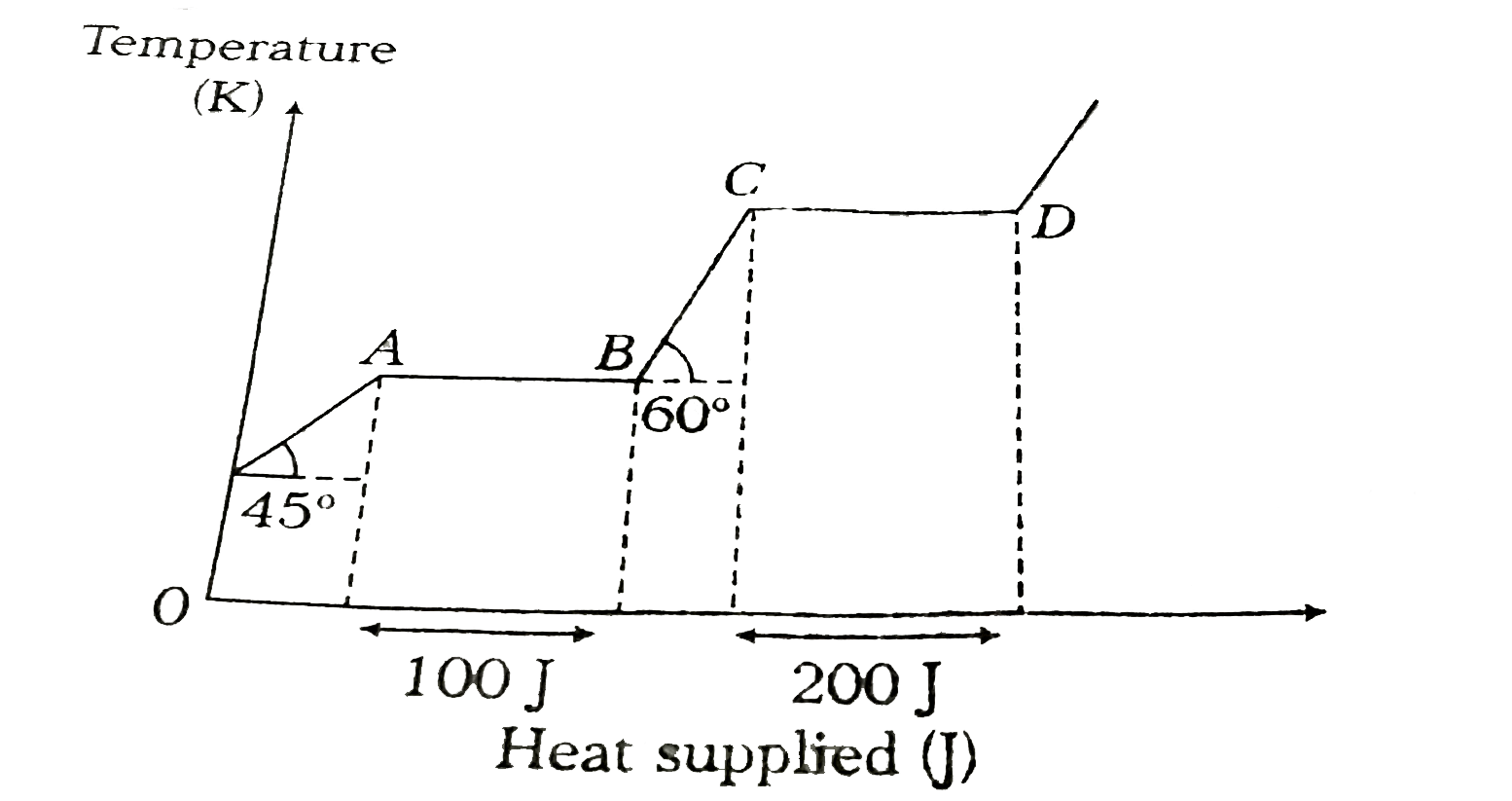
View SolutionV vs T curves at different pressures P1 and P2 for an ideal gas are shown below:
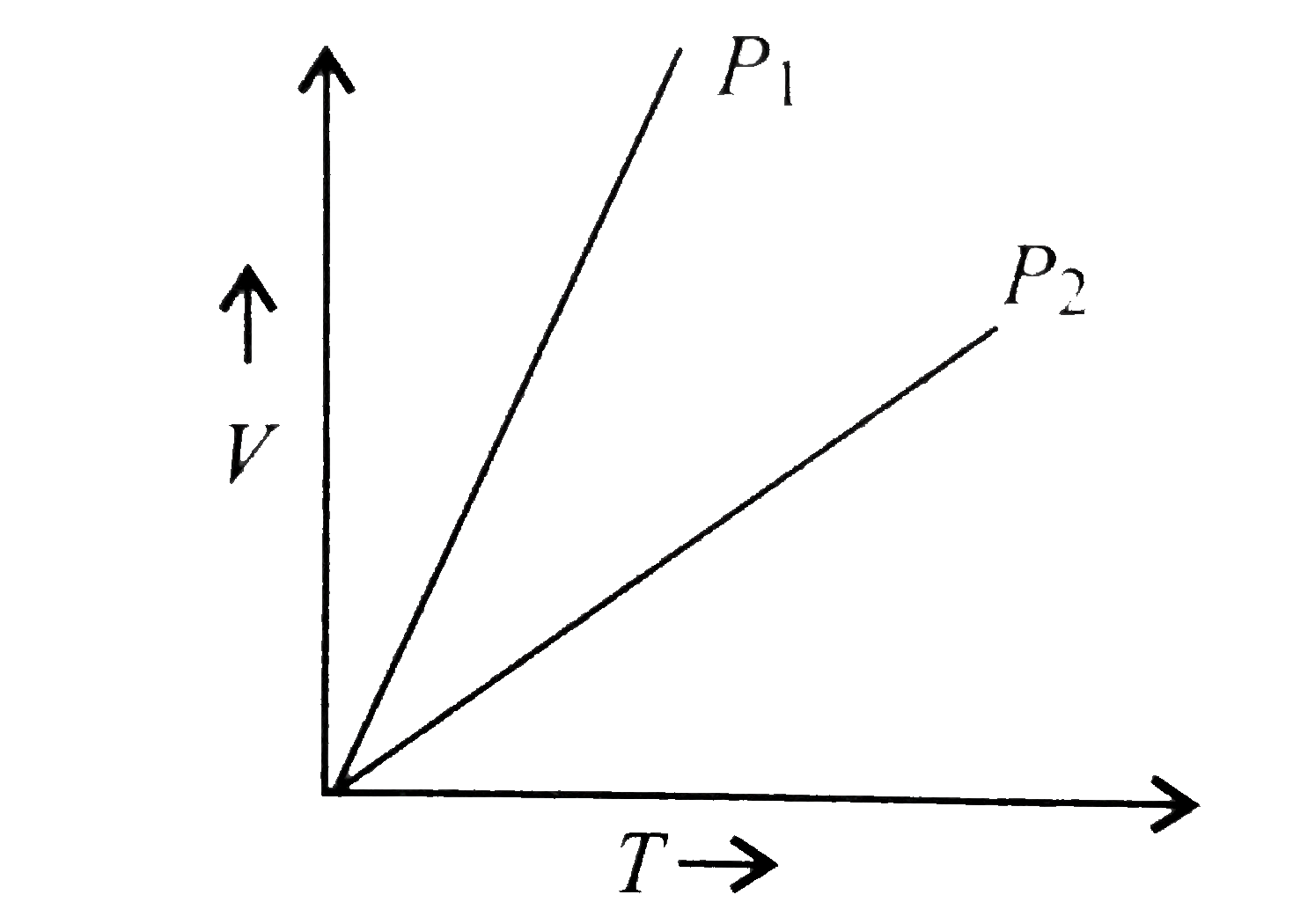
Which one of the following is correct?
View SolutionOrbitals are the pictorial representationofΨ or Ψ2

Ψ2 tell about the probability of finding electron.
Which of the following graph is correct for 3p ?
View SolutionFigure shows the displacement -time curve of the particles P and Q. Which of the following statement is correct ?
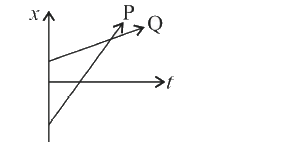
View SolutionFor the curve y=cex/a, which one of the following is incorrect?
View SolutionA ball is thrown downward with some velocity into a.viscous liquid. Which of the following curves represents correct variation for velocity versus time?
View SolutionConsider the following radial distribution function diagrams. Which of the following has the correct matching of curve and orbital?
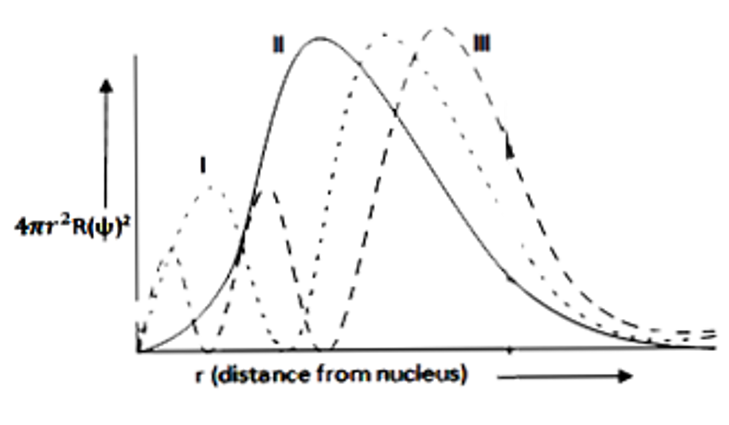
View SolutionWhich of the following plots of radial - probability function 4π2ψ2 is /are correct
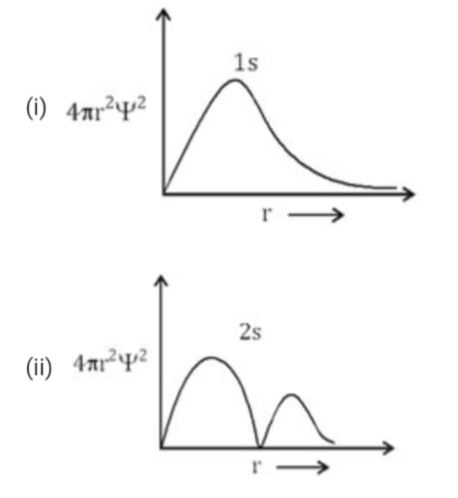
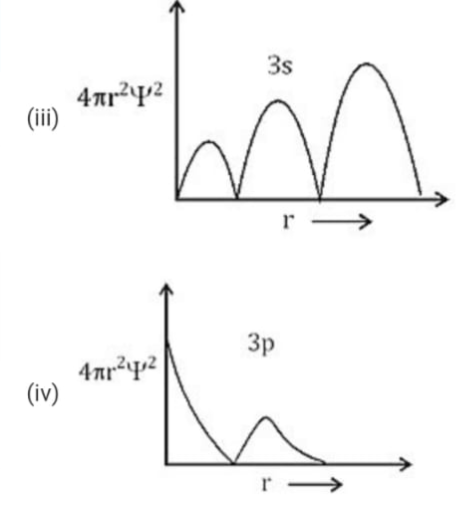
View Solution
