For the first order thermal decomposition reaction, the following data were obained :
C2H5Cl(g)→C2H4(g)+HCl(g)
Time/Sec Total pressure/atm0 0.30300 0.50
Calculate the rate constant
(Given : log 2= 0.301, log 3 = 0.4771, log 4=0.6021)
To calculate the rate constant for the first order thermal decomposition reaction C2H5Cl(g)→C2H4(g)+HCl(g), we will follow these steps:
Step 1: Understand the Reaction
The reaction involves the decomposition of ethyl chloride (C2H5Cl) into ethylene (C2H4) and hydrochloric acid (HCl).
Step 2: Gather Initial and Final Conditions
From the data provided:
- Initial pressure (P0) = 0.30 atm
- Total pressure at t=300 seconds (Pt) = 0.50 atm
Step 3: Determine Change in Pressure
Let x be the change in pressure due to the decomposition of C2H5Cl. The total pressure at any time t can be expressed as:
Pt=P0+x
Since each molecule of C2H5Cl that decomposes produces one molecule of C2H4 and one molecule of HCl, the change in pressure x will be equal to the increase in pressure due to the formation of the products.
From the total pressure data:
x=Pt−P0=0.50atm−0.30atm=0.20atm
Step 4: Calculate Initial and Final Concentrations
The initial pressure of C2H5Cl is P0=0.30atm and the final pressure of C2H5Cl after 300 seconds is:
PC2H5Cl=P0−x=0.30atm−0.20atm=0.10atm
Step 5: Use the First Order Rate Equation
For a first order reaction, the rate constant k can be calculated using the equation:
t=2.303klog(P0PC2H5Cl)
Substituting the values we have:
300=2.303klog(0.300.10)
Step 6: Calculate the Logarithm
Calculate the logarithm:
0.300.10=3⇒log(3)=0.4771
Step 7: Substitute and Solve for k
Now substituting back into the equation:
300=2.303k×0.4771
Rearranging to solve for k:
k=2.303×0.4771300
Step 8: Calculate k
Calculating k:
k=1.0987300≈0.00366atm−1s−1
Final Answer
The rate constant k for the reaction is approximately 0.00366atm−1s−1.
---
The reaction involves the decomposition of ethyl chloride (C2H5Cl) into ethylene (C2H4) and hydrochloric acid (HCl).
From the data provided:
- Initial pressure (P0) = 0.30 atm
- Total pressure at t=300 seconds (Pt) = 0.50 atm
Let x be the change in pressure due to the decomposition of C2H5Cl. The total pressure at any time t can be expressed as:
Pt=P0+x
Since each molecule of C2H5Cl that decomposes produces one molecule of C2H4 and one molecule of HCl, the change in pressure x will be equal to the increase in pressure due to the formation of the products.
x=Pt−P0=0.50atm−0.30atm=0.20atm
The initial pressure of C2H5Cl is P0=0.30atm and the final pressure of C2H5Cl after 300 seconds is:
PC2H5Cl=P0−x=0.30atm−0.20atm=0.10atm
For a first order reaction, the rate constant k can be calculated using the equation:
t=2.303klog(P0PC2H5Cl)
Substituting the values we have:
300=2.303klog(0.300.10)
Calculate the logarithm:
0.300.10=3⇒log(3)=0.4771
Now substituting back into the equation:
300=2.303k×0.4771
Rearranging to solve for k:
k=2.303×0.4771300
Calculating k:
k=1.0987300≈0.00366atm−1s−1
The rate constant k for the reaction is approximately 0.00366atm−1s−1.
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - C.B.S.E CLASS XII
37 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - DELHI BOARD SET II
8 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET-III
31 Videos
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION: D
1 Videos
Similar Questions
The following data were obtained during the first order thermal decomposition of SO2Cl2 at constant volume :
ExperimentTime (s)Total Pressure (atm)100.321000.5
Calculate rate constant
[Given : log 6 = 0.7782, log 3 = 0.4771]
View SolutionThe following data were obtained during the first thermal decompoistion of N2O5(g) at constant volume.
2N2O5(g)→2N2O4(g)+O2(g)
∣∣ ∣ ∣∣S.No.Time (s)Total pressure (atm)i.00.5ii.1000.512∣∣ ∣ ∣∣
Calculate the rate constant.
View Solution
Knowledge Check
The following data were obtained during the first order thermal decomposition of SO2CI2 at a constant volume.
SO2CI2(g)→SO2(g)+CI2(g)
ExperimentTime/s−1Total pressure/atm100.521000.6
What is the rate of reaction when total pressure is 0.65 atm?A0.35atms−1B2.235×10−3atms−1C7.8×10−4atms−1D1.55×10−4atms−1
The following data is obtained during the first order thermal decomposition of
2A(g)→B(g)+C(s) at constant volume and temperature
S.No.TimeTotal pressure1.At the end of 10 minutes3002.After completion200
The rate constant in min−1 is
View SolutionThe following data is obtained during the first order thermal decomposition of
2A(g)→B(g)+C(s) at constant volume and temperature
S.No.TimeTotal pressure1.At the end of 10 minutes3002.After completion200
The rate constant in min−1 is
View SolutionThe following data were obtained during the first order thermal decomposition of N2O5 (g) at constant volume :
2N2O5(g)→2N2O4(g)+O2(g)
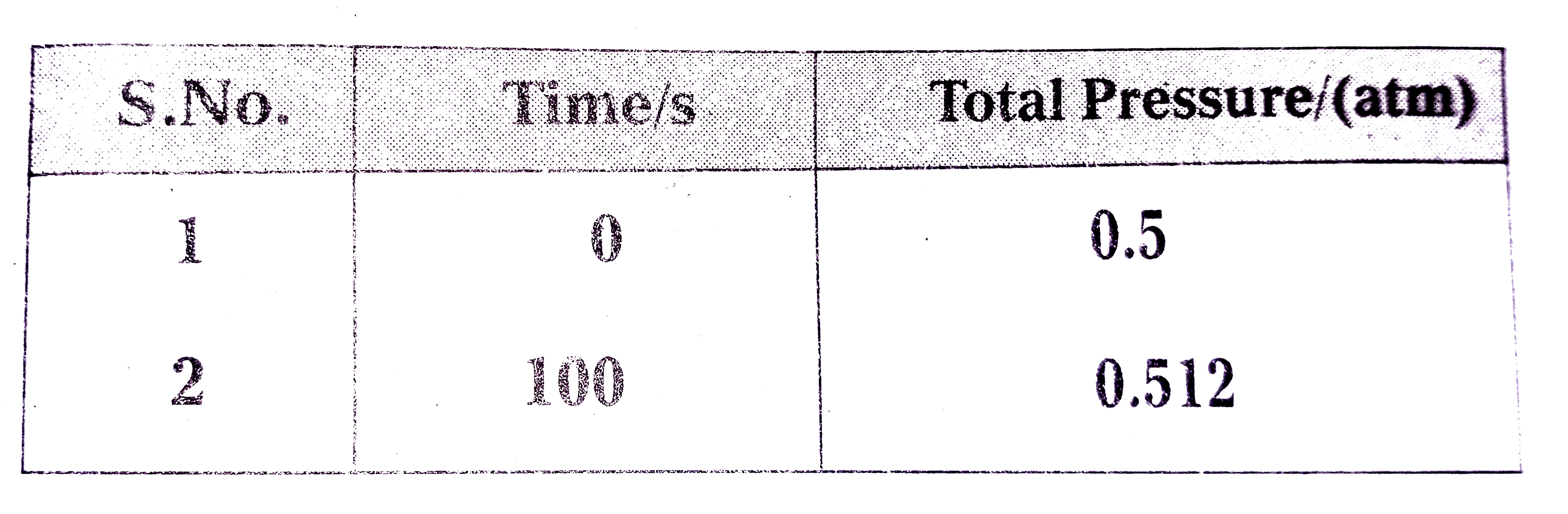
Calculate the rate constant.
View SolutionThe following data were obtained during the first order thermal decomposition of SO2Cl2 at a constant volume :
SO2Cl2(g)→SO2(g)+Cl2(g)

Calculate the rate constant
( given : log 4=0.6021,log2=0.3010)
View SolutionThe following data were obtained during the first order thermal decomposition of SO2Cl2 at a constant volume :
SO2Cl2(g)→SO2(g)+Cl2(g)

Calculate the rate constant
( given : log 4=0.6021,log2=0.3010)
View SolutionThe following data were obtained during the first order thermal decomposition of SO2Cl2 at a constant volume :
SO2Cl2(g)→SO2(g)+Cl2(g)

Calculate the rate constant
( given : log 4=0.6021,log2=0.3010)
View SolutionFor the hydrolysis of methyl acetate in aqueous solution, the following results were obtained :
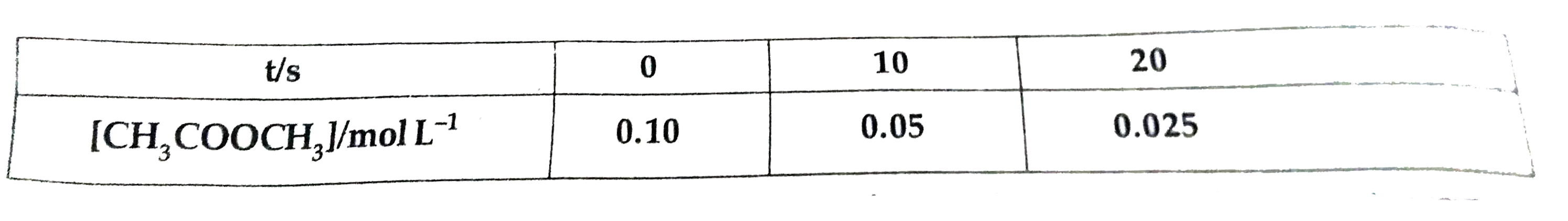
(a) Show that it follows pseudo first order reaction, as the concentration of water remains constant.
(b) Calculate the average rate of reaction between the time interval 10 to 20 seconds. (Given : log 2 = 0.3010, log 4 = 0.6021)
View Solution
