If the radius of the octaheral void is $r$ and the radius of the atoms in close-packing is $R$ , derive relation between $r$ and $R$

Video Solution

Text Solution

Verified by Experts

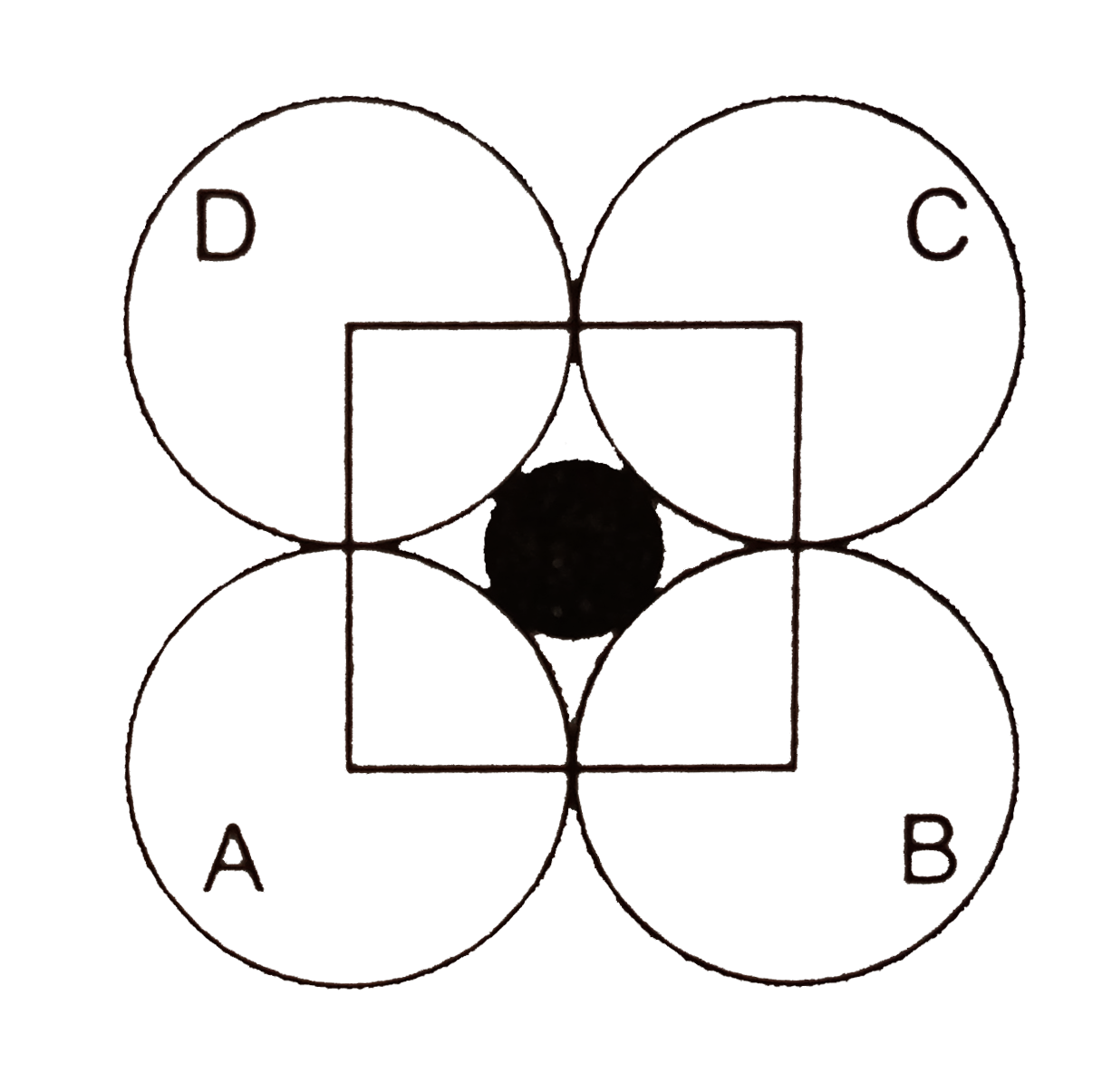
Consider an octahedral void represented by the shaded portion $($ the shown $)$ . Now suppose the length of the each arm of the square will be $2 R (R$ is radius of atom $)$ . Let $r$ be the radius of void. For right angle triangle $A B C$ .
$A C = \sqrt{[{(A B)}^{2} + {(B C)}^{2}]}$
$\sqrt{[{(2 R)}^{2} + {(2 R)}^{2}]} = \sqrt{8 R}$
Also, $A C = R + R + 2 r = 2 r + 2 R = 2 (r + R)$
or $\sqrt{8 R} = 2 \sqrt{2 R} = 2 (r + R)$
or $r = \sqrt{2 R} - R$
$r = [\sqrt{2} - 1] R = 0.414 \times R$

Updated on:21/07/2023

If $r$ is the radius of the octahedral voids and $R$ is the radius of the atom in close packing,then $:$
A $\frac{R}{r} = 9.1$
B $\frac{R}{r} = 3.22$
C $\frac{R}{r} = 2.41$
D $\frac{R}{r} = 4.67$
If the radius of the octahedral void is $r$ and radius of the atom in closet packed structure is $R$ then
A $r / R = 0.414$
B $r / R = 0.225$
C $r / R = 0.155$
D $r / R = 0.732$
If $R$ is the radius of the octahedral voids and $r$ is the radius of the atom in close packing, then $r / R$ is equal to
A $2.41$
B $4.76$
C $3.22$
D $9.1$