Radiactive decay is a reaction of
Zero order
First order
Second order
Third order
The correct Answer is:B
To determine the order of radioactive decay, we can follow these steps:
Step 1: Understand the concept of radioactive decay
Radioactive decay is a process by which unstable atomic nuclei lose energy by emitting radiation. This process results in the transformation of the original nucleus into a different nucleus or a different energy state.
Hint: Remember that radioactive decay involves the transformation of nuclei and the emission of radiation.
Step 2: Analyze the rate of decay
The rate of radioactive decay is defined as the change in the number of radioactive nuclei over time. Mathematically, this can be expressed as:
dNdt∝−N
where N is the number of radioactive nuclei present at time t.
Hint: Look for how the rate of decay relates to the number of nuclei present.
Step 3: Introduce the decay constant
To remove the proportionality, we introduce a constant, known as the decay constant (λ):
dNdt=−λN
This equation indicates that the rate of decay is directly proportional to the number of nuclei present.
Hint: The decay constant is a key factor in determining the behavior of the decay process.
Step 4: Identify the order of the reaction
The equation dNdt=−λN is characteristic of a first-order reaction. In first-order reactions, the rate of reaction depends linearly on the concentration (or number of nuclei, in this case) of the reactant.
Hint: Recall the general form of first-order reactions and how they relate to the concentration of reactants.
Conclusion
Based on the analysis above, we conclude that radioactive decay is a first-order reaction.
Final Answer: Radioactive decay is a first-order reaction.
Radioactive decay is a process by which unstable atomic nuclei lose energy by emitting radiation. This process results in the transformation of the original nucleus into a different nucleus or a different energy state.
The rate of radioactive decay is defined as the change in the number of radioactive nuclei over time. Mathematically, this can be expressed as:
dNdt∝−N
where N is the number of radioactive nuclei present at time t.
To remove the proportionality, we introduce a constant, known as the decay constant (λ):
dNdt=−λN
This equation indicates that the rate of decay is directly proportional to the number of nuclei present.
The equation dNdt=−λN is characteristic of a first-order reaction. In first-order reactions, the rate of reaction depends linearly on the concentration (or number of nuclei, in this case) of the reactant.
Based on the analysis above, we conclude that radioactive decay is a first-order reaction.
Topper's Solved these Questions
- NUCLEAR CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Ex6.4 Objective
7 Videos
- NUCLEAR CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Ex6.5 Objective
10 Videos
- NUCLEAR CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Ex6.2 Objective
8 Videos
- NCERT BASED EXERCISEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NCERT BASED EXERCISEEXERCISE - Nuclear Chemistry (NCERT Exercise)
29 Videos
- ORGANIC COMPOUNDS WITH FUNCTIONAL GROUPBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ORGANIC COMPOUNDS WITH FUNCTIONAL GROUPEXERCISE - Archives Analytical And Descriptive
24 Videos
Similar Questions
Radioactive decay is a first− order process. Radioactive carbon in wood sample decays with a half− life of 5770 years. What is the rate constant ( in years ) for the decay ? What fraction would remains after 11540 years ?
View SolutionThe decay constant (λ) for radioactive decay is independent of
View Solution
Knowledge Check
A fraction f1 of a radioactive sample decays in one mean life, and a fraction f2 decays in one half life. Then
Af1>f2Bf1<f2Cf1=f2Deither of (a),(b ) or (c ) depending on the values of the mean life and half life.
An elementX decays , first by positron emission and then two α-particles are emitted in successive radiactive decay. If the product nucleus has a mass number 229 and atomic number 89, the mass number and atomic number of element X are.
View SolutionA radioactive sample decays to half of its initial concentration in 6.93 minutes. If it further decays another half in next 6.93 minutes, then the rate constant for the reaction is:
View SolutionIn a radioactive decay, neither the atomic number nor the mass number changes. Which of the following particles is emitted in the decay?
View SolutionHow are radioactive decay series distinguished ? Name the decay series which is not natural but artifical.
View SolutionConsider radioactive decay of A to B with which further decays either to X or Y, λ1,λ2 and λ3 are decay constant for A to B decay, B to X decay and Bto Y decay respectively. At t=0, the number of nuclei of A,B,X and Y are N0,N0 zero and zero respectively.
N1,N2,N3 and N4 are the number of nuclei of A,B,X and Y at any instant t.
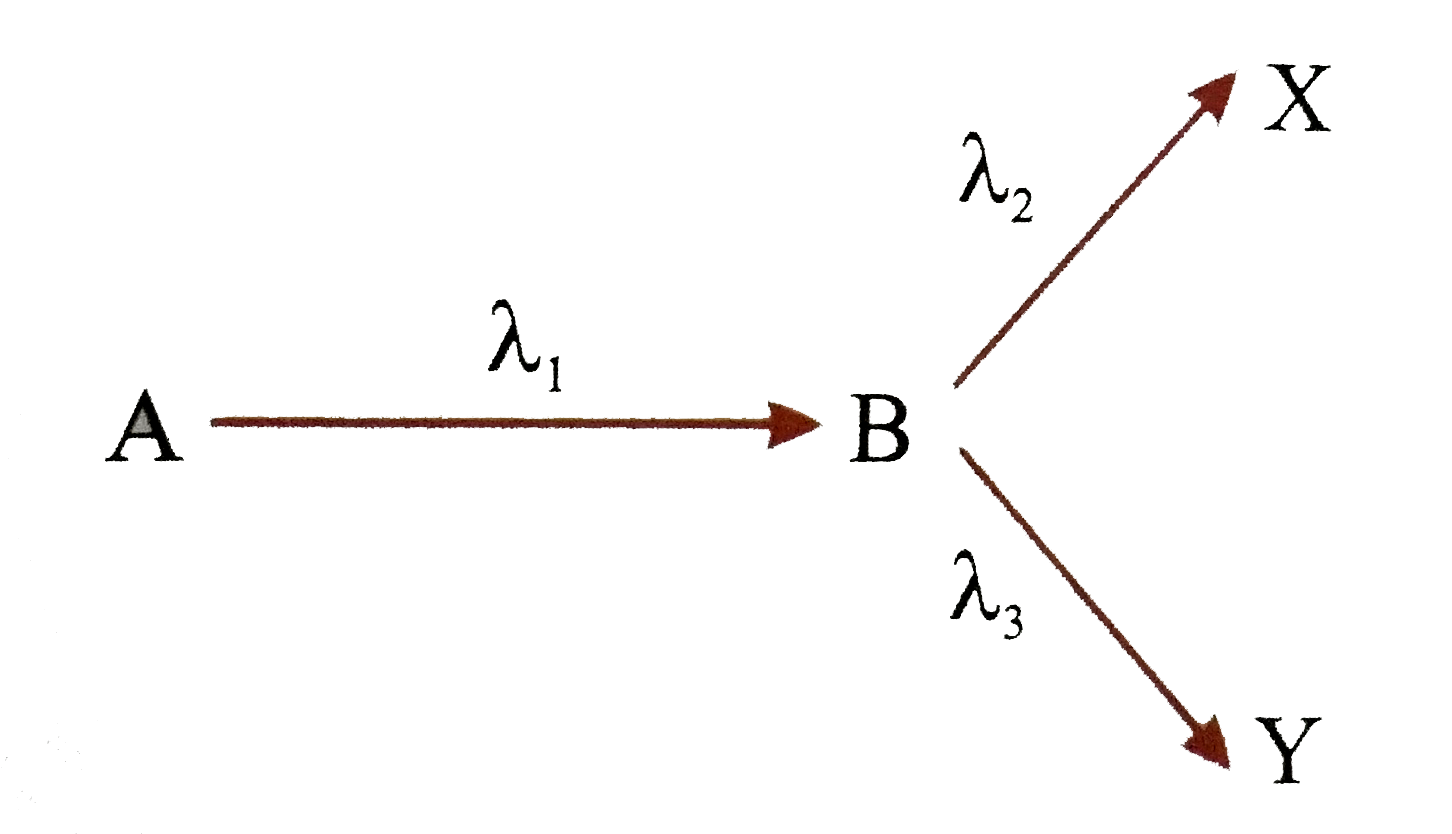
The net rate of accumulation of B at any instant is
View SolutionThe radioactive decay follows zero− order kinetics.
View SolutionSpores are liberated only after decay and decomposition of thallus in
View Solution
