The distribution of the molecular velocities of gas molecules at any temperature T is shown below. (The plot below is known as Maxwell's distribution of molecular speeds.)
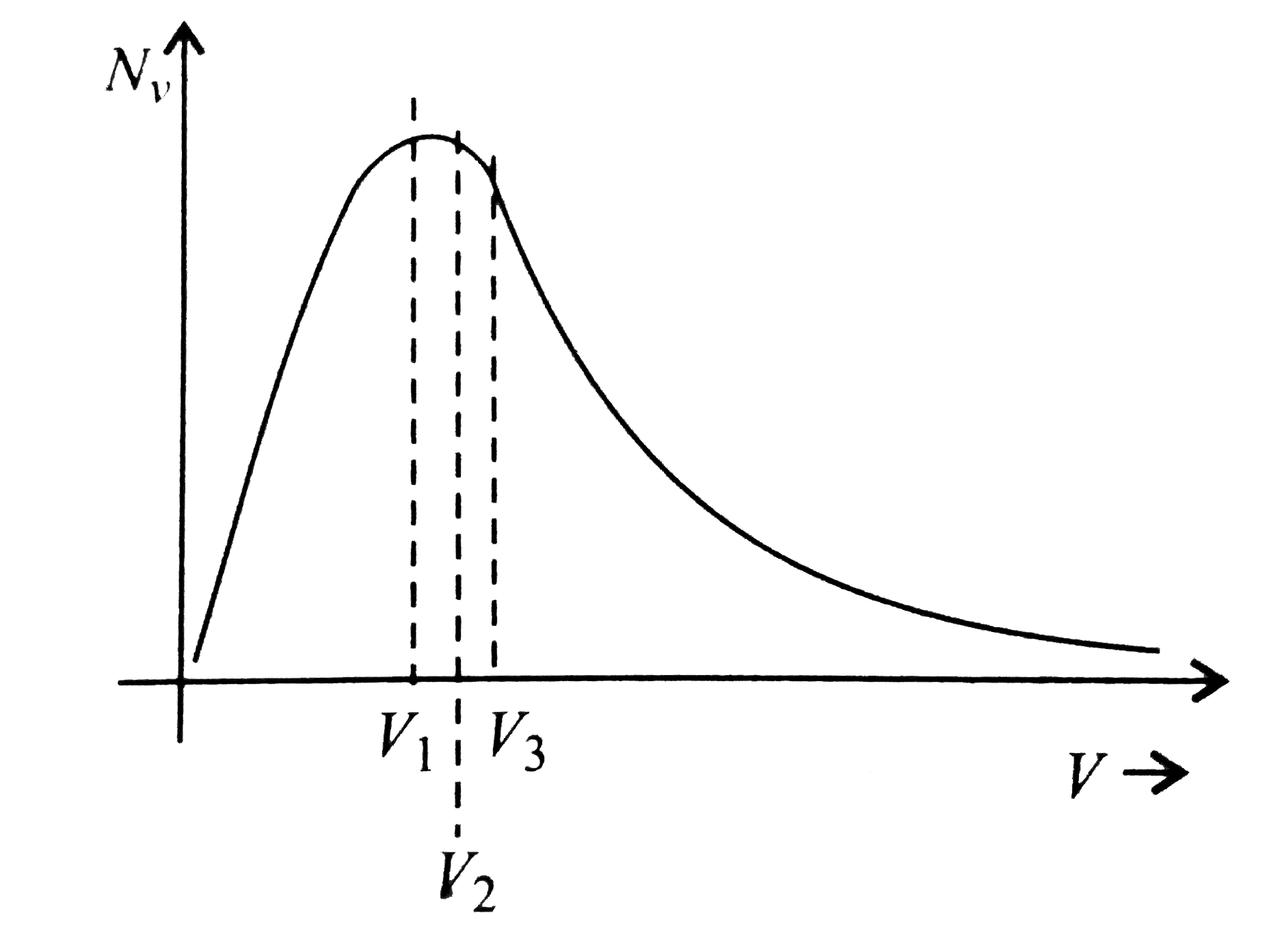
where
v is molecular velocity
n is number of molecules having velocity v
Let us define ΔNv, which is equal to the number of molecules between the velocity range v and v+Δv, given by
ΔNv=4πNa3e−bv2v2Δv
where
N is total number of molecules
a=√M02πRT and b=M02RT
R is universal gas constant
T is temperature of the gas
M0 is molecular weight of the gas
Answer the following question:
SI units of b are
A
m−2s−2
B
m2s2
C
m2s−2
D
ms−1
Text Solution
m−2s−2
M0:kgmol−1, R:kgm2s−2molK
|
Topper's Solved these Questions
- STATES OF MATTERBOOK - CENGAGE CHEMISTRYCHAPTER - STATES OF MATTEREXERCISE - Exercises (Multiple Correcttype)
32 Videos
- STATES OF MATTERBOOK - CENGAGE CHEMISTRYCHAPTER - STATES OF MATTEREXERCISE - Exercises (Single Correct)
85 Videos
- STATES OF MATTERBOOK - CENGAGE CHEMISTRYCHAPTER - STATES OF MATTEREXERCISE - Exercises
21 Videos
- SOME BASIC CONCEPTS AND MOLE CONCEPTBOOK - CENGAGE CHEMISTRYCHAPTER - SOME BASIC CONCEPTS AND MOLE CONCEPTEXERCISE - Archives Subjective
11 Videos
- STOICHIOMETRYBOOK - CENGAGE CHEMISTRYCHAPTER - STOICHIOMETRYEXERCISE - Archives Subjective
33 Videos
Similar Questions
Knowledge Check
The distribution of the molecular velocities of gas molecules at any temperature T is shown below. (The plot below is known as Maxwell's distribution of molecular speeds.)

where
v is molecular velocity
n is number of molecules having velocity v
Let us define ΔNv, which is equal to the number of molecules between the velocity range v and v+Δv, given by
ΔNv=4πNa3e−bv2v2Δv
where
N is total number of molecules
a=√M02πRT and b=M02RT
R is universal gas constant
T is temperature of the gas
M0 is molecular weight of the gas
Answer the following question:
If PPc=Pr, TTc=Tr, and VmVm,c=Vr whereAPr ia reduced pressure, Pc is critical pressureBTr ia reduced temperature, Tc is critical temperatureCVr is reduced volume, Vc is critical volumeDthen the temperature of state (or van der Waals equation), only in terms of Pr, Tr, and Vr isThe root mean square velocity of a gas molecule at any temperature T K of a gas molecule of molecular weight M is
A√8RT/MB√2RT/MC√3RT/MDzeroThe velocity of molecules of a gas at temperature 120 K is v. At what temperature will the velocity be 2v?
A120 KB240 KC480 KD1120 K
The velocity of the molecules of a gas at temperature 120 K is v. At what temperature will the velocity be 2 v ?
View SolutionA graph of Maxwell distribution of molecular velocities is plotted below.

Correct order of temperature
View SolutionIn maxwell's speed distribution curve, for N2 gas, the average of |relative velocity| between two molecules at 300 k will be : -
View SolutionPlot of Maxwell's distribution of velocities is given below:
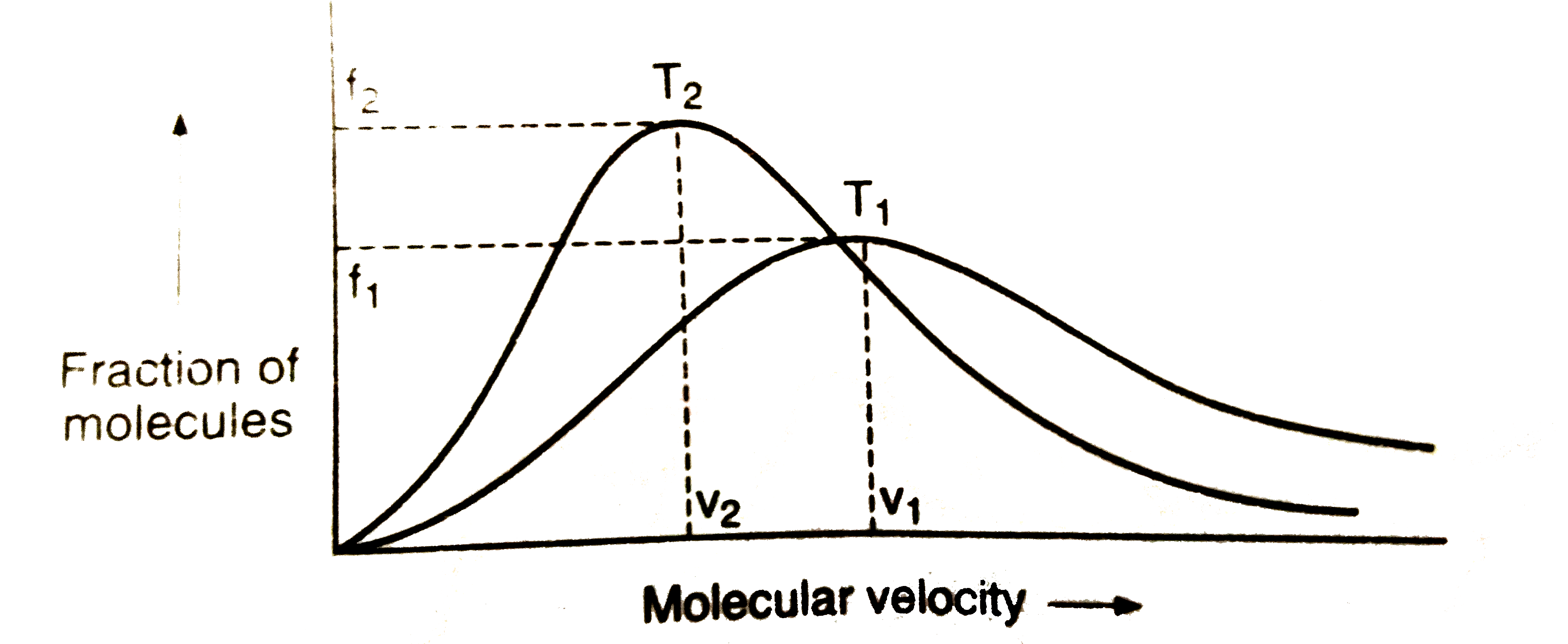
which of following is correct ?
View SolutionIn maxwell's speed distribution curve, for N2 gas, the average of |relative velocity| between two molecules at 300 k will be : -
View Solution
