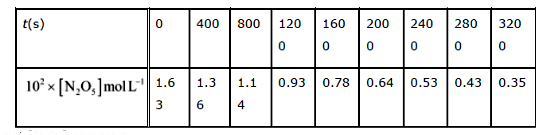
The experimental data for the decomposition of N2O5[2N2O5→4NO2+O2] in gas phase at 318 K are given below :
t/s0400800120016002000240028003200102×[N2O5]/molL−11⋅631⋅361⋅140⋅930⋅780⋅640⋅530⋅430⋅35
(i) Plot [N2O5] against time.(ii) Find the half-life period for the reaction.(iii) Draw a graph between log[N2O5] and t.(iv) What is rate law ?
(v) Calculate the rate constant. (vi) Calculate the half-life period from k and compare it with (ii).

Text Solution
Verified by Experts

t(s)0400800120016002000240028003200[N2O5](molL−1)1⋅631⋅361⋅140⋅930⋅780⋅640⋅530⋅430⋅35]×10−2log[N2O5]−1⋅79−1⋅87−1⋅94−2⋅03−2⋅11−2⋅19−2⋅28−2⋅37−2⋅46
(i) Plot of [N2O5] vs time
(ii) Initial conc. of N2O5=1⋅63×10−2 M,
Half of this concentration =0⋅815×10−2 M
Time corresponding to this concentration =1440 s. Hence, t1/2=1440 s.
(iii) Plot of log[N2O5] vs time
ocr_image
(iv) As plot of log[N2O5] vs time is a straight line, hence it is a reaction of first order, i.e., rate law is :
Rate =k[N2O5].
(v) Slope of the line =−k2⋅303
Slope =−2⋅46−(−1⋅79)3200−0=−0⋅673200,i.e.,k2⋅303=0⋅673200
or k=0⋅673200×2⋅303=4⋅82×10−4molL−1s−1
(vi) t1/2=0⋅693k=0⋅693482×10−4=1438s.

Doubtnut Promotions Banner
|
Updated on:21/07/2023
Class 12CHEMISTRYCHEMICAL KINETICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    2N2O5→4NO2+O2. The rate of reaction in terms of N2O5 will be

    A−d[N2O5]dt
    B−14d[N2O5]dt
    C−12d[N2O5]dt
    D−13d[N2O5]dt
  • Question 2 - Select One

    The first order rate constant for the decomposition of N2O5 is 6.2×10−4sec−1. The t1/2 of decomposition is

    A1117.2
    B111.7
    C223.4
    D160.9