Question

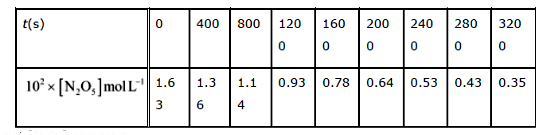
The experiment data for decomposition of $N_2{O}_5[2N_2{O}_5\to 4N{O}_2+O_2]$ in gas phase at 318 K are given below:

a) Plot $\left[N_2{O}_5\right]$ against t
b) Find the half life period for the reaction.
c) Draw a graph between log $\left[N_2{O}_5\right]$ and t
d) What is rate law?
e) Calculate the rate constant
f) Calculate the half life period from k and compare it with (b)

Explanation

The available data is:

a) The plot of `[N_(2)O_(5)]` versus time is as follows:

b) Initial conc. Of `N_(2)O_(5) = 1.63 xx 10^(-2)M`. Half of initial conc. `=1//2 xx (1.63 xx 10^(-2)M) = 0.815 xx 10^(-2)`M
Time corresponding to half of initial concentration `(t//2)` from the plot a) = 1400(s) approximately
c) The graph of log `[N_(2)O_(5)]` V.s time has been plotted.
d) Since the graph between log `[N_(2)O_(5)]` and time is a straight line the reaction is of first order.
The rate equation : rate (r) `= k[N_(2)O_(5)]`
e) Slope of the line `= ((-2.39) - (-1.88))/(2800 -400) =-(0.51)/(2400)`
But slope = `-k/2.303`, therefore = -(slope)`xx 2.303 = -(0.51/2400 xx 2.303)`
`=4.89 xx 10^(-4)s^(-1)`
f) Half of period `(t_(1//2))= 0.693/k = 0.693/k = 0.0693/(4.89 xx 10^(-4)s^(-1)) =1417s`
The half life period `t_(1//2)` as calculated from the slope is nearly the same as already predicted.