The experimental data for the decomposition of N2O5[2N2O5→4NO2+O2] in gas phase at 318 K are given below :
{t/s0400800120016002000240028003200102×[N2O5]/molL−11⋅631⋅361⋅140⋅930⋅780⋅640⋅530⋅430⋅35
(i) Plot "[N_(2)O_(5)]" against time.",,,,"
(ii) Find the half-life period for the reaction.")
("(iii) Draw a graph between "log[N_(2)O_(5)]" and t.",,,,"
(iv) What is rate law ?"
(v) Calculate the rate constant.
(vi) Calculate the half-life period from k and compare it with (ii).
To solve the problem step by step, we will follow the instructions given in the question and the video transcript. Let's break it down:
Step 1: Plot (N₂O₅) against time
1. Data Preparation: We have the following data:
- Time (s): 0, 400, 800, 1200, 1600, 2000, 2400, 2800, 3200
- Concentration of N₂O₅ (mol/L): 1.63, 1.36, 1.14, 0.93, 0.78, 0.64, 0.53, 0.43, 0.35
2. Graph Plotting: On a graph paper, plot the time on the x-axis and the concentration of N₂O₅ on the y-axis. The points will be plotted as follows:
- (0, 1.63)
- (400, 1.36)
- (800, 1.14)
- (1200, 0.93)
- (1600, 0.78)
- (2000, 0.64)
- (2400, 0.53)
- (2800, 0.43)
- (3200, 0.35)
3. Connecting the Points: Connect the points with a smooth curve. The curve should show a downward trend as the concentration of N₂O₅ decreases over time.
Step 2: Find the half-life period for the reaction
1. Identifying Half-Life: The half-life is the time at which the concentration of N₂O₅ is half of its initial value. The initial concentration is 1.63 mol/L, so half of this is 0.815 mol/L.
2. Determining Time: From the graph, find the time at which the concentration reaches approximately 0.815 mol/L. From the data, this occurs around 1600 seconds.
Step 3: Draw a graph between log(N₂O₅) and time
1. Calculate log(N₂O₅): Calculate the logarithm (base 10) of the concentrations:
- log(1.63) = 0.213
- log(1.36) = 0.134
- log(1.14) = 0.058
- log(0.93) = -0.031
- log(0.78) = -0.107
- log(0.64) = -0.193
- log(0.53) = -0.278
- log(0.43) = -0.367
- log(0.35) = -0.455
2. Plotting the Graph: Plot log(N₂O₅) on the y-axis and time on the x-axis. The points will be:
- (0, 0.213)
- (400, 0.134)
- (800, 0.058)
- (1200, -0.031)
- (1600, -0.107)
- (2000, -0.193)
- (2400, -0.278)
- (2800, -0.367)
- (3200, -0.455)
3. Connecting the Points: Draw a straight line through the points. This line should have a negative slope, indicating a first-order reaction.
Step 4: Determine the rate law
1. Rate Law Identification: Since the plot of log(N₂O₅) vs. time gives a straight line, this indicates that the reaction follows first-order kinetics. Therefore, the rate law can be expressed as:
Rate=k(N₂O₅)
Step 5: Calculate the rate constant (k)
1. Using the Slope: The slope of the line from the log(N₂O₅) vs. time graph is equal to −k/2.303.
2. Calculating the Slope: If we assume the slope is approximately −0.67 (as per the video), we can rearrange the equation:
k=−slope×2.303
k=0.67×2.303≈0.00146mol L−1s−1
Step 6: Calculate the half-life period from k
1. Half-Life Formula: For a first-order reaction, the half-life (t₁/₂) is given by:
t1/2=0.693k
2. Substituting k: Using the calculated value of k:
t1/2=0.6930.00146≈474.0s
3. Comparison: Compare this calculated half-life with the one obtained from the graph (1600 seconds). The values may differ due to experimental errors or approximations in plotting.
1. Data Preparation: We have the following data:
- Time (s): 0, 400, 800, 1200, 1600, 2000, 2400, 2800, 3200
- Concentration of N₂O₅ (mol/L): 1.63, 1.36, 1.14, 0.93, 0.78, 0.64, 0.53, 0.43, 0.35
- (0, 1.63)
- (400, 1.36)
- (800, 1.14)
- (1200, 0.93)
- (1600, 0.78)
- (2000, 0.64)
- (2400, 0.53)
- (2800, 0.43)
- (3200, 0.35)
1. Identifying Half-Life: The half-life is the time at which the concentration of N₂O₅ is half of its initial value. The initial concentration is 1.63 mol/L, so half of this is 0.815 mol/L.
1. Calculate log(N₂O₅): Calculate the logarithm (base 10) of the concentrations:
- log(1.63) = 0.213
- log(1.36) = 0.134
- log(1.14) = 0.058
- log(0.93) = -0.031
- log(0.78) = -0.107
- log(0.64) = -0.193
- log(0.53) = -0.278
- log(0.43) = -0.367
- log(0.35) = -0.455
- (0, 0.213)
- (400, 0.134)
- (800, 0.058)
- (1200, -0.031)
- (1600, -0.107)
- (2000, -0.193)
- (2400, -0.278)
- (2800, -0.367)
- (3200, -0.455)
1. Rate Law Identification: Since the plot of log(N₂O₅) vs. time gives a straight line, this indicates that the reaction follows first-order kinetics. Therefore, the rate law can be expressed as:
Rate=k(N₂O₅)
1. Using the Slope: The slope of the line from the log(N₂O₅) vs. time graph is equal to −k/2.303.
2. Calculating the Slope: If we assume the slope is approximately −0.67 (as per the video), we can rearrange the equation:
k=−slope×2.303
k=0.67×2.303≈0.00146mol L−1s−1
1. Half-Life Formula: For a first-order reaction, the half-life (t₁/₂) is given by:
t1/2=0.693k
2. Substituting k: Using the calculated value of k:
t1/2=0.6930.00146≈474.0s
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - PRADEEPCHAPTER - CHEMICAL KINETICSEXERCISE - NCERT EXEMPLAR PROBLEMS WITH ANSWERS, HINTS AND SOLUTTIONS (MULTIPLE CHOICE QUESTIONS-1)
20 Videos
- CHEMICAL KINETICSBOOK - PRADEEPCHAPTER - CHEMICAL KINETICSEXERCISE - NCERT EXEMPLAR PROBLEMS WITH ANSWERS, HINTS AND SOLUTTIONS (MULTIPLE CHOICE QUESTIONS-II)
12 Videos
- CHEMICAL KINETICSBOOK - PRADEEPCHAPTER - CHEMICAL KINETICSEXERCISE - NCERT QUESTIONS AND EXERCISES WITH ANSWERS (NCERT INTEXT UNSOLVED QUESTIONS & PROBLEMS)
9 Videos
- BIOMOLECULES BOOK - PRADEEPCHAPTER - BIOMOLECULES EXERCISE - IMPORTANT QUESTIONS (FOR BOARD EXAMINATION)
25 Videos
- CHEMISTRY IN EVERYDAY LIFE BOOK - PRADEEPCHAPTER - CHEMISTRY IN EVERYDAY LIFE EXERCISE - IMPORTANT QUESTION FOR BOARD EXAMINATION
30 Videos
Similar Questions
The experimental data for decomposition of N2O5
[2N2O5→4NO2+O2]
in gas phase at 318K are given below:
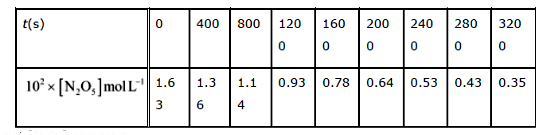
(i) Plot [N2O5] against t.
(ii) Find the half-life period for the reaction.
(iii) Draw a graph between log[N2O5] and t.
(iv) What is the rate law ?
(v) Calculate the rate constant.
(vi) Calculate the half-life period from k and compare it with (ii).
View SolutionThe experimental data for the decomposition of N2O5,[2N2O5→4NO2+O2] in gas phase at 318 K are given below:

(i) Plot [N2O5] against t.
(ii) Find the half-life period for the reaction.
(iii) Draw a graph between log[N2O5] and t.
(iv) What is the rate law?
(v) Calculate the rate constant.
(vi) Calculate the half-life period from k and compare it with (ii).
View Solution
Knowledge Check
For the reaction 2N2O5→4NO2+O2, the rate of the reaction can be given by
Ad[N2O5]dtB−12d[N2O5]dtC+14d[NO2]dtD−4d[NO2]dt2N2O5→4NO2+O2. The rate of reaction in terms of N2O5 will be
A−d[N2O5]dtB−14d[N2O5]dtC−12d[N2O5]dtD−13d[N2O5]dtThe first order rate constant for the decomposition of N2O5 is 6.2×10−4sec−1. The t1/2 of decomposition is
A1117.2B111.7C223.4D160.9
The experiment data for decomposition of N2O5[2N2O5→4NO2+O2] in gas phase at 318 K are given below:

a) Plot [N2O5] against t
b) Find the half life period for the reaction.
c) Draw a graph between log [N2O5] and t
d) What is rate law?
e) Calculate the rate constant
f) Calculate the half life period from k and compare it with (b)
View SolutionThe rate law for decomposition N2O5 is rate =k[N2O5]
What is the significance of k in the equation?
View SolutionThe rate law for the decomposition of N2O5 is given as: Rate =k[N2O5]. What is the significance of k in the equation?
View SolutionThe rate law for decomposition N2O5 is rate =k[N2O5]
What is the significance of k in the equation?
View SolutionAt 373 K, the half-life period for the thermal decomposition of N2O5 is 4.6 sec and is independent of the initial pressure of N2O5. Calculate the specific rate constant at this temperature.
View Solution
