The transition of electron in if atom that will emit maximum energy is
n3→n2
n4→n3
n2→n4
n6→n5
The correct Answer is:A
To determine the transition of an electron in an atom that will emit maximum energy, we can follow these steps:
Step 1: Understand the Energy Formula
The energy of an electron in an atom is given by the formula:
E=−13.6Z2n2
where E is the energy, Z is the atomic number, and n is the principal quantum number.
Step 2: Analyze the Relationship Between Energy and n
From the formula, we can see that energy E is inversely proportional to the square of the principal quantum number n:
E∝1n2
This means that as n increases, the energy E decreases.
Step 3: Determine the Condition for Maximum Energy
To achieve maximum energy, we need to minimize the value of n. The lowest value of n in a hydrogen-like atom is n=1.
Step 4: Identify the Possible Transitions
Given the options for transitions (for example, from n2 to n1, n3 to n2, etc.), we need to find the transition that involves the smallest n value.
Step 5: Evaluate the Transitions
- Transition from n2 to n1 (maximum energy emission)
- Transition from n3 to n2 (less energy)
- Transition from n4 to n3 (even less energy)
- Transition from n6 to n5 (least energy)
Among these transitions, the transition from n2 to n1 will emit the maximum energy because it involves the largest change in energy levels.
Conclusion
The transition of an electron in an atom that will emit maximum energy is the transition from n2 to n1.
---
The energy of an electron in an atom is given by the formula:
E=−13.6Z2n2
where E is the energy, Z is the atomic number, and n is the principal quantum number.
From the formula, we can see that energy E is inversely proportional to the square of the principal quantum number n:
E∝1n2
This means that as n increases, the energy E decreases.
To achieve maximum energy, we need to minimize the value of n. The lowest value of n in a hydrogen-like atom is n=1.
Given the options for transitions (for example, from n2 to n1, n3 to n2, etc.), we need to find the transition that involves the smallest n value.
- Transition from n2 to n1 (maximum energy emission)
- Transition from n3 to n2 (less energy)
- Transition from n4 to n3 (even less energy)
- Transition from n6 to n5 (least energy)
The transition of an electron in an atom that will emit maximum energy is the transition from n2 to n1.
Topper's Solved these Questions
- ATOMIC STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ATOMIC STRUCTUREEXERCISE - Exercises Assertion And Reason
21 Videos
- ATOMIC STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ATOMIC STRUCTUREEXERCISE - Exercises Integer
11 Videos
- ATOMIC STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ATOMIC STRUCTUREEXERCISE - Exercises Multiple Correct
45 Videos
- APPENDIX - INORGANIC VOLUME 1BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - APPENDIX - INORGANIC VOLUME 1EXERCISE - chapter-7 Single correct answer
1 Videos
- CHEMICAL BONDING AND MOLECULAR STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTUREEXERCISE - Archives Subjective
15 Videos
Similar Questions
Knowledge Check
The ground state energy of an atom is −13.6eV. The photon emitted during the transition of electron from n=3 to n=1 state, is incidenet on a photosensitive material of unknown work function. The photoelectrons are emitted from the materials with a maximum kinetic energy of 9 eV. the threshold wavelength of the material used is
A0.9×10−7mB4×10−7mC0.47×10−7mD9×10−7m
Which of the following transition will emit maximum energy in hydrogen atom ?
View SolutionIn Bohr Atomic model regarding transition of electron for hydrogen like atom following observation were noted.
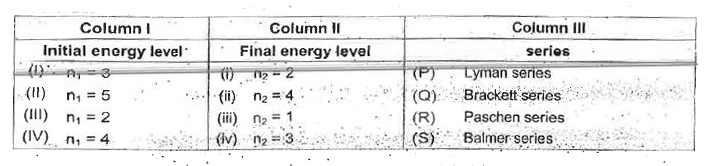
Transition of electron to corresponding to lowest energy released is
View SolutionWhat will be the kinetic energy and total energy of an electron in H atom if the atom emit a photon of wavelength 4860Å ?
View SolutionCalculate the ratio of the frequencies of the radiation emitted due to transition of the electron in a hydrogen atom from its (i) second permitted energy level to the first level and (ii) highest permitted energy level to the second permitted level .
View SolutionIf the wavelength of photon emitted due to transition of electron from third orbit to first orbit in a hydrogen atom is λ then the wavelength of photon emitted due to transition of electron from fourth orbit to second orbit will be
View SolutionWhich electronic transition in a hydrogen atom releases the greatest amount of energy?
View SolutionIf an electron transits from n = 7 to n = 1 in a hydrogen atom then the maximum number of spectral lines that can form will be?
View Solution
