X, Y and Z react in the 1:1:1 stoichiometric ratio.
The concentration of X, Y and Z we are found to vary with time as shown in the figure below:
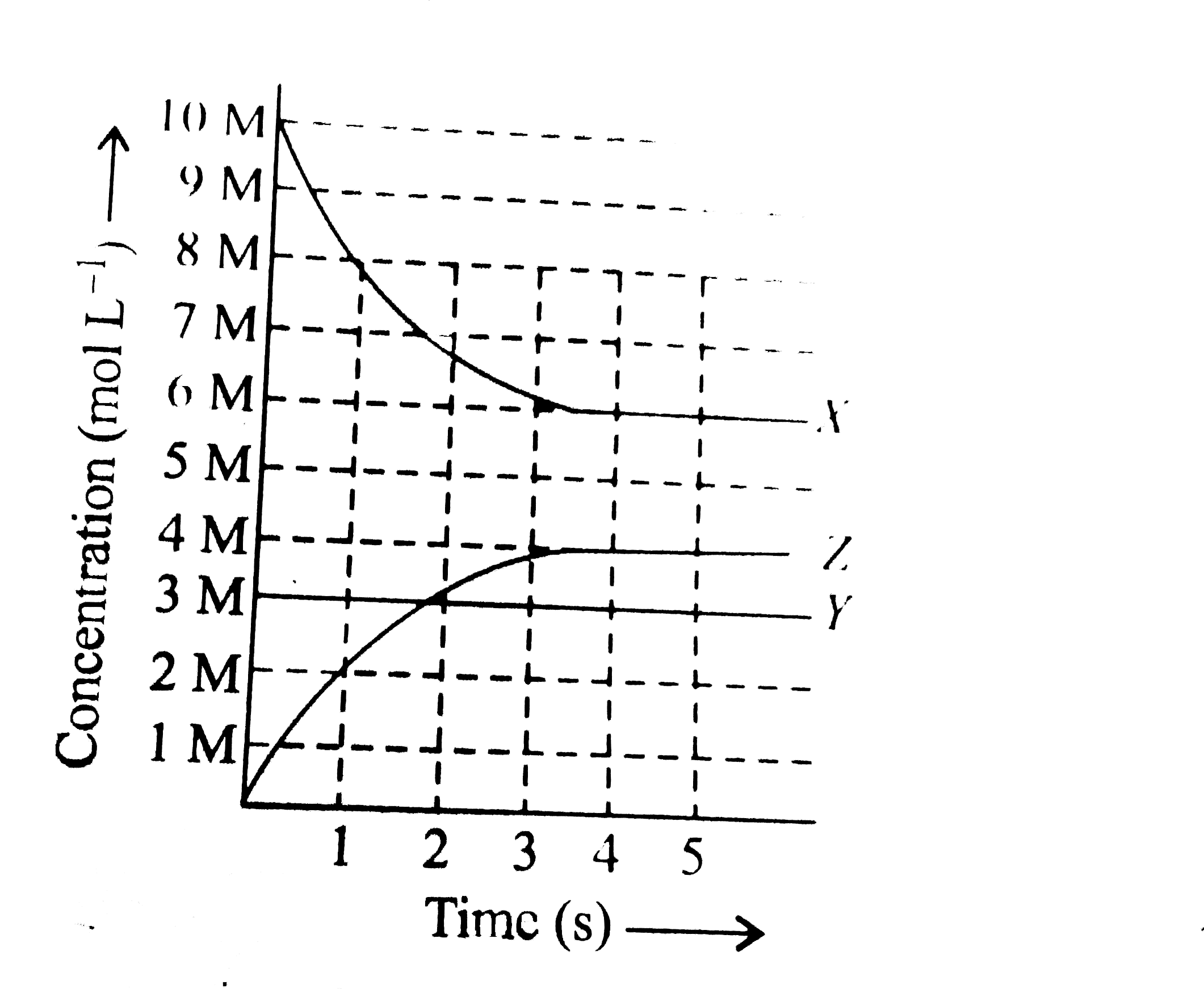
If the above equilibrium is established in a 2.0L container by taking reactants in sufficient amount then how many moles of components Y must have reacted to establish the equilibrium?
0
6
12
8
The correct Answer is:D
X(g)+Y(g)⇐⇒Z(g)moles leftatt=tEq6×2=124×2=8
To get 8 moles of Z, moles of Y reacted =8
Topper's Solved these Questions
- CHEMICAL EQUILIBRIUMBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Exercises (Multiple Correct)
26 Videos
- CHEMICAL EQUILIBRIUMBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Exercises (Single Correct)
58 Videos
- CHEMICAL EQUILIBRIUMBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Exercises (Subjective)
46 Videos
- CHEMICAL BONDING AND MOLECULAR STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTUREEXERCISE - Archives Subjective
15 Videos
- CLASSIFICATION AND NOMENCLATURE OF ORGANIC COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CLASSIFICATION AND NOMENCLATURE OF ORGANIC COMPOUNDSEXERCISE - Analytical and Descriptive Type
3 Videos
Similar Questions
X, Y and Z react in the 1:1:1 stoichiometric ratio.
The concentration of X, Y and Z we are found to vary with time as shown in the figure below:
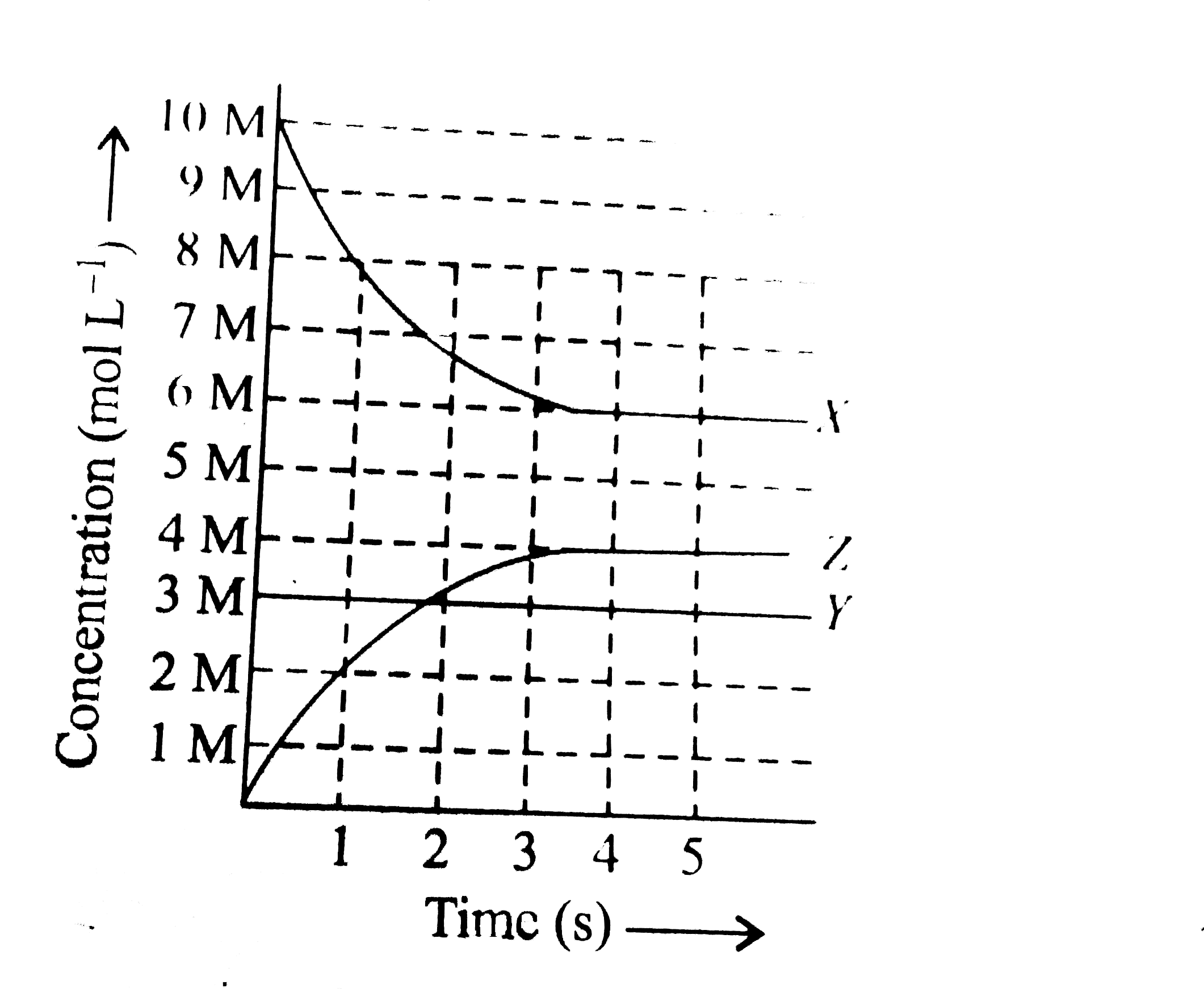
Which of the following equilibrium reaction represents the correct variation of concentration with time?
View SolutionX, Y and Z react in the 1:1:1 stoichiometric ratio.
The concentration of X, Y and Z we are found to vary with time as shown in the figure below:
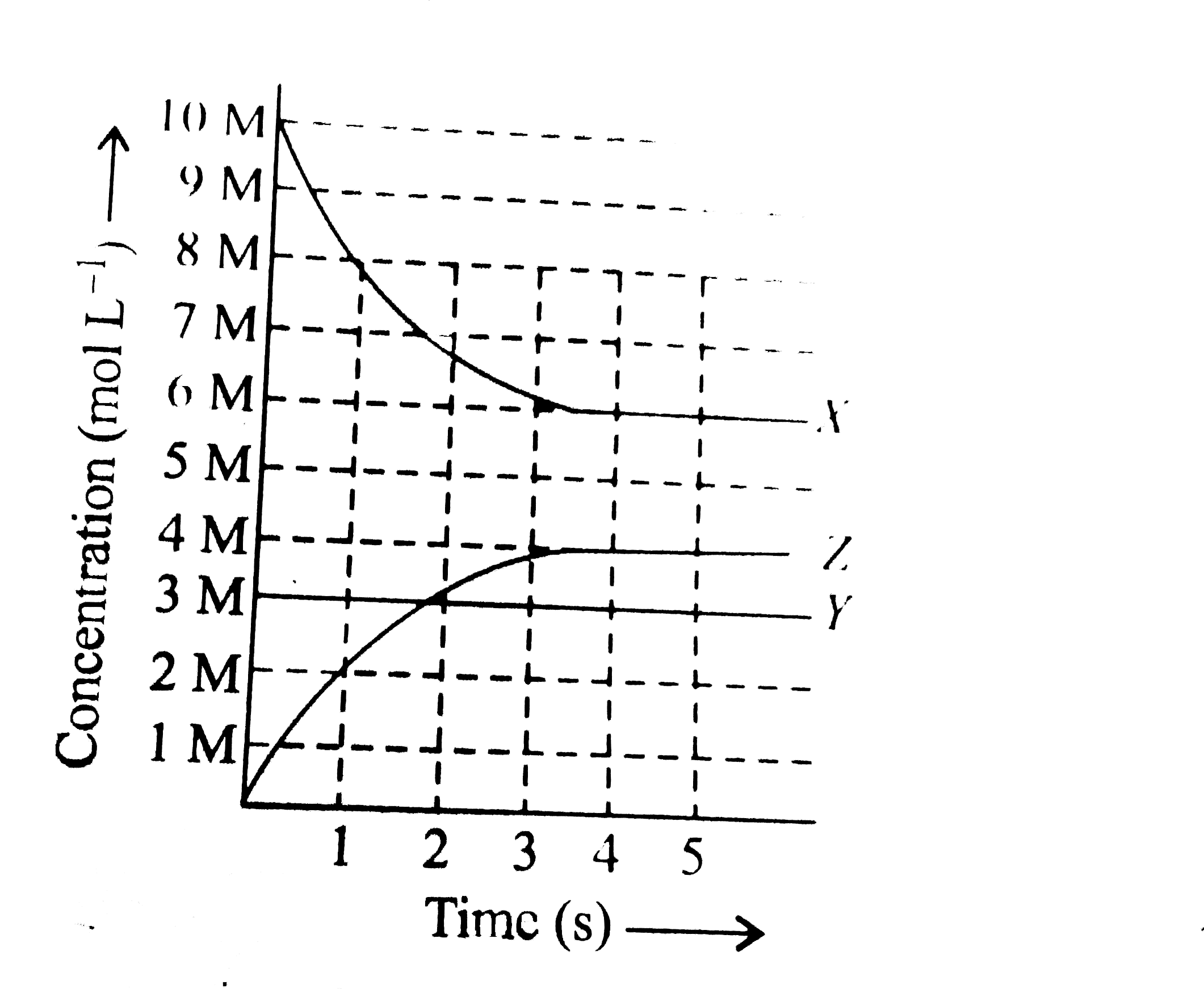
The value of the equilibrium constant (Kc) for the equilibrium represented the in above sketch will be
View SolutionThe equilibrium concentration of x, y and z are 4,2 and 2 mol L−1, respectively, at equilibrium of the reaction 2x+y⇔z. The value of Kc is ……………..
View SolutionThe initial concentration of X and Y were 2 and 4 mole / L respectively . For the following equilibrium X+2Y⇔Z which of the following relationship among equilibrium concentrations of x , y and z is not feasible ?
View SolutionOne mole of X and Y are allowed to react in a 2L container when equilinrium is reached the following reaction occurs 2X+Y⇔Z.
If the concentration of Z is 0.2M calculate the
equilibrium constant for this reaction
View SolutionFor a reversible reaction: X+2Y→2Z, the equilibrium concentrations of X,Y and Z are 0.32,0.40 and 0.35 moles L−1 respectively at 25∘C.
a. If unitially the system contained only X and Y and then reached the state of equilibrium, what were the initial concentrations of X and Y.
b. If at the start only X and Z were present, what were the initial concentrations?
View SolutionIn the reaction X(g)+Y(g)⇔2Z(g),2 mole of X,1 mole of Y and 1 mole of Z are placed in a 10 litre vessel and allowed to reach equilibrium .If final concentration of Z is 0.2 M , then Kcfor the given reaction is :
View SolutionIn the reaction X(g)+Y(g)⇔2Z(g), 2 moles of X, I mole of Y and I mole of Z are placed in a 10 lit vessel and allowed to reach equilibrium. If final concentration of Z is 0.2 M, then Kc. for the given reaction is
View SolutionFor the reaction, x+y↔2z initially 1 mole of x, 1.5 mole of y & 0.5 mole of z are taken, then at equilibrium 1 mole of z is obtained . If Keq=X/15 then , find the value of X.
View SolutionAt equilibrium X+Y⇔3Z,1 mol of X,2 mol of Y and 4 mol of Z are contained in a 3−L vessel. What will be the value of the reaction coefficient Q,
View Solution
