A 4m long ladder weighing 25 kg rests with its upper end against a smooth wall and lower end on rough ground. What should be the minimum coefficient of friction between the ground and the ladder for it to be inclined at 60∘ with the horizontal without slipping? (Take g=10ms−2)
In figure, AB is a ladder of weight w which acts at its centre of gravity G.
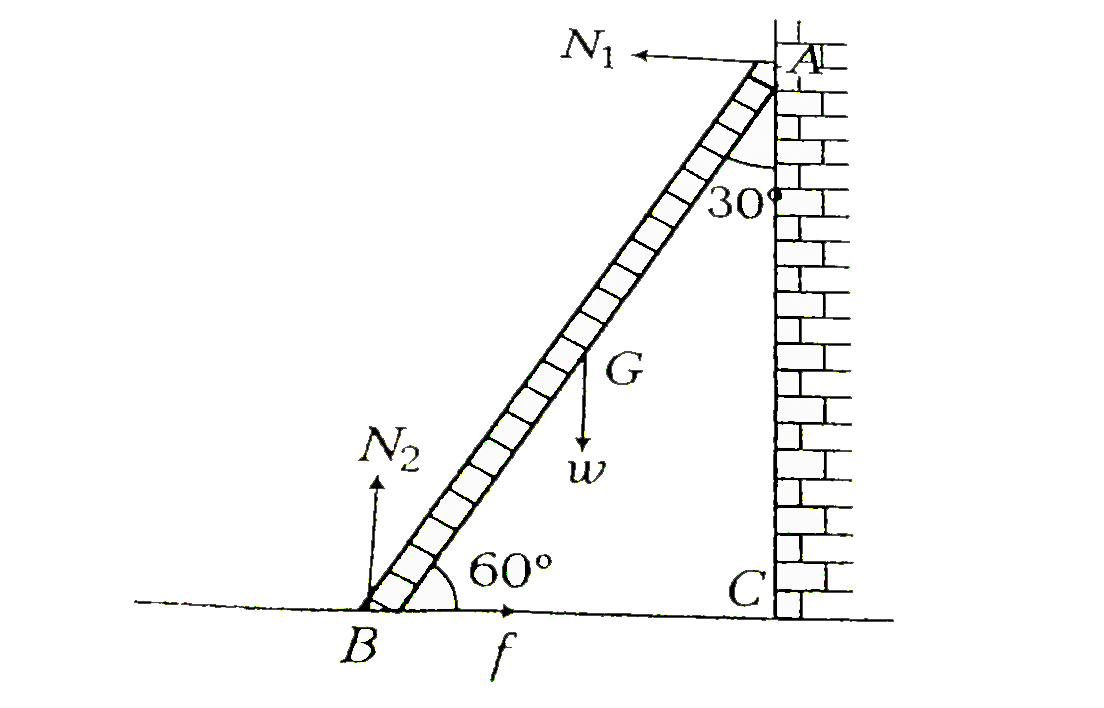
∠(ABC)=60∘∴∠(BAC)=30∘
Let N1 be the reaction of the wall, and N2 the reaction of the ground.
Force of friction f between the ladder and the ground acts along BC.
For horizontal equilibrium , f=N1.........(i)
For vertical equilibrium, N2=w ..........(ii)
Taking moments about B, we get for equilibrium,
N1(4cos30∘)−w(2cso60∘)=0.......(iii)
Here, w=250N
Solving these three equations , we get
f=72.17 Nand N2=250N
∴μ=fN2=72.17250=0.288
Similar Questions
A4m long ladder weighing 25kg rests with its upper and against a smooth wall and lower and on rought ground.What should be the minimum coefficient of friction between the ground and ladder for it to be inclined at 60∘ with the horizontal without slipping? (Takeg=10m/s2)
View SolutionA car goes on a circular horizontal road of radius 100 m. What should be the minimum coefficient of friction between the tyres and the road so that it completes the circle with velocity 10 m/s without slipping ? (Take g=10m/s2)
View SolutionA ladder of mass 10kg is held at reat against a smooth wall on a rough ground as shown in figure. The normal reaction between the wall and the ladder is
View SolutionA ladder of length l and mass m is placed against a smooth vertical wall, but the ground is not smooth. Coefficient of friction between the ground and the ladder is μ. The angle θ at which the ladder will stay in equilibrium is
View SolutionA ladder of length 5m is placed against a smooth wall as shown in figure. The coefficient or friction is μ between ladder and ground. What is the minimum value of μ , If the ladder is not to slip?

View SolutionA ladder is kept at rest with is upper end against a wall and the lower end on the ground. The ladder is more like to slip when a mass stands on it at the top than at the bottom. Why ?
View SolutionThe length of a uniform ladder of mass m is 1. It leans against a smooth wall making an angle θ with the horizontal. What should be the force of friction between the floor and ladder so that the ladder does not slip ?
View SolutionA ladder of length 5m is placed against a smooth wall as shown in figure. The coefficient or friction is μ between ladder and ground. What is the minimum value of μ , If the ladder is not to slip?

View SolutionA ladder of length 5m is placed against a smooth wall as shown in figure. The coefficient or friction is μ between ladder and ground. What is the minimum value of μ , If the ladder is not to slip?

View SolutionA uniform ladder which is 5m long has a mass of 25kg , leans with its upper end against a smooth vertical wall and its lower end on rough ground. The bottom of the ladder is 3m from the wall. Calculate the frictional force between the ladder and the ground . (g=10ms−2)
View Solution
