A ball falls freely form a height onto and smooth inclined plane forming an angle a with the horizontal. Find the ratio of the distance between the points at which the jumping ball strikes the inclined plane. Assume the impacts to be elastic.
The correct Answer is:1:2:3
To solve the problem of a ball falling freely from a height onto a smooth inclined plane, we need to analyze the motion of the ball and the impacts it makes with the inclined plane. Let's break down the solution step by step.
Step 1: Understanding the Motion of the Ball
The ball falls freely under the influence of gravity from a height h onto the inclined plane, which makes an angle α with the horizontal. The initial velocity of the ball just before it strikes the inclined plane can be determined using the equations of motion.
Hint: Remember that the initial velocity of the ball just before impact can be found using the kinematic equations.
Step 2: Resolving the Velocity Components
At the moment of impact, the velocity v0 of the ball can be resolved into two components:
- Perpendicular to the inclined plane: v0y=v0cosα
- Parallel to the inclined plane: v0x=v0sinα
Hint: Use trigonometric functions to resolve the velocity into components based on the angle of inclination.
Step 3: Analyzing the Impact
Since the impact is elastic, the component of velocity perpendicular to the inclined plane will reverse its direction, while the parallel component will remain unchanged. Therefore, after the impact:
- The new perpendicular velocity: v1y=−v0y=−v0cosα
- The new parallel velocity: v1x=v0x=v0sinα
Hint: Recall that in an elastic collision, the speed is conserved, but the direction of the perpendicular component changes.
Step 4: Time of Flight Calculation
To find the distance traveled by the ball after each impact, we need to calculate the time of flight until it strikes the inclined plane again. The time of flight can be calculated using the kinematic equations.
Using the equations of motion, we can find the time t for the ball to travel a distance d along the inclined plane after the first impact.
Hint: Use the equation d=v1xt+12axt2 to find the time of flight.
Step 5: Finding Distances After Each Impact
The distances r1,r2,r3 that the ball travels after each impact can be calculated using the respective time of flight and the velocity components.
1. For the first impact, the distance r1 can be calculated as:
r1=v0xt1+12axt21
2. For the second impact, the distance r2 can be calculated similarly, but using the new velocities after the first impact.
3. For the third impact, repeat the process.
Hint: Remember to substitute the values of v1x and ax correctly for each subsequent impact.
Step 6: Ratio of Distances
Finally, we need to find the ratio of the distances r1:r2:r3. After calculating the distances, we can express them in terms of a common factor and simplify to find the ratio.
Hint: Factor out common terms to simplify the ratio of the distances.
Conclusion
After performing the calculations, we find that the ratio of the distances at which the ball strikes the inclined plane is:
r1:r2:r3=1:2:3
This concludes the solution to the problem.
The ball falls freely under the influence of gravity from a height h onto the inclined plane, which makes an angle α with the horizontal. The initial velocity of the ball just before it strikes the inclined plane can be determined using the equations of motion.
At the moment of impact, the velocity v0 of the ball can be resolved into two components:
- Perpendicular to the inclined plane: v0y=v0cosα
- Parallel to the inclined plane: v0x=v0sinα
Since the impact is elastic, the component of velocity perpendicular to the inclined plane will reverse its direction, while the parallel component will remain unchanged. Therefore, after the impact:
- The new perpendicular velocity: v1y=−v0y=−v0cosα
- The new parallel velocity: v1x=v0x=v0sinα
To find the distance traveled by the ball after each impact, we need to calculate the time of flight until it strikes the inclined plane again. The time of flight can be calculated using the kinematic equations.
The distances r1,r2,r3 that the ball travels after each impact can be calculated using the respective time of flight and the velocity components.
r1=v0xt1+12axt21
2. For the second impact, the distance r2 can be calculated similarly, but using the new velocities after the first impact.
3. For the third impact, repeat the process.
Finally, we need to find the ratio of the distances r1:r2:r3. After calculating the distances, we can express them in terms of a common factor and simplify to find the ratio.
After performing the calculations, we find that the ratio of the distances at which the ball strikes the inclined plane is:
r1:r2:r3=1:2:3
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Single Correct
141 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Multiple Correct
25 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.3
24 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
A ball starts falling freely from a height h from a point on the inclined plane forming an angle α with the horizontal as shown. After collision with the incline it rebounds elastically off the plane. Then it again strikes the incline at
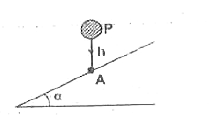
View SolutionA block is placed on a smooth inclined plane at an angle 'θ' to the horizontal. The acceleration must the plane be moved horizontally so that the block to fall freely is
View SolutionA trolley containing a liquid slides down a smooth inclined plane of angle α with the horizontal. Find the angle of inclination θ of the free surface with the horizontal.

View SolutionA uniform solid cyinder of radius R=15cm rolls over a horizontal plane passing into an inclined plane forming an ange α=30∘ with the horizontal. Find the maximum value of the velocity v0 which still permits the cylinder to roll on the inclined plane section without a jump. (The sliding is asumed to be absent).

View SolutionA ball is projected form a point A on a smooth inclined plane which makes an angle a to the horizontal. The velocity of projection makes an angle θ with the plane upwards. If on the second bounce the ball is moving perpendicular to the plane, find e in terms of α and θ. Here e is the coefficient of restitution between the ball and the plane.
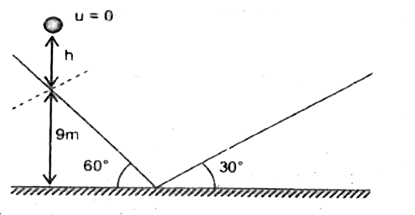
View SolutionA ball is dropped from a height h on a inclined plane, ball collides elastically with the first plane. Find the value of 'h' (in meter) so that it strikes perpendicular on the second inclined plane.
View SolutionA ball is projected horizontally with a speed v from the top of the plane inclined at an angle 45∘ with the horizontal. How far from the point of projection will the ball strikes the plane?
View SolutionA projectile si thrown with velocity of 50m/s towards an inclined plane from ground such that is strikes the inclined plane perpendiclularly. The angle of projection of the projectile is 53∘ with the horizontal and the inclined plane is inclined at an angle of 45∘ to the horizonta..

(a) Find the time of flight.
(b) Find the distance between the point of projection and the foot of inclined plane.
View SolutionA particle is projected with a velocity u making an angle θ with the horizontal. The instantaneous power of the gravitational force
View SolutionA particle is projected up an inclined plane of inclination β at an elevation ∝ to the horizontal. Find the ratio between tan∝andtanβ, if the particle strikes the plane horizontally.
View Solution
