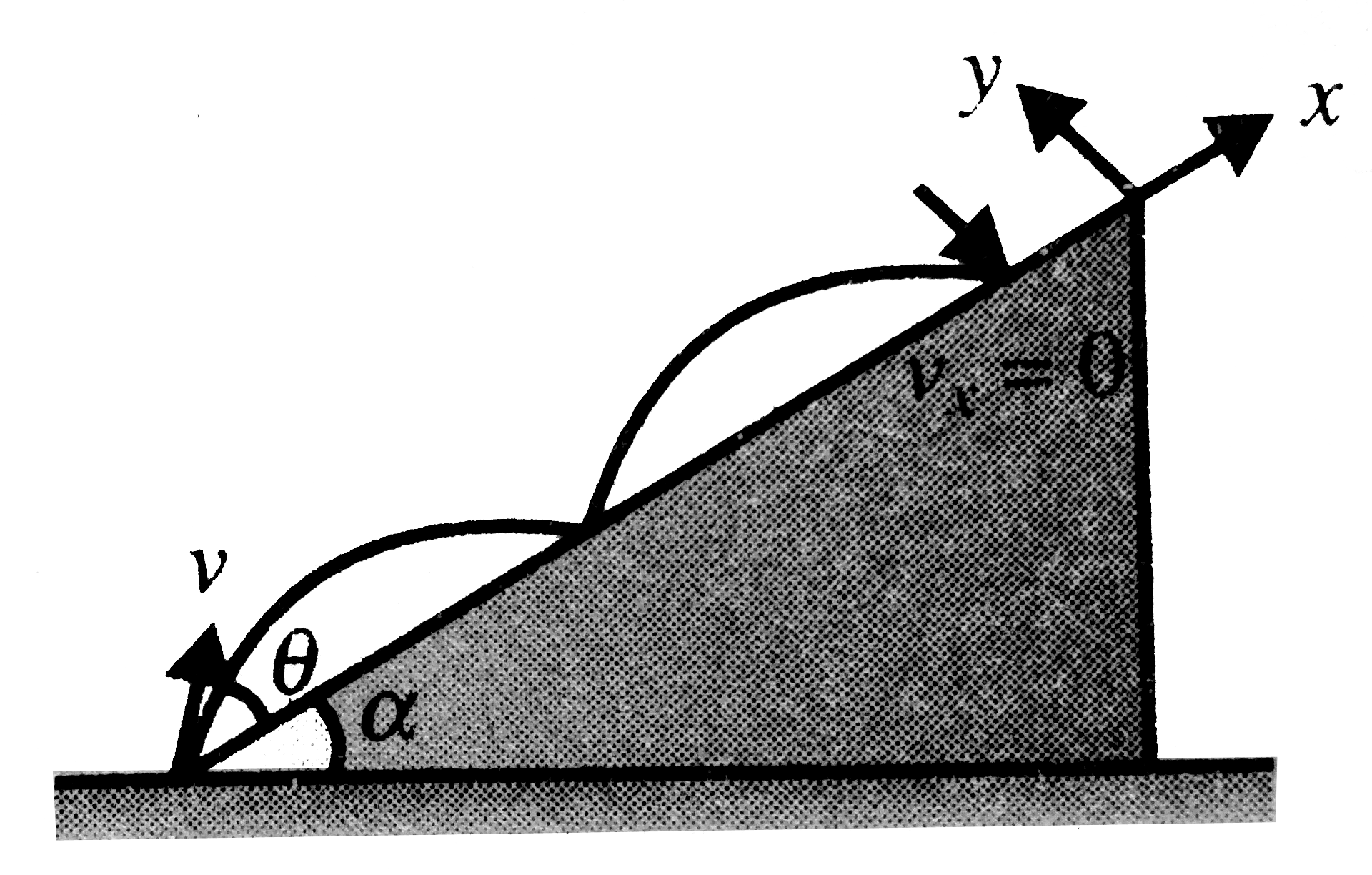
A ball is projected form a point A on a smooth inclined plane which makes an angle a to the horizontal. The velocity of projection makes an angle θ with the plane upwards. If on the second bounce the ball is moving perpendicular to the plane, find e in terms of α and θ. Here e is the coefficient of restitution between the ball and the plane.
The correct Answer is:θ−1
Let v be the velocity of projection,
a−x=−gsiα,a−y=−gcosα
Time of flight up the plane is T=2vsinθgcosα
Here vsinθ is component of velocity perpendicular to the plane which will becoem evsinθ after first imact e2vsinθ after second impact and so on. hence time of flight and so on. Hence time f flight will also be T,eT,e2T etc.
component of velocity along the plane becomes zero (vx=0) the time of second colision or after time T+et or (1+e)T. Hence applying
vx=ux+axt
0=vosθ−(gsinα)(1+e)T
or 0=vosθ−(gsinα)(1+e)2vsinθgcosα
or 1+e=cosθ2tanαsinθ
or 1+e=12cotαcotθ
or e=12cotαcotθ−1
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Single Correct
141 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Multiple Correct
25 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.3
24 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
A ball is projected from a point in a horizontal plane with a velocity of 20 m/s at 30∘ with the horizontal. Coefficient of restitution is 0.8, then
View SolutionA ball is projected from a point on a smooth horizontal floor at an angle θ with the horizontal . Speed of projection is u . Ball collides with the floor several times . Find the total time and horizontal distance covered by the ball .
View Solution
Knowledge Check
A ball is projected from a point in a horizontal plane with a velocity of 20 m/s at 30∘ with the horizontal. Coefficient of restitution is 0.8, then
Aduration for which ball will rebound is 20 sBduration for which ball will rebound is 10 sChorizontal component of velocity of the ball is 17.3 m/s.Dhorizontal distance before the ball ceases to rebound is 173 mA ball is projected horizontally with a speed v from the top of the plane inclined at an angle 45∘ with the horizontal. How far from the point of projection will the ball strikes the plane?
A2v2gB√2[2v2g]Cv2gD√2v2g
A ball is projected horizontally with a speed v from the top of the plane inclined at an angle 45∘ with the horizontal. How far from the point of projection will the ball strikes the plane?
View SolutionA ball falls freely form a height onto and smooth inclined plane forming an angle a with the horizontal. Find the ratio of the distance between the points at which the jumping ball strikes the inclined plane. Assume the impacts to be elastic.
View SolutionA ball falls freely form a height onto and smooth inclined plane forming an angle a with the horizontal. Find the ratio of the distance between the points at which the jumping ball strikes the inclined plane. Assume the impacts to be elastic.
View SolutionA ball is projected from the foot of an inclined plane of inclination 30∘ at an angle 60∘ with the horizontal,with velocity 20√3ms−1 .The time after which the ball will strike the plane is
View SolutionA particle is projected with a velocity u making an angle θ with the horizontal. The instantaneous power of the gravitational force
View SolutionA ball is projected horizontally with a speed u from the top of inclined plane of inclination α with horizontal. At what distance along the plane, the ball will strike the plane ?
View Solution
