A ball is prouected vertical up from the top of a cliff of height h with a speed v1 Another ball is projected vertically up with a speed v2 from the bottom of the cliff, after a time t0 from the instantof projection of the first ball, When will the balls meet?.
The correct Answer is:2(h+v2t0)+gt202{(v2−v1)+gt0}
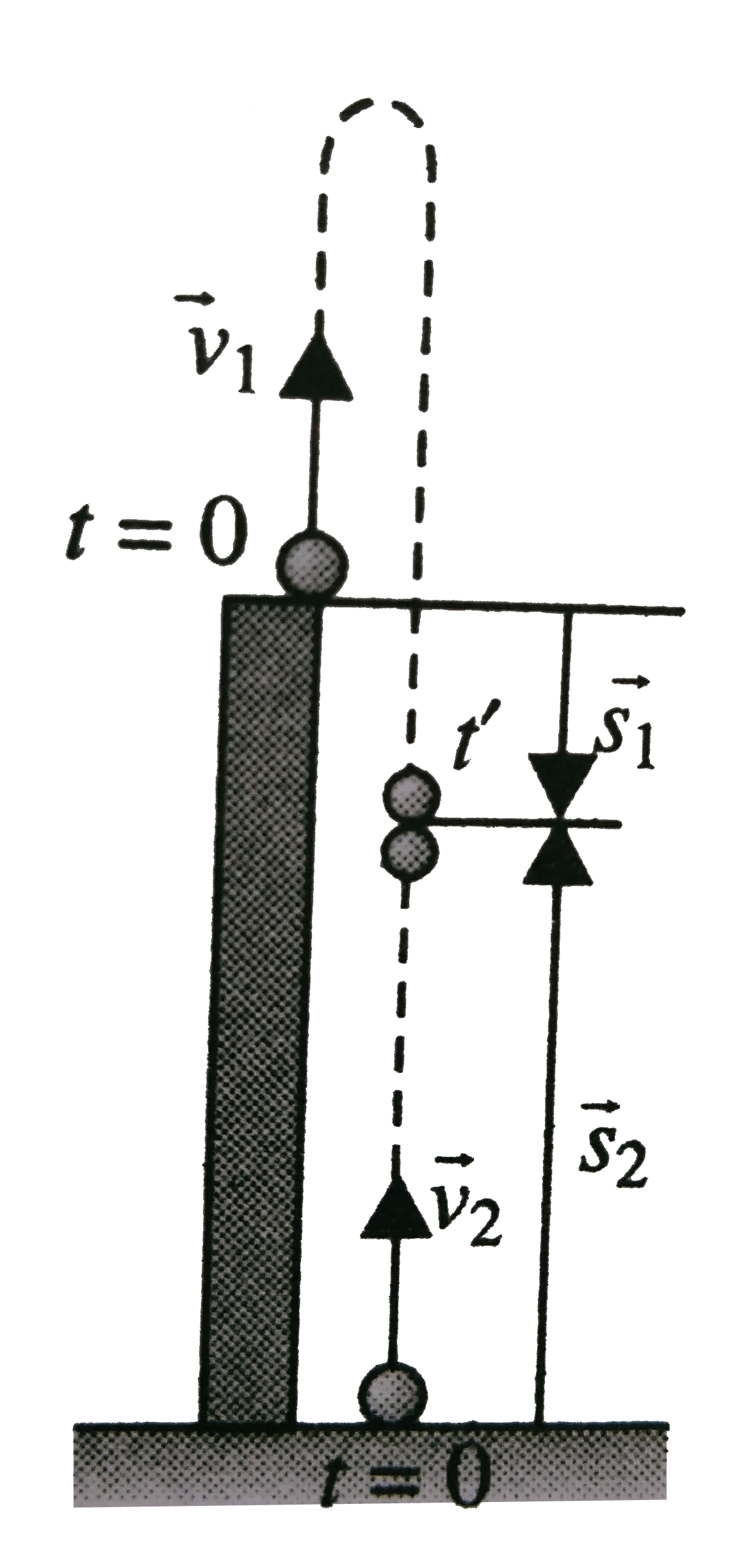
Let the balls meer after a time t' measured form the instant of projection of the first ball. Since, the second ball is projected aftre a time t0 from the instant of projection of the first ball, the second ball falls freely for a time t'−t0.
If the balls meet between the top and bottom of the cliff, for the first ball, s=−s1,v0=v1,A=−g and t=t'
This yields s1=−v1t'+12gt'2 (i)
For the second ball, S=S2,v0=v2,a=−g and t=(t'−t0)
Hence S2=v2(t'−t0)−12g(t'−t0)2 (ii)
Referring tothe fig we have s1+s2=h (iii)
Substituting s1 from eq, (i). s2 from eq. (ii) in eq, (iii), we have<br>v_(1)t'+(1)/(2)g t'^(2) +v_(2)(t'-t_(0))-(1)/(2)-(1)/(2)g(t'-t_(0))^(2)=h<br>Thisyields t'=(2(h+v_(2)t_(0))+g t_(0)^(2))/(2{(v_(2)-v_(1))+g t_(0)})
 .
.
Topper's Solved these Questions
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Single Correct
52 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Graphical Concept
17 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.4
16 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer
9 Videos
Similar Questions
A ball is projected downward from the top of a cliff of height h with an initial speed v.Another ball is simultaneously projected vertically up from the bottommost of the cliff with the same speed.When do they meet?
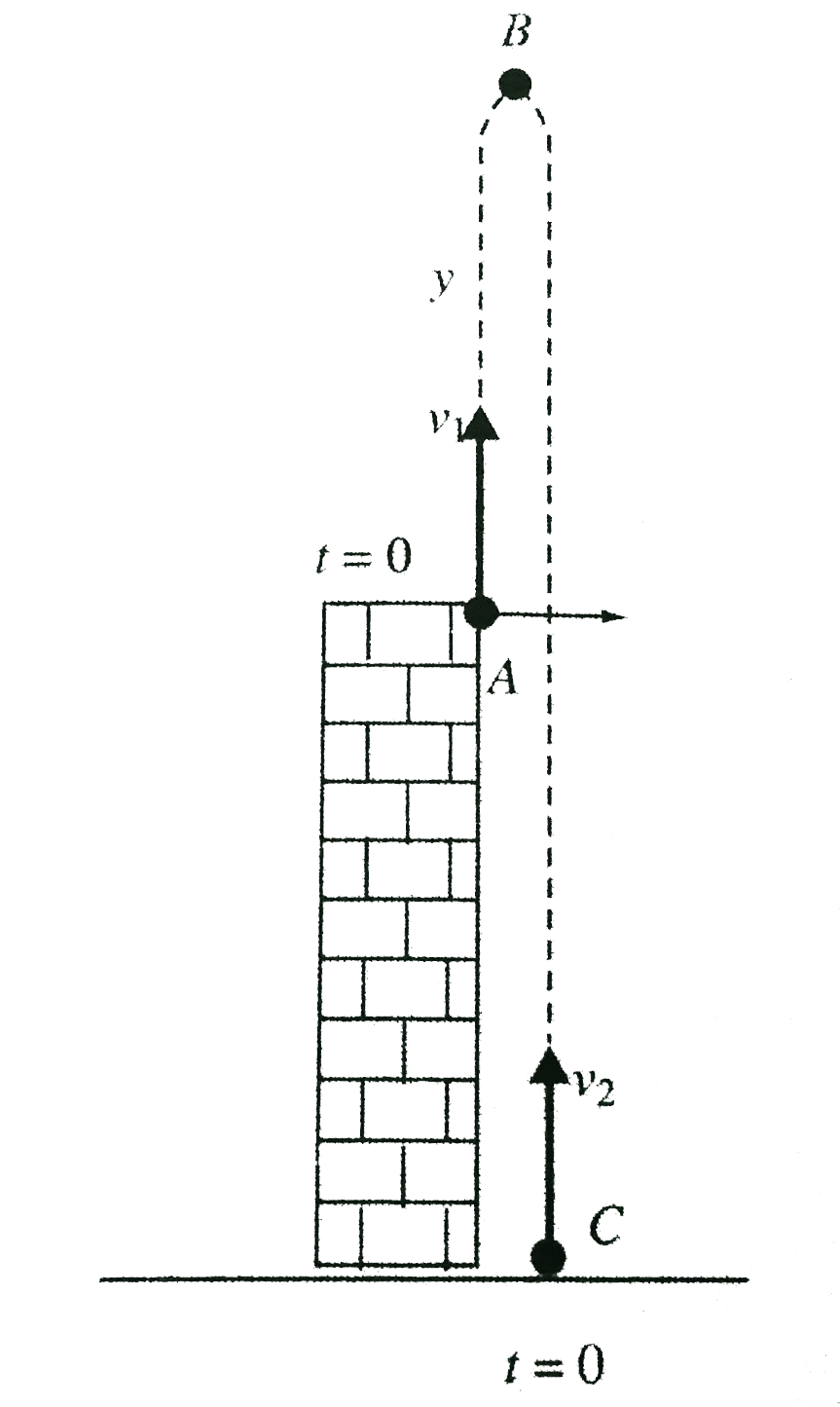
View SolutionTwo paarticles 1 and 2 are projected simultaneusly with velocities v1 and v2, respectively. Particle 1 is projectected vertically up from the top of a cliff of heitht h and particle 2 is projected vertically up from the bottom of the cliff. If the bodies meet (a) above the top of the cliff, (b) between the top and bottom of the the cliff, and (c ) below the bottom of the cliff, find the time of meeting of the particles.
 .
.
View Solution
Knowledge Check
A ball is projected vertically up with speed 20 m/s. Take g=10m/s2
AIt covers a distance of 5 m in 2nd second of its motionBThe displacement in 2nd and 3rd second are equalCThe distance covered in 2nd and 3rd second are equalDThe average speed for first 4 seconds is zeroA ball is thrown vertically upwards from the top of tower of height h with velocity v . The ball strikes the ground after time.
Avg[1+√1+2ghv2]Bvg[1+√1−2ghv2]Cvg(1+2ghv2)1/2Dvg(1−2ghv2)1/2A ball is projected horizontally with a speed v from the top of the plane inclined at an angle 45∘ with the horizontal. How far from the point of projection will the ball strikes the plane?
A2v2gB√2[2v2g]Cv2gD√2v2g
A body is dropped from a height h=50 m. Another body is projected vertically up with a speed V=10 m//sec after a time t0= 2 sec from the instant of release of the first body. Find the time of their meetings.
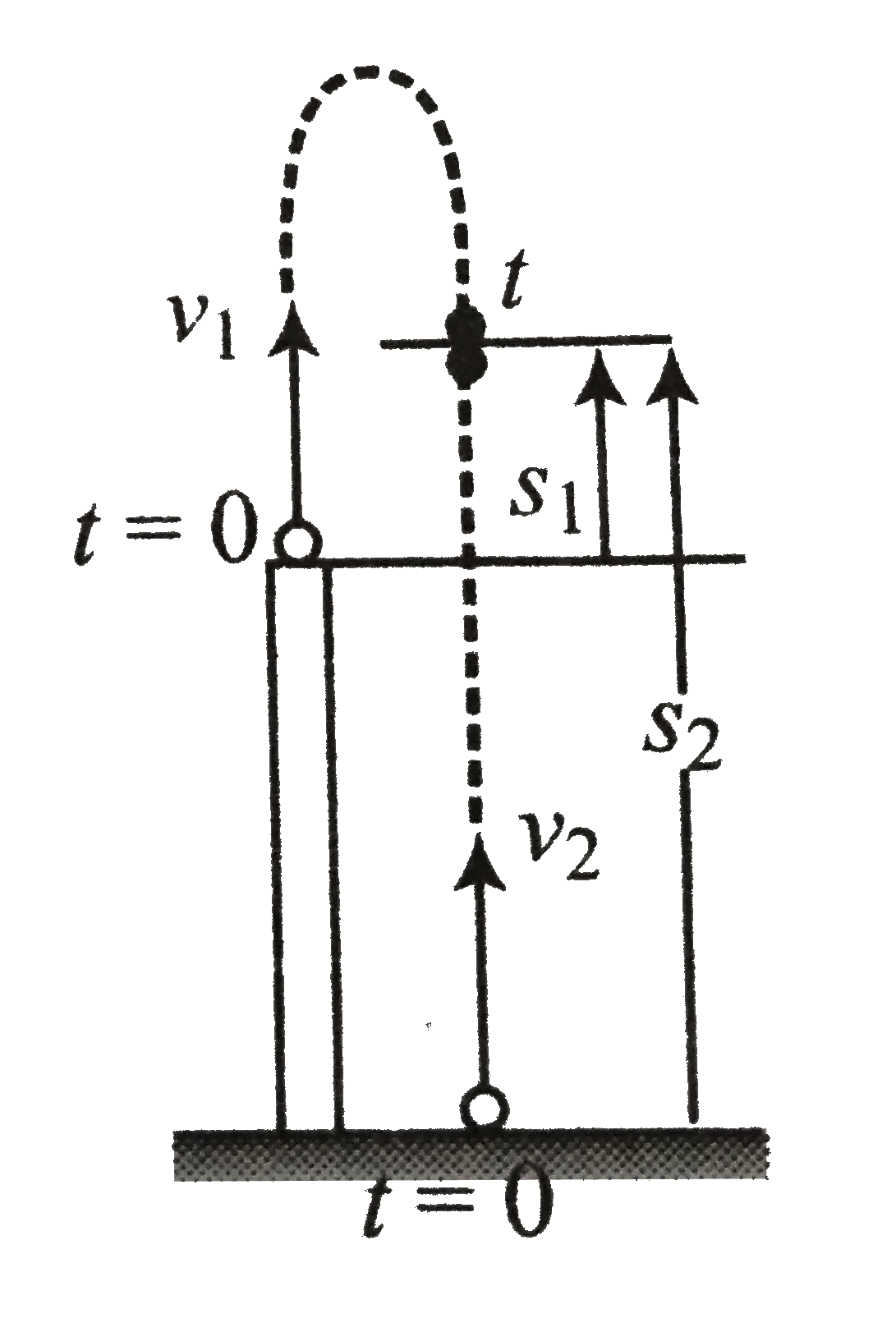
View SolutionTwo bodies 1 and 2 are projected simultaneously with velocities v1=2ms−1andv2=4ms−1 respectively. The body 1 is projected vertically up from the top of a cliff of height h = 10 and the body 2 is projected vertically up from the bottom of the cliff. If the bodies meet, find the time (in s) of meeting of the bodies.
View SolutionA stone projected vertically up with velocity v from the top of a tower reaches the ground with velocity 2 v The height of the tower
View SolutionA ball is dropped from the top of a building of height 80 m. At same instant another ball is thrown upwards with speed 50 m/s from the bottom of the building. The time at which balls will meet is
View SolutionA ball is projected vertically up with an initial velocity. Which of the following graphs represents the KE of the ball?
View Solution
