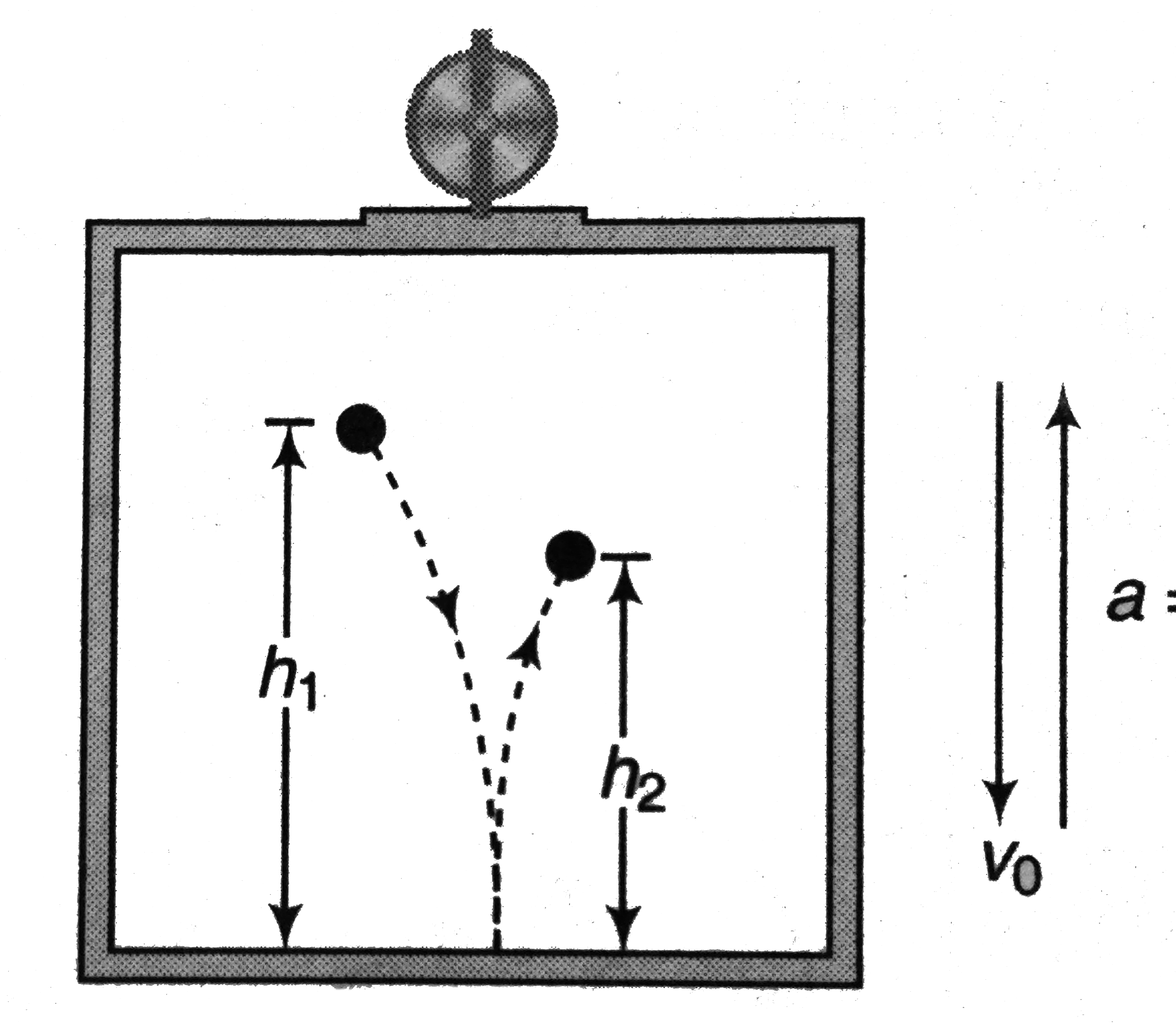
A ball is released from rest relative to the elevator at a distance $h_{1}$ above the floor. The speed of the elevator at the time of ball release is $v_{0}$ . Determine the bounce height $h_{2}$ relative to elevator of the ball (a) if $v_{0}$ is constant and (b) if an upward elevator accleration $a = \frac{g}{4}$ begins at the instant the ball is released. The coefficient of restitution for the impact is $e$ .

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B

Updated on:21/07/2023

A ball of mass $m$ is released from rest relative to elevator at a height $h$ , above the floor of the elevator. After making collision with the floor of the elevator it rebounces to height $h_{2}$ . The coefficient of restitution for Collision is $e$ . For this situation, mark the correct statement(s).

AIf elavator is moving done with constant velocity $v_{0}, t h e n h_{2} = e^{2} h_{1}$
BIf elevator is moving down with constasnt velocity $v_{0}$ , then $h_{2} = e^{2} h_{1} - \frac{v_{0}^{2}}{2 g}$
Cif elevator is moving down with constant velocity $v_{0}$ , then impulse imparted by floor of the elevator of the ball is $m (\sqrt{2 g h_{2}} + \sqrt{2 g h_{1}} + 2 v_{0})$ is the upward direction.
DIf elevator is moving with constant acceleration of $g / 4$ in upward direction, then it is not possible to determine a relation between $h_{1}$ and $h_{2}$ from the given information.
A ball released from rest from the roof of a building of height 45 m at t = 0. Find the height of ball from ground at t = 2s.
A10 m
B20 m
C25 m
D30 m
A ball is thrown straight upward with a speed v from a point h meter above the ground. The time taken for the ball to strike the ground is
A $\frac{v}{g} [1 + \sqrt{1 + \frac{2 h g}{v^{2}}}]$
B $\frac{v}{g} [1 - \sqrt{1 - \frac{2 h g}{v^{2}}}]$
C $\frac{v}{g} [1 - \sqrt{1 + \frac{2 h g}{v^{2}}}]$
D $\frac{v}{g} [2 + \frac{2 h g}{v^{2}}]$