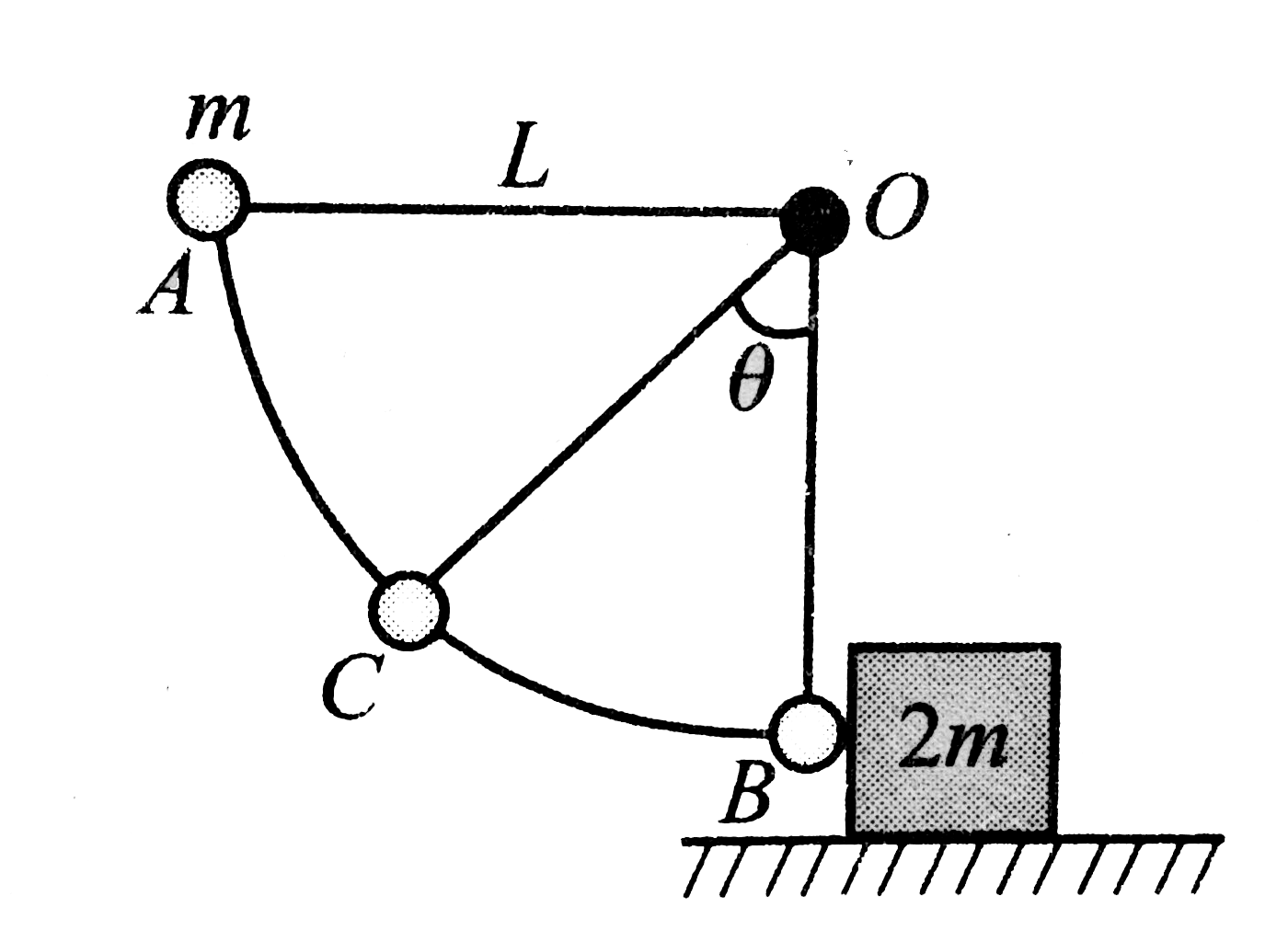
A ball of mass m is attached to a cord of length L, pivoted at point O, as shown in Fig. The ball is released from rest at point A, swings down and makes an inelastic collision with a block of mass 2m kept on a rough horizontal floor. The coefficient of restitution of collision is e=2/3 and coefficient of friction between block and surface is After collision, the ball comes momentarily to rest at C when cord makes an angle of θ with the vertical and block moves a distance of 3L/2 on rough horizontal floor before stopping. The values of μ and θ are, respectively,
50243,cos−1(8081)
5081,cos−1(8081)
281,cos−1(80243)
2243,cos−1(80243)
The correct Answer is:A
Velocity of the ball just before collision v0=√2gL
Applying momentum conservation along horizontal direction (because momentum is conserved in collision along the line of impact), we get
mv0=−mv1+2mv2
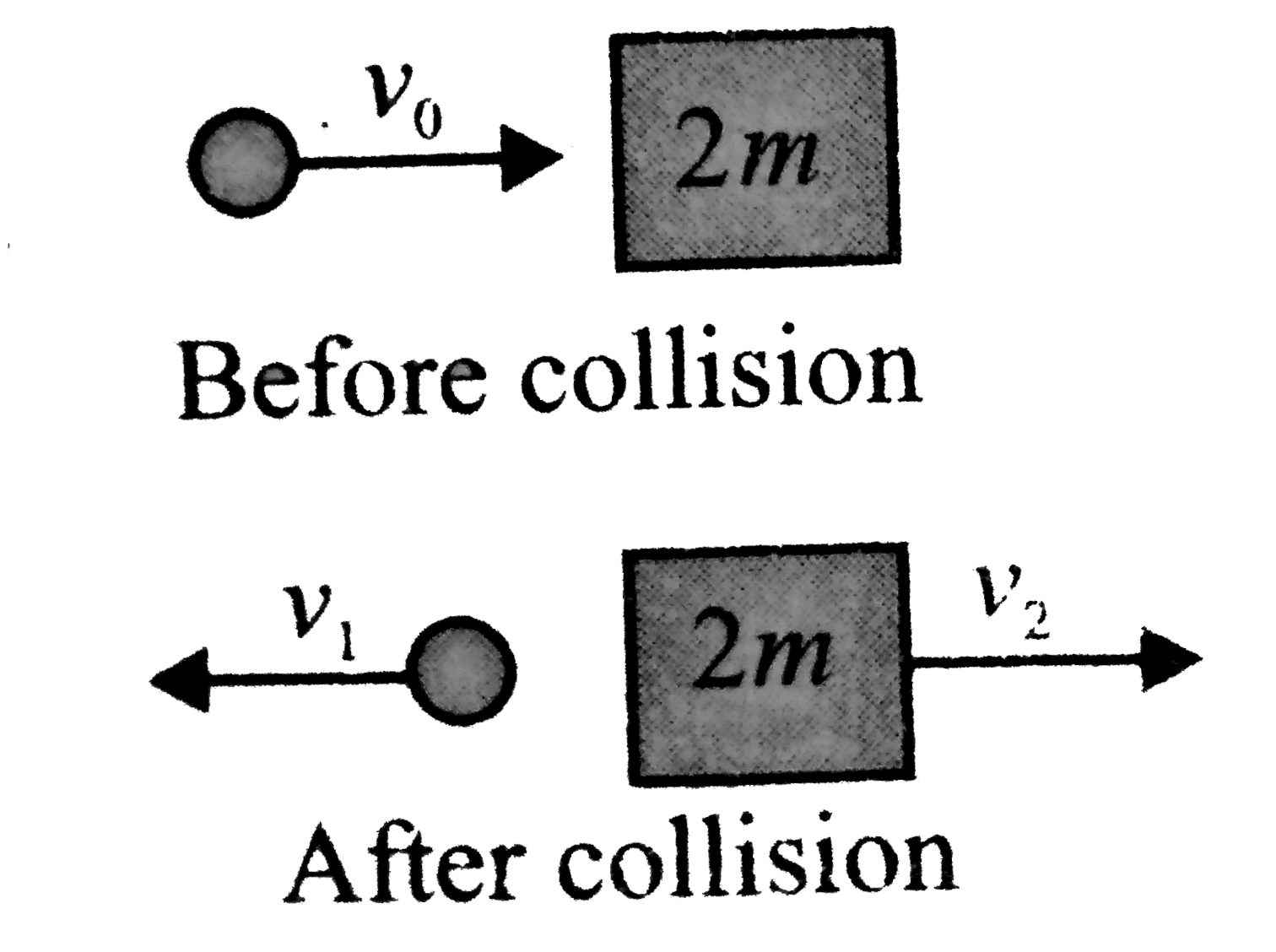
Applying coefficient of restitution equation, we get
e=23=v1+v2v0
Solving the above two equations we get
v1=v09 and v2=5v09
As the block moves a distance of 3L2 before coming to rest, so from work energy theorem,
0−12(2m)v22=−μ×2mg(3L)2⇒v22=3μgL
2581×2gL=3μgLimpliesμ=50243
For ball KE is converted to gravitational potential energy after collision, so
0−mv212=−mg×L(1−cosθ)
cosθ=8081
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Multiple Correct25videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion - Reasoning2videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Subjective23videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example13videos
CALORIMETRY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer2videos
DIMENSIONS & MEASUREMENT
Similar Questions
A block is kept on a rough horizontal surface as shown. Its mass is 2 kg and coefficient of friction between block and surface (μ)=0.5. A horizontal force F is acting on the block. When
 View Solution
View SolutionA block of mass m is moving on a rough horizontal surface. μ is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
View Solution
Knowledge Check
- Question 1 - Select One
A block slides with a velocity of 10 m/sec. on a rough horizontal surface. It comes to rest after covering of 50 m. If g is 10 m/sec2, then the coefficient of dynamic friction between the block and surface is
A1B10C2D0.1
A ball is dropped on a horizontal surface from height h. If it rebounds upto height h2 after first collision then coefficient of restitution between ball and surface is
View SolutionA block of mass 4 kg is kept on a rough horizontal surface. The coefficient of static friction is 0.8. If a forace of 19 N is applied on the block parallel to the floor, then the force of friction between the block and floor is
View SolutionA wooden block of mass M resting on a rough horizontal surface is pulled with a force T at an angle q to the horizontal. If m is coefficient of kinetic friction between the block and the surface, the acceleration of the block is
View SolutionA block of mass 2 kg is kept on a rough horizontal floor an pulled with a force F. If the coefficient of friction is 0.5. then the minimum force required to move the block is :-
 View Solution
View SolutionA ball hits a floor and rebounds after an inelastic collision. In this case
View SolutionA ball of mass 1kg is dropped from a height of 3.2m on smooth inclined plane. The coefficient of restitution for the collision is e=1/2. The ball's velocity become horizontal after the collision.
 View Solution
View SolutionA ball is dropped from a height h on a floor. The coefficient of restitution for the collision between the ball and the floor is e. The total distance covered by the ball before it comes to the rest.
View Solution

