A bar of copper of length and a bar of steel of length are joined together end to end. Both are of circular cross section with diameter . The free ends of the copper and the steel bars are maintained at and respectively. The curved surface of the bars are thermally insulated. What is the temperature of the copper-steel junction? What is the amount of heat transmitted per unit time across the junction? Thermal conductivity of copper is and that of steel is
To solve the problem, we need to find the temperature at the junction of the copper and steel bars and the amount of heat transmitted per unit time across the junction.
Step 1: Define the Variables
- Length of copper bar, Lc=75cm=0.75m
- Length of steel bar, Ls=125cm=1.25m
- Diameter of both bars, d=2cm=0.02m
- Radius of both bars, r=d2=0.01m
- Temperature at the free end of copper, Tc=100∘C
- Temperature at the free end of steel, Ts=0∘C
- Thermal conductivity of copper, kc=386J s−1m−1C−1
- Thermal conductivity of steel, ks=46J s−1m−1C−1
Step 2: Set Up the Heat Transfer Equation
Since the heat current (rate of heat flow) through both materials is the same, we can set up the equation using Fourier's law of heat conduction:
kc⋅A⋅(Tc−Tj)Lc=ks⋅A⋅(Tj−Ts)Ls
Where:
- Tj is the temperature at the junction.
- A is the cross-sectional area of the bars, which is the same for both bars.
Step 3: Calculate the Cross-Sectional Area
The cross-sectional area A is given by:
A=πr2=π(0.01)2=π×0.0001≈3.14×10−4m2
Step 4: Substitute Values into the Equation
Substituting the known values into the heat transfer equation:
386⋅(100−Tj)0.75=46⋅(Tj−0)1.25
Step 5: Simplify the Equation
Cross-multiplying to eliminate the fractions gives:
386⋅(100−Tj)⋅1.25=46⋅Tj⋅0.75
Expanding both sides:
482.5⋅(100−Tj)=34.5⋅Tj
Step 6: Solve for Tj
Distributing on the left side:
48250−482.5Tj=34.5Tj
Combining like terms:
48250=482.5Tj+34.5Tj
48250=517Tj
Now, solving for Tj:
Tj=48250517≈93.2∘C
Step 7: Calculate the Rate of Heat Flow
Now, we can calculate the rate of heat flow using either bar. Using the steel bar:
Qt=ks⋅A⋅(Tj−Ts)Ls
Substituting the values:
Qt=46⋅3.14×10−4⋅(93.2−0)1.25
Calculating:
Qt=46⋅3.14×10−4⋅93.21.25≈1.07J/s
Final Answers
- Temperature at the junction Tj≈93.2∘C
- Rate of heat flow Qt≈1.07J/s
- Length of copper bar, Lc=75cm=0.75m
- Length of steel bar, Ls=125cm=1.25m
- Diameter of both bars, d=2cm=0.02m
- Radius of both bars, r=d2=0.01m
- Temperature at the free end of copper, Tc=100∘C
- Temperature at the free end of steel, Ts=0∘C
- Thermal conductivity of copper, kc=386J s−1m−1C−1
- Thermal conductivity of steel, ks=46J s−1m−1C−1
Since the heat current (rate of heat flow) through both materials is the same, we can set up the equation using Fourier's law of heat conduction:
- Tj is the temperature at the junction.
- A is the cross-sectional area of the bars, which is the same for both bars.
The cross-sectional area A is given by:
Substituting the known values into the heat transfer equation:
Cross-multiplying to eliminate the fractions gives:
Distributing on the left side:
Now, we can calculate the rate of heat flow using either bar. Using the steel bar:
- Temperature at the junction Tj≈93.2∘C
- Rate of heat flow Qt≈1.07J/s
Topper's Solved these Questions
- HEAT TRANSFERBOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - OBJECTIVE I
10 Videos
- HEAT TRANSFERBOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - OBJECTIVE II
6 Videos
- HEAT TRANSFERBOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - QUESTIONS FOR SHORT ANSWER
11 Videos
- HEAT AND TEMPERATUREBOOK - HC VERMA ENGLISHCHAPTER - HEAT AND TEMPERATUREEXERCISE - Objective 2
6 Videos
- INTRODUCTION TO PHYSICSBOOK - HC VERMA ENGLISHCHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Question for short Answer
4 Videos
Similar Questions
A copper rod of length 75 cm and an iron rod of length 125cm are joined together end to end . Both are of circular cross section with diameter 2 cm . The free ends of the copper and iron are maintained at 100∘C and0∘C respectively . The surface of the bars are insulated thermally . The temperature of the copper -iron junction is [Thermal conductivity of the copper is 386.4W/m−K and that of iron is 48.46W/m−K].
View SolutionThree bars of equal lengths and equal areas of cross-section are connected in series. Their thermal conductivities are in the ratio of 2:4:3. If the open ends of the first and last bars are at temperatures 20∘Cand18∘C respectively in the steady state calculate the temperature of both the junctions.
View SolutionTwo rods of similar area of cross section and length are joined as shown in the figure. The ratio of their thermal conductivity is 2:3. The free ends are maintained at 100∘C and 0∘C respectivley. THE temperature at junction in steady state is
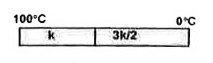
View SolutionTwo rods of same dimensions, but made of different materials are joined end to end to end with their free end being maintained at 100∘C and 0∘C respectively. The temperature of the junction is 70∘C. Then the temperature of the junction if the rods are inerchanged will be equal to T∘C Find T:
View SolutionFigure shows a copper rod joined to a steel rod. The rods have equal length and and the equal cross sectional area. The free end of the copper rod is kept at 0∘C and that of the steel rod is kept at 100∘C . Find the temperature at the junction of the rods. conductivity of copper =390Wm−1C−1 and that of steel =46Wm−1C−1 .

View SolutionFigure shows a copper rod joined to a steel rod. The rods have equal length and and the equal cross sectional area. The free end of the copper rod is kept at 0∘C and that of the steel rod is kept at 100∘C . Find the temperature at the junction of the rods. conductivity of copper =390WM−1.∘C−1 and that of steel =46Wm−1.∘C−1 .

View SolutionThree bars of equal lengths and equal area of cross-section are connected in series fig. their thermal conducitives are in the ratio 2:3:4. If at the steady state the open ends of the first and the last bars are at temperature 200∘C and 20∘C respectively, find the temperature of both the junctions.
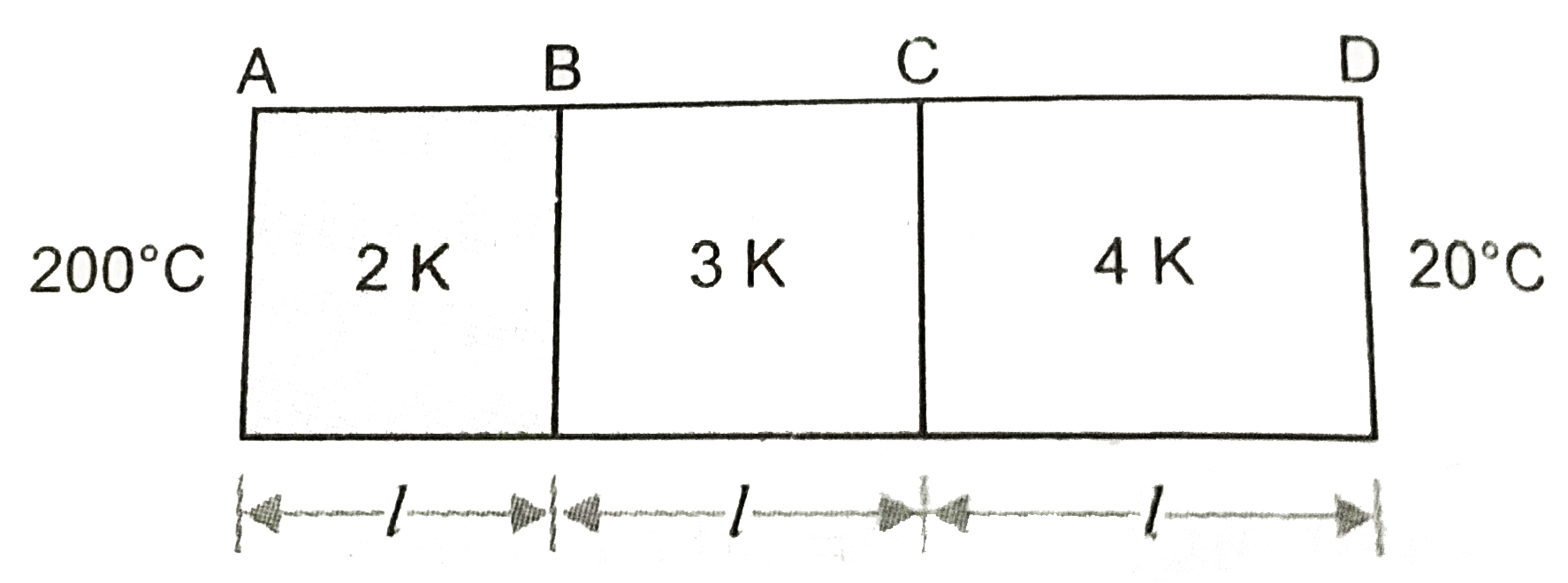 .
.
View SolutionOne face of a copper cube of edge 10 cm is maintained at 100∘C and the opposite face is maintained at 0∘C. All other surfaces are covered with an insulating material. Find the amount of heat flowing per second through the cube. Thermal conductivity of copper is 385Wm−1C−1.
View SolutionThree metal rods made of copper, aluminium and brass, each 20cm long 4cm in diameter, are placed end to end with aluminium between the other two. The free ends of copper and brass are maintained at 100 and 0∘C respectively. Assume that the thermal conductivity of copper is twice that of aluminium and four times that of brass. The approximately equilibrium temperatures of the copper-aluminiu and aluminium-brass junctions are respectively.

View SolutionThe ends of a copper rod of length 1m and area of cross-section 1cm2 are maintained at 0∘C and 100∘C. At the centre of the rod there is a source of heat of power 25 W. Calculate the temperature gradient in the two halves of the rod in steady state. Thermal conductivity of copper is 400Wm−1K−1.
View Solution
