A copper rod of length 75 cm and an iron rod of length 125cm are joined together end to end . Both are of circular cross section with diameter 2 cm . The free ends of the copper and iron are maintained at 100∘C and0∘C respectively . The surface of the bars are insulated thermally . The temperature of the copper -iron junction is [Thermal conductivity of the copper is 386.4W/m−K and that of iron is 48.46W/m−K].
100∘C
0∘C
93∘C
50∘C
The correct Answer is:C
To solve the problem, we will use the concept of heat conduction through two rods in series. The heat flow rate through both rods must be equal since they are connected end to end.
1. Identify Given Data:
- Length of copper rod, Lc=75cm=0.75m
- Length of iron rod, Li=125cm=1.25m
- Diameter of rods, d=2cm=0.02m
- Temperature at the free end of copper, Tc=100∘C
- Temperature at the free end of iron, Ti=0∘C
- Thermal conductivity of copper, kc=386.4W/m-K
- Thermal conductivity of iron, ki=48.46W/m-K
2. Calculate the Cross-Sectional Area (A):
The cross-sectional area of the rods can be calculated using the formula for the area of a circle:
A=πd24=π(0.02)24=π×0.00044=0.0004π4≈3.14×10−4m2
3. Set Up the Heat Flow Rate Equations:
The heat flow rate through the copper rod is given by:
dQdt=kcA(Tc−T)Lc
The heat flow rate through the iron rod is given by:
dQdt=kiA(T−Ti)Li
4. Equate the Heat Flow Rates:
Since the heat flow rates are equal:
kcA(Tc−T)Lc=kiA(T−Ti)Li
The area A cancels out from both sides:
kc(Tc−T)Lc=ki(T−Ti)Li
5. Substituting Known Values:
Substitute the known values into the equation:
386.4(100−T)0.75=48.46(T−0)1.25
6. Cross Multiply and Simplify:
Cross-multiplying gives:
386.4×1.25(100−T)=48.46×0.75T
Simplifying:
483.0(100−T)=36.345T
48300−483.0T=36.345T
48300=483.0T+36.345T
48300=519.345T
7. Solve for T:
T=48300519.345≈93.0°C
Final Answer:
The temperature of the copper-iron junction is approximately 93.0°C.
- Length of copper rod, Lc=75cm=0.75m
- Length of iron rod, Li=125cm=1.25m
- Diameter of rods, d=2cm=0.02m
- Temperature at the free end of copper, Tc=100∘C
- Temperature at the free end of iron, Ti=0∘C
- Thermal conductivity of copper, kc=386.4W/m-K
- Thermal conductivity of iron, ki=48.46W/m-K
The cross-sectional area of the rods can be calculated using the formula for the area of a circle:
A=πd24=π(0.02)24=π×0.00044=0.0004π4≈3.14×10−4m2
The heat flow rate through the copper rod is given by:
dQdt=kcA(Tc−T)Lc
The heat flow rate through the iron rod is given by:
dQdt=kiA(T−Ti)Li
Since the heat flow rates are equal:
kcA(Tc−T)Lc=kiA(T−Ti)Li
The area A cancels out from both sides:
kc(Tc−T)Lc=ki(T−Ti)Li
Substitute the known values into the equation:
386.4(100−T)0.75=48.46(T−0)1.25
Cross-multiplying gives:
386.4×1.25(100−T)=48.46×0.75T
Simplifying:
483.0(100−T)=36.345T
48300−483.0T=36.345T
48300=483.0T+36.345T
48300=519.345T
T=48300519.345≈93.0°C
The temperature of the copper-iron junction is approximately 93.0°C.
Similar Questions
A bar of copper of length 75cm and a bar of steel of length 125cm are joined together end to end. Both are of circular cross section with diameter 2cm. The free ends of the copper and the steel bars are maintained at 100∘C and 0∘C respectively. The curved surface of the bars are thermally insulated. What is the temperature of the copper-steel junction? What is the amount of heat transmitted per unit time across the junction? Thermal conductivity of copper is 386Js−1m−1C−1 and that of steel is 46Js−1m−1C−1
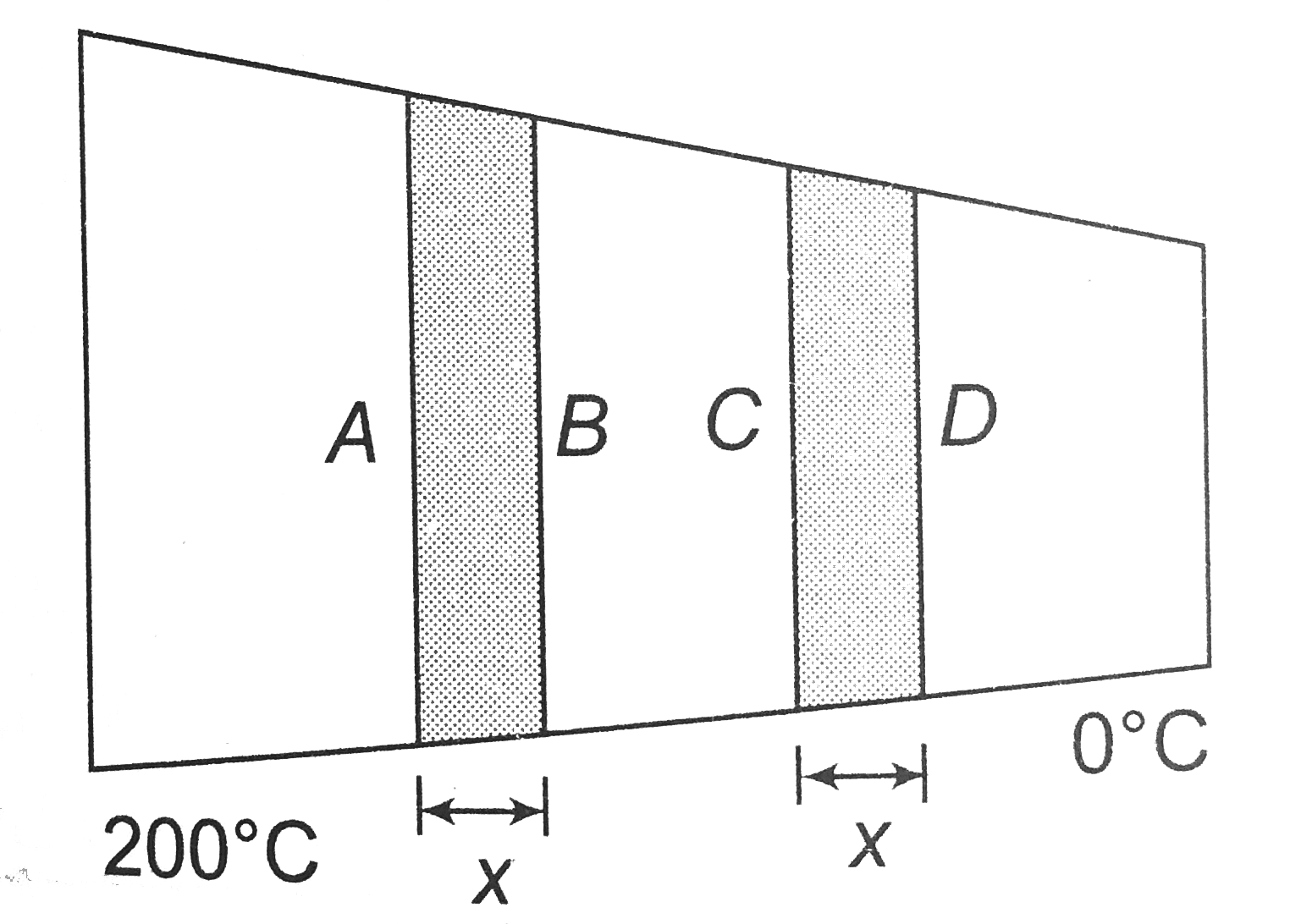
View SolutionTwo ends of a conducting rod of varying cross-section are maintained at 200∘C and 0∘C respectively. In steady state:
View SolutionThe ends of a copper rod of length 1m and area of cross-section 1cm2 are maintained at 0∘C and 100∘C. At the centre of the rod there is a source of heat of power 25 W. Calculate the temperature gradient in the two halves of the rod in steady state. Thermal conductivity of copper is 400Wm−1K−1.
View SolutionThe ends of a copper rod of length 1m and area of cross-section 1cm2 are maintained at 0∘C and 100∘C. At the centre of the rod there is a source of heat of power 25 W. Calculate the temperature gradient in the two halves of the rod in steady state. Thermal conductivity of copper is 400Wm−1K−1.
View SolutionThe ends of copper rod of length 1m and area of cross section 1cm2 are maintained at 0∘C and 100∘C. At the centre of rod, there is a source of heat of 32W. The thermal conductivity of copper is 400W/mK

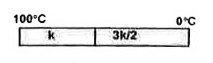
View SolutionTwo rods of similar area of cross section and length are joined as shown in the figure. The ratio of their thermal conductivity is 2:3. The free ends are maintained at 100∘C and 0∘C respectivley. THE temperature at junction in steady state is
View SolutionFigure shows a copper rod joined to a steel rod. The rods have equal length and and the equal cross sectional area. The free end of the copper rod is kept at 0∘C and that of the steel rod is kept at 100∘C . Find the temperature at the junction of the rods. conductivity of copper =390Wm−1C−1 and that of steel =46Wm−1C−1 .

View SolutionAssume that the thermal conductivity of copper is twice that of aluminium and four times that of brass. Three metal rods made of copper, aluminium and brass are each 15 cm long and 2 cm in diameter. These rods are placed end to end, with aluminium between the other two. The free ends of the copper and brass rods are maintained at 100∘C and 0∘C respectively. The system is allowed to reach the steady state condition. Assume there is no loss of heat anywhere.
When steady state condition is reached everywhere, which of the following statement is true?
View SolutionAssume that the thermal conductivity of copper is twice that of aluminium and four times that of brass. Three metal rods made of copper, aluminium and brass are each 15 cm long and 2 cm in diameter. These rods are placed end to end, with aluminium between the other two. The free ends of the copper and brass rods are maintained at 100∘C and 0∘C respectively. The system is allowed to reach the steady state condition. Assume there is no loss of heat anywhere.
When steady state condition is reached everywhere, which of the following statement is true?
View SolutionFigure shows a copper rod joined to a steel rod. The rods have equal length and and the equal cross sectional area. The free end of the copper rod is kept at 0∘C and that of the steel rod is kept at 100∘C . Find the temperature at the junction of the rods. conductivity of copper =390WM−1.∘C−1 and that of steel =46Wm−1.∘C−1 .

View Solution
