A barometer tube reads 76 cm of mercury. If the tube is gradually inclined at an angle of 60 with vertical, keeping the open end immersed in the mercury reservoir, the length of the mercury column will be
152 cm
76 cm
38 cm
38√3cm
The correct Answer is:A
To solve the problem of determining the length of the mercury column in an inclined barometer tube, we can follow these steps:
1. Understand the Problem: We have a barometer tube that reads 76 cm of mercury when vertical. When the tube is inclined at an angle of 60 degrees with the vertical, we need to find the new length of the mercury column.
2. Identify the Variables:
- Let Sv = height of the mercury column in the vertical tube = 76 cm.
- Let Si = height of the mercury column in the inclined tube.
- Let H = vertical height of the mercury column when inclined.
- Angle of inclination θ=60∘.
3. Use the Relationship Between Heights:
- In the vertical tube, the pressure is given by the formula P=ρgh, where h is the vertical height of the mercury column.
- For the inclined tube, the vertical height H can be related to the inclined length Si using trigonometry:
H=Sicos(θ)
- Thus, we can rearrange this to find Si:
Si=Hcos(θ)
4. Set Up the Pressure Equality:
- The pressure exerted by the mercury column must be equal in both cases:
ρgH=ρgSv
- Since ρg cancels out, we have:
H=Sv
- Therefore, H=76cm.
5. Calculate Si:
- Substitute H into the equation for Si:
Si=Hcos(60∘)
- We know that cos(60∘)=12:
Si=76cm12=76cm×2=152cm
6. Conclusion:
- The length of the mercury column when the tube is inclined at 60 degrees is Si=152cm.
Final Answer:
The length of the mercury column in the inclined barometer tube is 152 cm.
- Let Sv = height of the mercury column in the vertical tube = 76 cm.
- Let Si = height of the mercury column in the inclined tube.
- Let H = vertical height of the mercury column when inclined.
- Angle of inclination θ=60∘.
- In the vertical tube, the pressure is given by the formula P=ρgh, where h is the vertical height of the mercury column.
- For the inclined tube, the vertical height H can be related to the inclined length Si using trigonometry:
H=Sicos(θ)
- Thus, we can rearrange this to find Si:
Si=Hcos(θ)
- The pressure exerted by the mercury column must be equal in both cases:
ρgH=ρgSv
- Since ρg cancels out, we have:
H=Sv
- Therefore, H=76cm.
- Substitute H into the equation for Si:
Si=Hcos(60∘)
- We know that cos(60∘)=12:
Si=76cm12=76cm×2=152cm
- The length of the mercury column when the tube is inclined at 60 degrees is Si=152cm.
The length of the mercury column in the inclined barometer tube is 152 cm.
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced
57 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - More than one option is correct
21 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
Similar Questions
A barometer tube reads 76 cm of Hg. If tube is : gradually inclined at an angle of 60∘ with vertical, keeping the open end in the mercury reservoir, the length of mercury column will be
View SolutionA barometer tube reads 76 cm of mercury. If the tube is gradually inclined keeping the open end immersed in the mercury reservoir, will the length of mercury column be 76 cm, more than 76 cm or less than 76 cm?
View Solution
Knowledge Check
A thin tube of uniform cross-section is sealed at both ends. It lies horizontally, the middle 5 cm containing mercury and the two equal end containing air at the same pressure P. When the tube is held at an angle of 60∘ with the vetical direction, the length of the air column above and below the mercury column are 46 cm and 44.5 cm respectively. Calculate the pressure P in centimeters of mercury. (The temperature of the system is kept at 30∘C).
A75.4B45.8C67.5D89.3
Water rises in a capillary tube to a height 4 cm. If the tube is inclined at an angle of 45∘ with the vertical , find the position of the water in the tube.
View Solution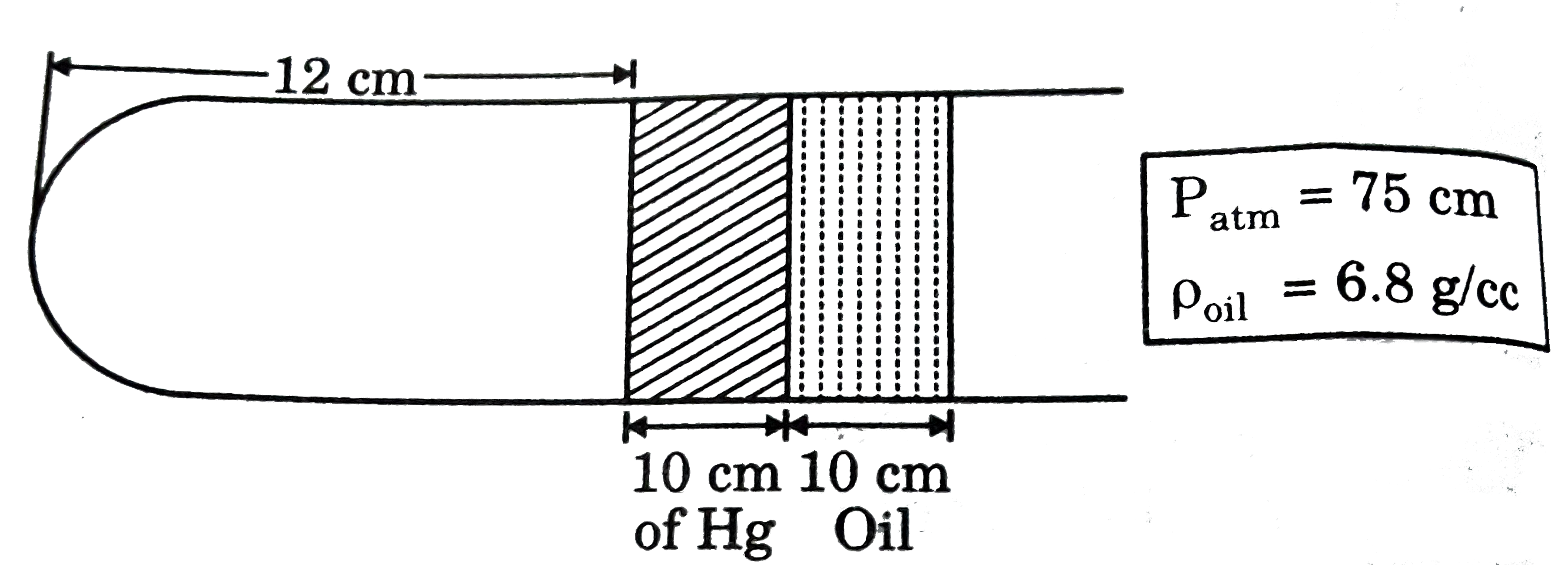
If above tube is placed vertically with the open and upward then find the length of the air column.
View Solution
If the above tube is kept in vertical position by how much length the mercury column descends ?
View SolutionWater rises in a vertical capillary tube up to a height of 2.0 cm. If the tube is inclined at an angle of 60∘ with the vertical, then up to what length the water will rise in the tube ?
View SolutionAir is trapped in a horizontal glass tube by 36 cm mercury column as shown below :
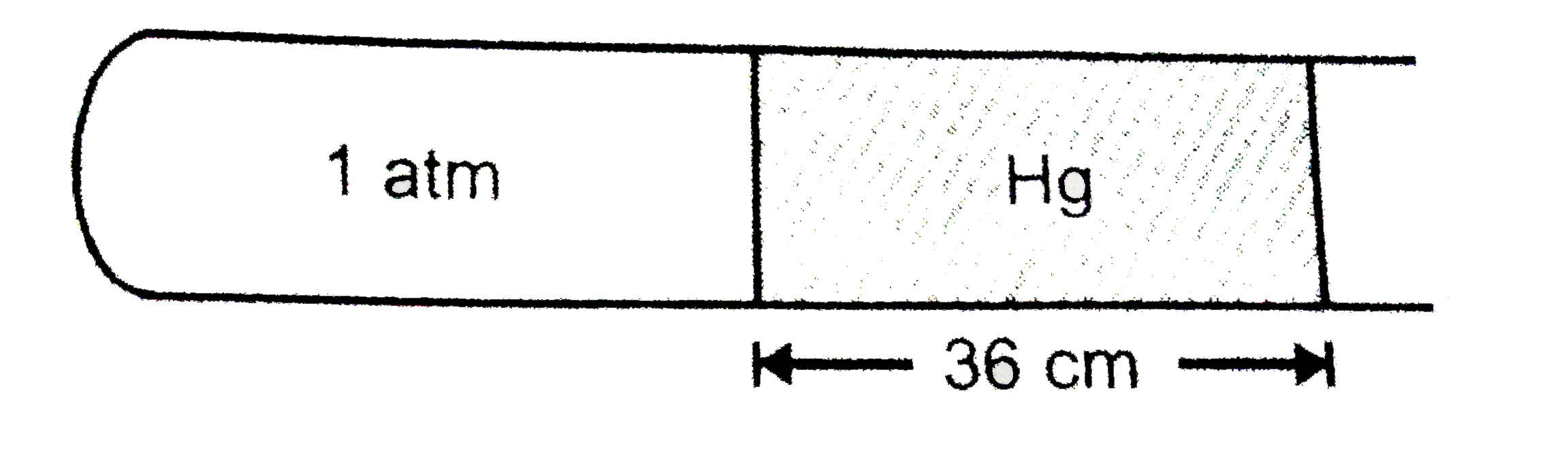 If the tube is held vertical keeping the open end up, lengh of air column shrink to 19 cm. What is the lengh (in cm) by which the mercury column shifts down?
If the tube is held vertical keeping the open end up, lengh of air column shrink to 19 cm. What is the lengh (in cm) by which the mercury column shifts down?
View SolutionA column of mercury is in the middle of horizontal tube of uniform cross section closed at both ends. The pressure of the enclosed air column on either side of the mercury is 75 cm of mercury column. When the tuve is placed vertical the length air below the mercury column is 35 th of that above it. if length of mercury column is 10n cm. find n.
View SolutionA thin tube of uniform cross-section is sealed at both ends. It lies horizontally, the middle 5 cm containing mercury and the two equal end containing air at the same pressure P. When the tube is held at an angle of 60∘ with the vetical direction, the length of the air column above and below the mercury column are 46 cm and 44.5 cm respectively. Calculate the pressure P in centimeters of mercury. (The temperature of the system is kept at 30∘C).
View Solution
