A batsman hits back a ball straight in the direction of the bowler without changing its initial speed of 12ms−1 . If the mass of the ball is 0.15 kg , determine the impulse imparted to the ball . (Assume linear motion of the ball).
1.8N s
2.8N s
3.6N s
4.2N s
The correct Answer is:C
To determine the impulse imparted to the ball, we can follow these steps:
Step 1: Understand the concept of impulse
Impulse is defined as the change in momentum of an object. Mathematically, it can be expressed as:
Impulse=Δp=pf−pi
where pf is the final momentum and pi is the initial momentum.
Step 2: Calculate the initial momentum
The initial momentum pi of the ball can be calculated using the formula:
pi=m⋅vi
where:
- m is the mass of the ball (0.15 kg)
- vi is the initial velocity of the ball (12 m/s)
So,
pi=0.15kg⋅12m/s=1.8kg m/s
Step 3: Calculate the final momentum
When the batsman hits the ball back, the direction of the ball changes. The final velocity vf of the ball will be -12 m/s (negative because it is in the opposite direction). Thus, the final momentum pf is:
pf=m⋅vf
So,
pf=0.15kg⋅(−12)m/s=−1.8kg m/s
Step 4: Calculate the change in momentum
Now, we can find the change in momentum:
Δp=pf−pi
Substituting the values we calculated:
Δp=−1.8kg m/s−1.8kg m/s=−3.6kg m/s
Step 5: Determine the impulse
The impulse imparted to the ball is equal to the change in momentum:
Impulse=−3.6kg m/s
Since impulse is often expressed in terms of magnitude, we can say:
Impulse=3.6N s
Final Answer
The impulse imparted to the ball is 3.6 N s.
---
Impulse is defined as the change in momentum of an object. Mathematically, it can be expressed as:
Impulse=Δp=pf−pi
where pf is the final momentum and pi is the initial momentum.
The initial momentum pi of the ball can be calculated using the formula:
pi=m⋅vi
where:
- m is the mass of the ball (0.15 kg)
- vi is the initial velocity of the ball (12 m/s)
pi=0.15kg⋅12m/s=1.8kg m/s
When the batsman hits the ball back, the direction of the ball changes. The final velocity vf of the ball will be -12 m/s (negative because it is in the opposite direction). Thus, the final momentum pf is:
pf=m⋅vf
So,
pf=0.15kg⋅(−12)m/s=−1.8kg m/s
Now, we can find the change in momentum:
Δp=pf−pi
Substituting the values we calculated:
Δp=−1.8kg m/s−1.8kg m/s=−3.6kg m/s
The impulse imparted to the ball is equal to the change in momentum:
Impulse=−3.6kg m/s
Impulse=3.6N s
The impulse imparted to the ball is 3.6 N s.
Topper's Solved these Questions
- LAWS OF MOTIONBook:NCERT FINGERTPS ENGLISHChapter:LAWS OF MOTIONExercise:HOTS
Explore 8 Videos
- LAWS OF MOTIONBook:NCERT FINGERTPS ENGLISHChapter:LAWS OF MOTIONExercise:Exemplar Problems
Explore 9 Videos
- KINETIC THEORYBook:NCERT FINGERTPS ENGLISHChapter:KINETIC THEORYExercise:Assertion And Reason
Explore 10 Videos
- MECHANICAL PROPERTIES OF FLUIDSBook:NCERT FINGERTPS ENGLISHChapter:MECHANICAL PROPERTIES OF FLUIDSExercise:NCERT Exemplar
Explore 5 Videos
Similar Questions
Knowledge Check
Two billiard ball A and B each of mass 50g and moving in opposite direction with speed of 5ms−1 each, collide and rebound with the same speed. The impulse imparted to each ball is
A0.25kgms−1B0.5kgms−1C0.8kgms−1D0.125kgms−1A ball of mass m strikes a rigid walJ with speed u and rebounds with the same speed. The impulse imparted to the ball by the wall is
A2muBmuCzeroD−2mu
A batsman deflects a ball by an angle of 45∘ without changing its initial speed which is equal to 54kmh. What is the impulse imparted to the ball ? (Mass of the ball is 0.15 kg)
View SolutionA batsman deflects a ball by an angle of 90∘ without changing its initial speed, which is equal to 54 km/hr. What is the impulse to the ball ? Mass of the ball is 0.15 kg.
View SolutionA bowler tbrows a ball horizontally along east direction with speed of 144km/hr. The batsman hits the ball such that it deviates from its initial direction of motion by 74∘ north of east direction, without changing its speed. If mass of the ball is 13kg and time of contact between bat and ball is 0.02 s. Average force applied by batsman on ball is:
A. 800 N, 53∘East of North
B. 800N, 53∘ North of East
C. 800 N, 53∘ North of West
D. 800N, 53∘ West of North
View SolutionCalculate the de Broglie wavelength of a ball of mass 0.1kg moving with a speed of 30ms−1
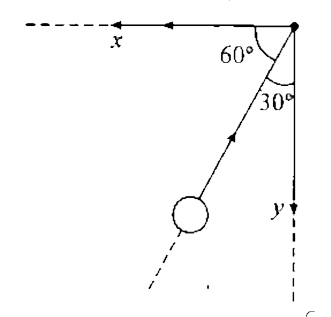
View SolutionA hockey player receives a corner shot at a speed of 15m/s at an angle of 30∘ with the y-axis and then shoots the ball along the x-axis with a speed of 30m/s If the mass of the ball is 150gm and it remains in contact with the hockey stick for 0.01s, the force imparted to the ball in the x-direction is :
View SolutionTwo billiard balls each of mass 0.05 kg moving in opposite directions with speed 6ms−1 collide and rebound with the same speed. What is the impulse imparted to each ball due to the other ?
View Solution