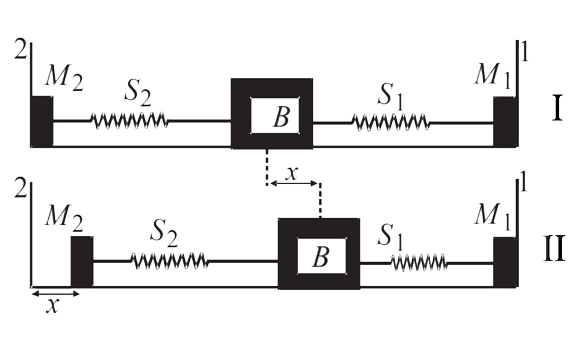
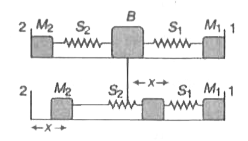
A block (B) is attached to two unstriched sprig S1 and S_(2) with spring constant K and 4K , respectively (see fig 1) The other ends are atteched in identical support M1 and M2 not attached in the walls . The springs and supports have negligible mass . There is no friction anywhere . The block B is displaced toword wall 1 by a small distance z (figure (ii)) and released . THe block return and moves a maximum displacements x and y are musured with reoact to the equalibrum of the block B and the ratio y/x is
4
2
(c) 12
(d) 14
The correct Answer is:C
From energy conservation, 12kx2=12(4k)y2
∴yx=12
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives(multiple Correct)3videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives (assertion-reasoning)1videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Integer14videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
A simple pendulum of length L and mass (bob) M is oscillating in a plane about a vertical line between angular limit −ϕ and +ϕ. For an angular displacement θ(|θ|<ϕ), the tension in the string and the velocity of the bob are T and V respectively. The following relations hold good under the above conditions:
06:00View SolutionIn the following questions, each question contains Statement I(assertion) and StatementII(reason). Each questions has four choices a, b, c, and d out of which only one is correct.
Statement I: A block of mass m starts moving on a rough horizontal surface with a velocity v. It stops due to friction between the block and the surface after moving through a certain distance. The surface is now titled to an angle of 30∘ with the horizontal and the same block is made to go up on the surface with the same initial velocity v. The decrease in mechanical energy in the second situation is smaller than that in the first situation.
Statement II: The coefficient of friction between the block and the surface decreases with the increase in the angel of inclination.View SolutionIn the situation shown in figure all contact surfaces are smooth. The force constant of the spring is K. Two forces F are applied as shown. The maximum elongation produced in the spring is how many times of F/K (initially the spring is relaxed)?
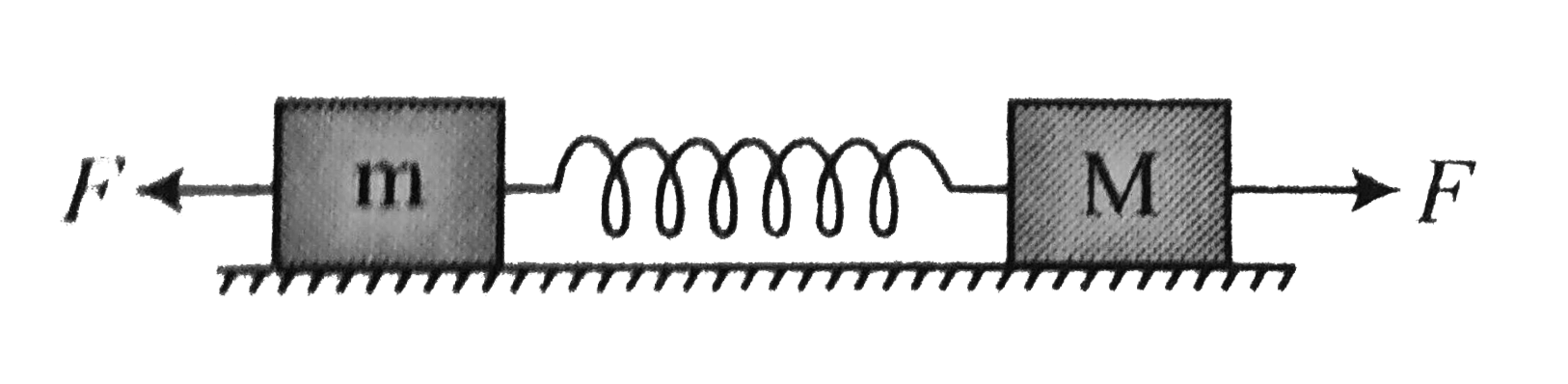 02:00View Solution
02:00View SolutionWhich of the following statements is/ are correct(Figure)
 03:37View Solution
03:37View SolutionA block (B) is attached to two unstriched sprig S_(1) and S_(2) with spring constant K and 4K , respectively (see fig 1) The other ends are atteched in identical support M_(1) and M_(2) not attached in the walls . The springs and supports have negligible mass . There is no friction anywhere . The block B is displaced toword wall 1 by a small distance z (figure (ii)) and released . THe block return and moves a maximum displacements x and y are musured with reoact to the equalibrum of the block B and the ratio y//x is
04:45View SolutionTwo blocks of masses m1 and m2, connected by a weightless spring of stiffness k rest on a smooth horizontal plane as shown in Fig. Block 2 is shifted a small distance x to the left and then released. Find the velocity of centre of mass of the system after block 1 breaks off the wall.
 04:01View Solution
04:01View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force.
01:51View SolutionTwo masses m1 and m2 are attached to a spring balance S as shown in figure. m1>m2 then the reading of spring balance will be .
 .04:44View Solution
.04:44View SolutionIn Fig 3E.17, the mass m2 rests on a rough table. The mass m1 is pushed against the spring to which it is not attached Force constant of the spring is k, coefficient of friction is μk.
(a) Find the speed of the blocks after the spring is released and m2 has fallen a distance of h.
(b) If the spring is attached to the block and it falls a distance h before coming to rest, calculate the coefficient of friction μk.
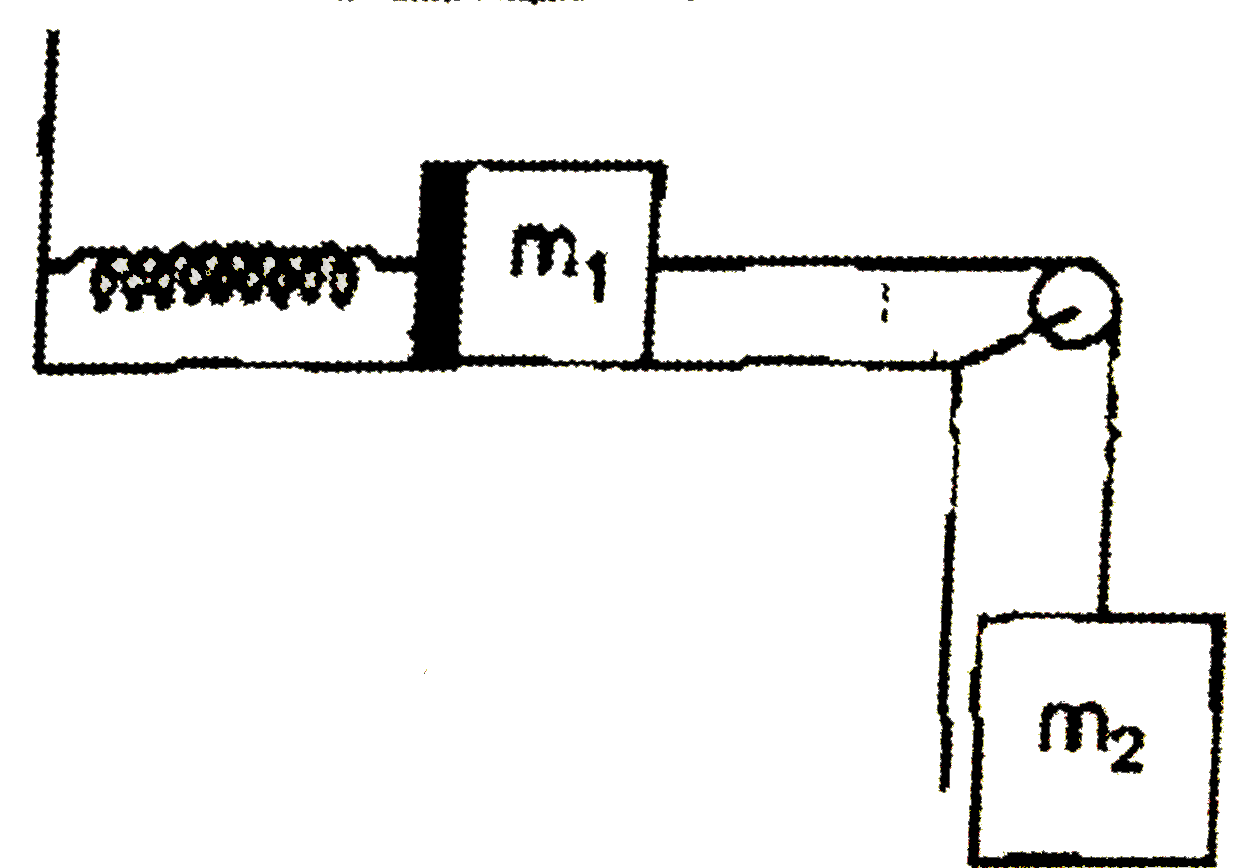 02:52View Solution
02:52View SolutionTwo blocks of masses m_(1) and m_(2) are kept on a smooth horizontal table as shown in figure. Block of mass m_(1) (but not m_(2) is fastened to the spring. If now both the blocks are pushed to the left so that the spring is compressed a distance d. The amplitude of oscillatin of block of mass m_(1) after the system is released is
View SolutionSpring of spring constant k is attached with a block of mass m_(1) , as shown in figure. Another block of mass m_(2) is placed angainst m_(1) , and both mass masses lie on smooth incline plane. Find the compression in the spring when lthe system is in equilibrium.
08:35View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in the figure. The time period of oscillation is
View Solutionएक गुटका (B) k तथा 4k स्प्रिंग नियतांक वाले दो स्प्रिंगों से चित्रानुसार जुड़ा है। स्प्रिंगों के दूसरे सिरे चित्रानुसार M1 व M2 से जुड़ें हुए हैं। स्प्रिंगे M1 व M2 भारहीन हैं। पूरा निकाय घर्षणहीन हैं। गुटके (B ) को दिवार-I की ओर अल्प दूरी x विस्थापित करके छोड़ दिया जाता है। ब्लॉक वापसी जाता है और दीवार 2 की ओर अधिकतम दूरी y ले जाता है, चित्र में x तथा y दूरियाँ माध्य-स्थिति के सापेक्ष नापी जाती हैं। yx का मान हैं
 01:46View Solution
01:46View SolutionTwo masses m_(1) and m_(2) are attached to a spring balance S as shown in Figure. If m_(1)gt m_(2) then the reading of spring balance will be
02:50View SolutionA mass m is attached to two identical springs each having spring constant k is in equilibrium as shown in the figure.The work done to lift the block upward at a distance (mg)/(4k) is (1)/(k)((mg)/(n))^(2) find n? (Distance between supports is sum of natural length of springs)
03:01View SolutionTwo blocks of masses m1 and m2 are connected by a spring having spring constant K. Initially , the spring is at its natural length. The coefficient of friction between the blocks and the surface is μ. What minimum constant force has to be applied in the horizontal direction to the block of mass m1, in order to shift the other block ?
05:10View Solution

