A block is tied to one end of a light string of length 1 whose other end is fixed to a rigid support. The block is given a speed of √7gl/2 from the lowermost position. Find the height and speed at which the block leaves the circle. Also find the maximum height to which it rises finally.
To solve the problem step by step, we will use the principles of energy conservation and circular motion.
Step 1: Identify the initial conditions
The block is given an initial speed u=√7gL2 at the lowest position of the circular path.
Step 2: Determine the height at which the block leaves the circular path
The block will leave the circular path when the tension in the string becomes zero. At this point, the only forces acting on the block are gravity and the centripetal force required to keep it moving in a circle.
Using the work-energy theorem, we can write:
Work done by gravity=ΔKE
The work done by gravity when the block rises to height h is:
W=−mgh=−mg(L+Lcosθ)
The change in kinetic energy is:
ΔKE=12mv2−12mu2
Setting these equal gives:
−mg(L+Lcosθ)=12mv2−12mu2
Canceling m and rearranging:
v2=u2−2g(L+Lcosθ)
Step 3: Apply the centripetal force condition
At the point where the block leaves the circular path, the centripetal force is provided by the component of gravitational force:
mgcosθ=mv2L
Cancelling m gives:
gcosθ=v2L
Substituting v2 from the previous equation:
gcosθ=u2−2g(L+Lcosθ)L
Step 4: Substitute u2 and solve for θ
Substituting u2=7gL2:
gcosθ=7gL2−2g(L+Lcosθ)L
Simplifying:
gcosθ=7gL−4gL−4gLcosθ2L
gcosθ=3gL−4gLcosθ2L
Multiplying through by 2L:
2gLcosθ=3gL−4gLcosθ
6gLcosθ=3gL
cosθ=12
Thus, θ=60∘.
Step 5: Calculate the speed at the point of leaving the circle
Substituting cosθ back into the equation for v2:
v2=u2−2g(L+Lcos60∘)
v2=7gL2−2g(L+L2)=7gL2−3gL=gL2
Thus, v=√gL2.
Step 6: Calculate the maximum height reached after leaving the circle
Using projectile motion, the maximum height H can be calculated using:
H=v2sin2θ2g
Substituting v=√gL2 and θ=60∘:
H=(gL2)(34)2g=3L8
Step 7: Total height from the ground
The total height from the ground is:
Total Height=L+Lcos60∘+H=L+L2+3L8
Calculating this gives:
Total Height=L+L2+3L8=8L8+4L8+3L8=15L8
Final Answers:
1. Height at which the block leaves the circle: 3L2
2. Speed at which the block leaves the circle: √gL2
3. Maximum height reached: 15L8
The block is given an initial speed u=√7gL2 at the lowest position of the circular path.
The block will leave the circular path when the tension in the string becomes zero. At this point, the only forces acting on the block are gravity and the centripetal force required to keep it moving in a circle.
Work done by gravity=ΔKE
The work done by gravity when the block rises to height h is:
W=−mgh=−mg(L+Lcosθ)
The change in kinetic energy is:
ΔKE=12mv2−12mu2
Setting these equal gives:
−mg(L+Lcosθ)=12mv2−12mu2
Canceling m and rearranging:
v2=u2−2g(L+Lcosθ)
At the point where the block leaves the circular path, the centripetal force is provided by the component of gravitational force:
mgcosθ=mv2L
Cancelling m gives:
gcosθ=v2L
Substituting v2 from the previous equation:
gcosθ=u2−2g(L+Lcosθ)L
Substituting u2=7gL2:
gcosθ=7gL2−2g(L+Lcosθ)L
Simplifying:
gcosθ=7gL−4gL−4gLcosθ2L
gcosθ=3gL−4gLcosθ2L
Multiplying through by 2L:
2gLcosθ=3gL−4gLcosθ
6gLcosθ=3gL
cosθ=12
Thus, θ=60∘.
Substituting cosθ back into the equation for v2:
v2=u2−2g(L+Lcos60∘)
v2=7gL2−2g(L+L2)=7gL2−3gL=gL2
Thus, v=√gL2.
Using projectile motion, the maximum height H can be calculated using:
H=v2sin2θ2g
Substituting v=√gL2 and θ=60∘:
H=(gL2)(34)2g=3L8
The total height from the ground is:
Total Height=L+Lcos60∘+H=L+L2+3L8
Calculating this gives:
Total Height=L+L2+3L8=8L8+4L8+3L8=15L8
1. Height at which the block leaves the circle: 3L2
2. Speed at which the block leaves the circle: √gL2
3. Maximum height reached: 15L8
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Solved Examples
15 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.1
25 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A block of mass 1kg is tied to a string of length 1m the other end of which is fixed The block is moved on a smooth horizontal table with constant speed 10ms−1. Find the tension in the string
View SolutionA bob of mass m suspended by a light inextensible string of length 'l' from a fixed point. The bob is given a speed of √6gl. Find the tension in the string when string deflects through an angle 120∘ from the vertical.
View SolutionA stone is tied to one end of a light inexensible string of length l and made to roate on a vertical circle keeping other end of the spring at the centre. If speed of stone at the highest point is v(v>√gl) then its speed at the lowest point is
View SolutionA block of mass m is attached to one end of a mass less spring of spring constant k. the other end of spring is fixed to a wall the block can move on a horizontal rough surface. The coefficient of friction between the block and the surface is μ then the compession of the spring for which maximum extension of the spring becomes half of maximum compression is .
View SolutionA block of mass M=1 kg resting on a smooth horizontal surface is connected to a horizontal light spring of spring constant k=6N/m whose other end is fixed to a verticall wall. Another block of mass m=0.5 kg is placed over the block M. If the coefficient of friction between the two blocks μ=0.4, find the maximum kinetic energy (in J) that the system can have for simple hormonic oscillation under the action of the spring.
View SolutionA block of mass 1 kg is attached to one end of a spring of force constant k = 20 N/m. The other end of the spring is attached to a fixed rigid support. This spring block system is made to oscillate on a rough horizontal surface (μ=0.04). The initial displacement of the block from the equilibrium position is a = 30 cm. How many times the block passes from the mean position before coming to rest ? (g=10m/s2)
View SolutionA block of mass m, lying on a smooth horizontal surface, is attached to a spring (of negligible mass) of spring constant k. The other end of the spring is fixed, as shown in the figure. The block is initally at rest in its equilibrium position. If now the block is pulled withe a constant force F, the maximum speed of the block is :

View SolutionA 2 kg block A is attached to one end of a light string that passes over an an ideal pulley and a 1 kg sleeve B slides down the other part of the string with an acceleration of 5m/s2 with respect to the string. Find the acceleration of the block, acceleration of sleeve and tension in the steing. [g=10m/s2]
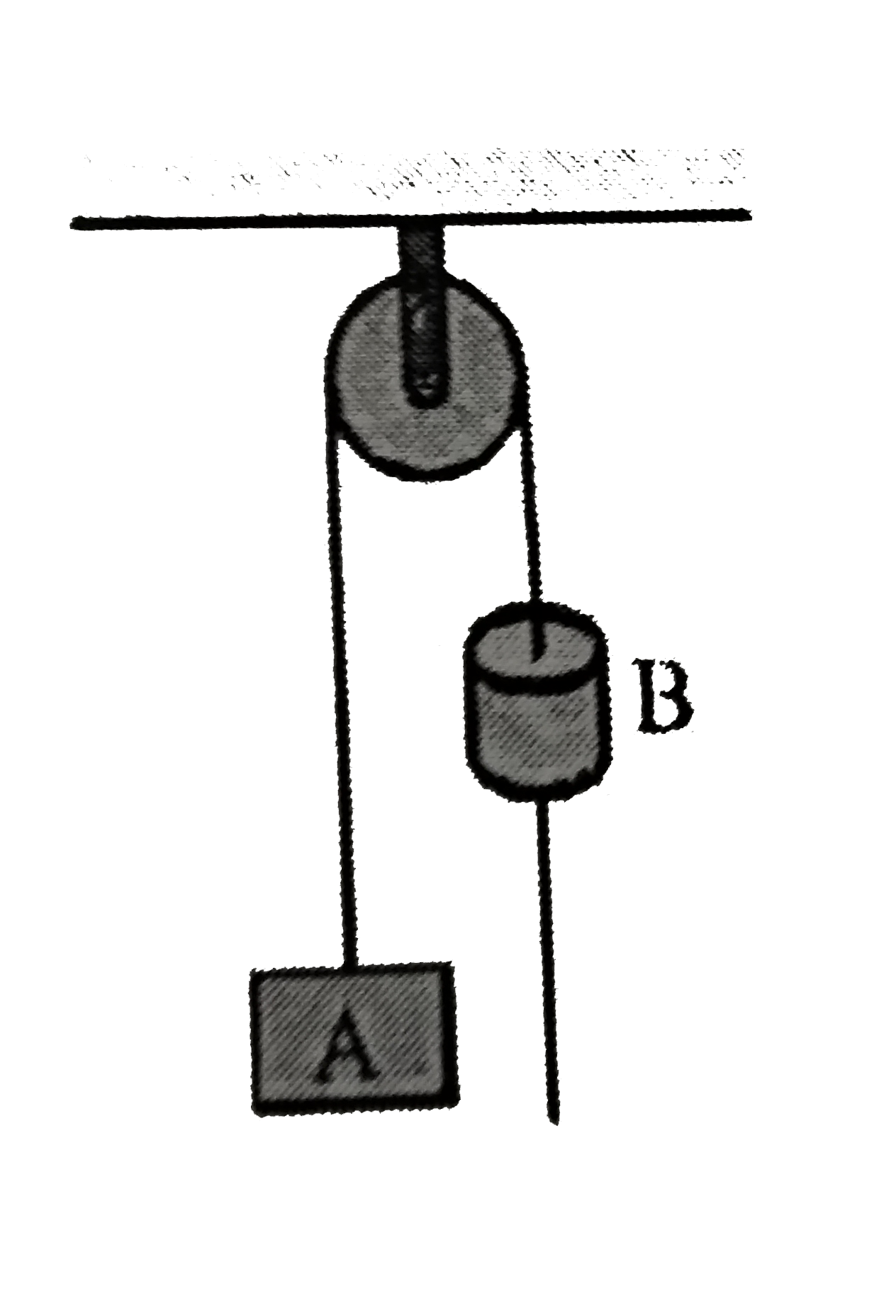
View SolutionA particle is rotated in a vertical circle by connecting it to a string of length l and keeping the other end of the string fixed. The minimum speed of the particle when the string is horizontal for which the particle will complete the circle is
View SolutionIn the figure shown, a person pulls a light string with a constant speed u=10m/s. The other end of the string is tied to a very small block which moves on a smooth horizontal surface. Find the angle 'θ'when the block 3 leaves the surface . Take g=10m/s2.
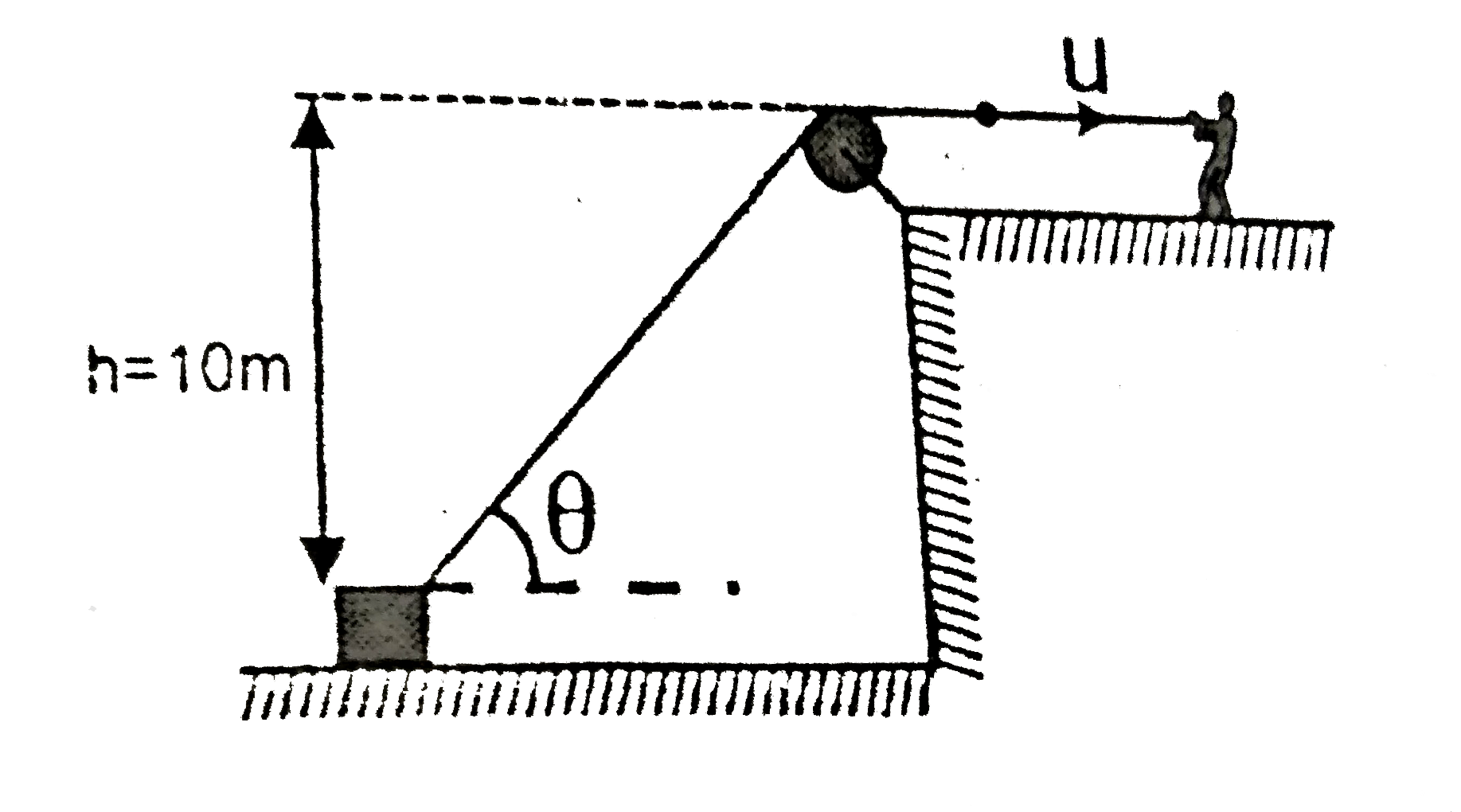
View Solution