A block of mass m=3kg is resting over a rough horizontal surface having coefficient of friction μ=1/3 The block is pulled to the right by appliying a force F inclined at angle 37∘ with the horizontal as shown in fig .The forceincreases with time according to law F=2r newton Calculate its velocity v at t=10s(g=10ms−2)

The correct Answer is:253ms−1
At initial moment (r=0) the force of F=2t=0 it mean no force is acting on the block and the force F increases gradually with time Therefore the friction will come into existence and it will come time existence and it will keep the block in static equlibruim till limiting equilibrium is reached let the come to limiting equilibrium at t=t0 then FBD at that moment will be as shown in fig.
N+2l0sin37∘=mg
For horizontal equilibrium.
μN=2t0cos37∘ .
, Solving equation (i) and (ii)
N=24 Newton and t0=5sec
At t=t0 block is in limiting equilibrium hence just after this moment it will start to slip over the surface threefour at t>t0 the FBD of the block will be as shown in figure for vertical equilibrium
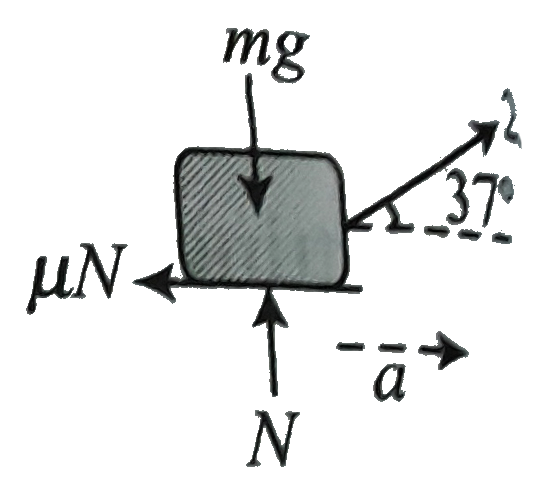
N+2tsin37∘=mg
In horizontal direction it will acceleration
2tcos37∘−μN=ma
From Eqs(iii) and (iv) a=13(2t−10)
, But accfeleration a=dv/dt Therefore
dv=a,d=13(2t−10)dt
Integrating above equation with the condition with the conditions that at t=5sv=0 and at t=10st=?
∫10dv=∫10513(2T−10).dt⇒v=253ms−1
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Single Correct
87 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Multiple Correct
2 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.3
27 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
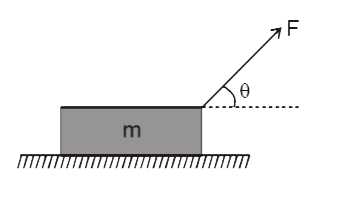
A wooden block of mass m resting on a rough horizontal table (coefficient of friction =μ ) is pulled by a force F as shown in figure.The acceleration of the block moving horizontally is:
View SolutionA block of mass m is placed on a rough horizontal surface. If minimum force to pull the block is mg2 then coefficient of friction is :
View Solution
Knowledge Check
A block of mass m=5kg is resting on a rough horizontal surface for which the coefficient of friction is 0.2 . When a force F=40N is applied, the acceleration of the block will be (g=10m/s2) .
 A5.73m/sec2B8.0m/sec2C3.17m/sec2D10.0m/sec2
A5.73m/sec2B8.0m/sec2C3.17m/sec2D10.0m/sec2A block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
A0.5m/s2B5m/s2C10m/s2D15m/s2A block of mass m is lying on horizontal surface of coefficient of friction μ. A force is applied to the block at an angle θ with the horizontal. The block will move with a minimum force F if
Aμ=tanθBμ=cosθCμ=sinθDμ=cotθ
A block of mass M rests on a rough horizontal surface as shown. Coefficient of friction between the block and the surface is μ. A force F=mg acting at angle θ with the vertical side of the block pulls it. In which of the following cases the block can be pulled along the surface?

View SolutionA block of mass M is at rest on a rough horizontal surface. The coefficient of friction between the block and the surface is μ. A force F = Mg acting at an angle θ with the vertical side of the block pulls it. In which of the following cases, the block can be pulled along the surface?
View SolutionA rectangular block of mass 5 kg is kept on a horizontal surface . The coefficient of friction between the block and the surface is 0.2 . If a force of 20 N is applied to the block at angle of 30∘ with the horizontal plane , what is the force of friction on the block ? (Take g = 10m/s2)
View SolutionA block of mass 2 kg is kept on a rough horizontal floor an pulled with a force F. If the coefficient of friction is 0.5. then the minimum force required to move the block is :-

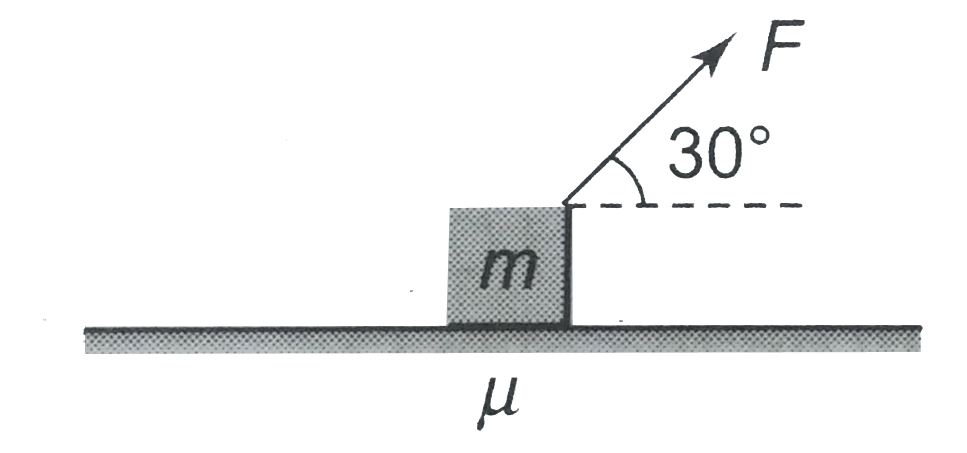
View SolutionA block of mass m lying on a rough horizontal surface of friction coefficient μ is pulled by a force F as shown , the limiting friction between the block and surface will be
View Solution
