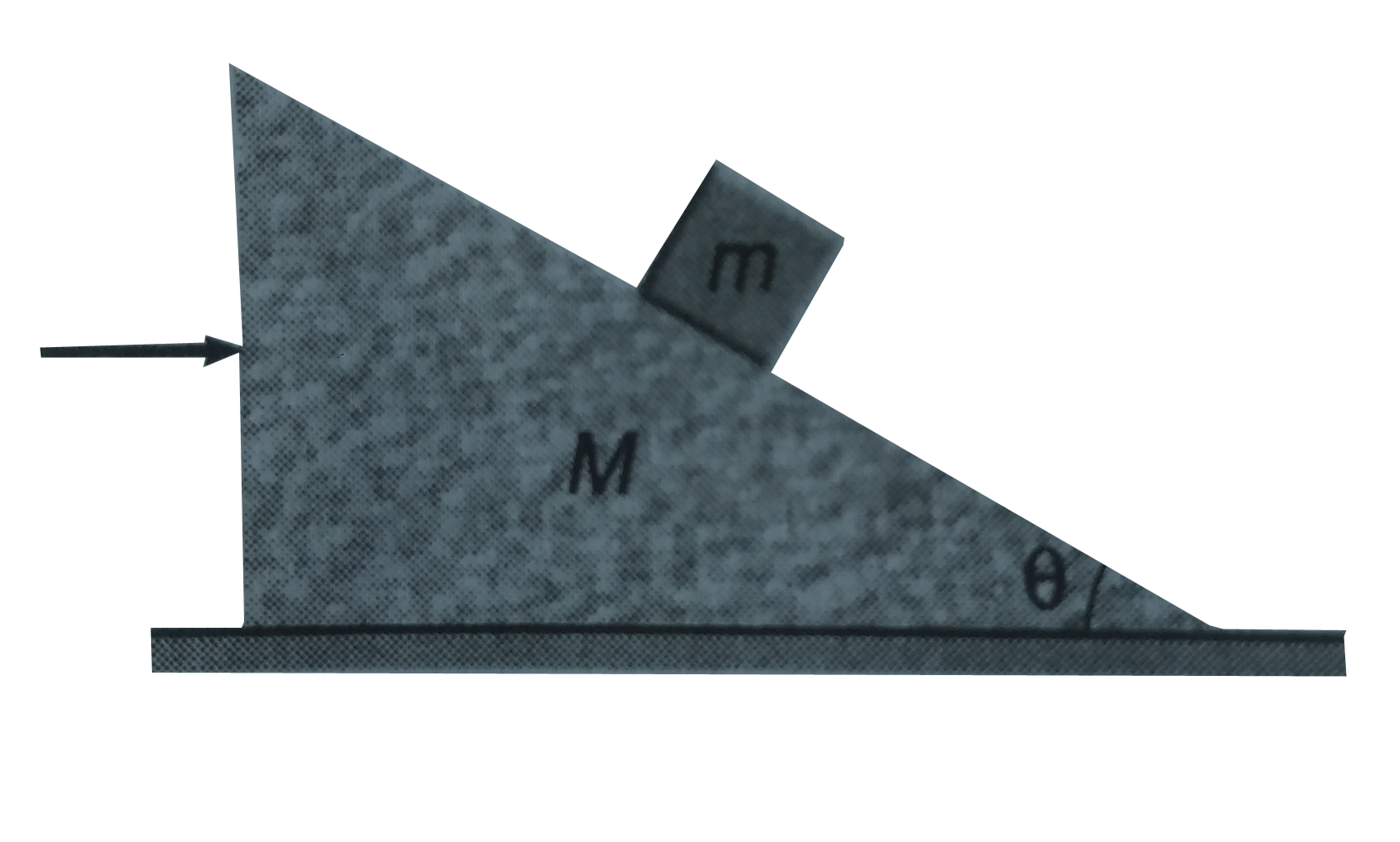
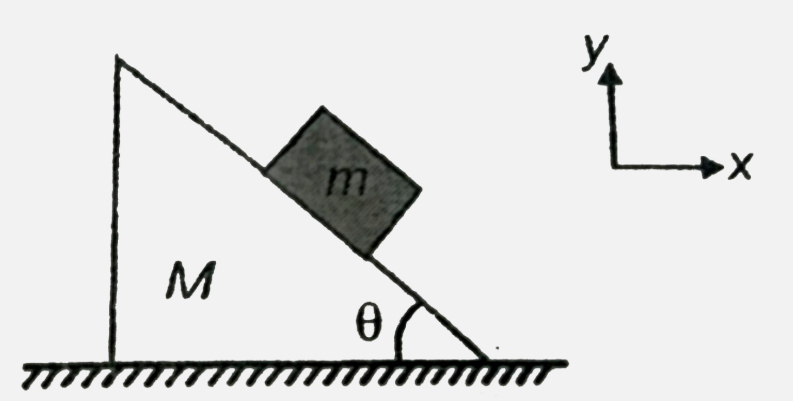
A block of mass m is placed on a smooth inclined wedge ABC of inclination as shown in the figure.

The wedge is given an acceleration a towards the right. The relation between a and e for the block to remain stationary on the wedge is

A

$a = \frac{g}{cos e c θ}$

B

$a = \frac{g}{sin θ}$

C

a=g cos theta

D

$a = g tan θ$

Text Solution

Verified by Experts

The correct Answer is:D

In non-inertial frame ,
$N sin θ = m a$ ….. (I )
$N cos θ = m g \dots \dots \dots (i i)$
from (I ) and (ii)
$tan θ = \frac{a}{g}$
$a = g tan θ$

Updated on:21/07/2023

|

Class 11 PHYSICS NEWTONS LAW OF MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

A block of mass m is placed on a smooth inclined wedge ABC of inclination $θ$ as shown in the figure. The wedge is given an acceleration 'a' towards the right. The relation between a and for the block to remain stationary on the wedge is:

A $a = g cos θ$
B $a = \frac{g}{sin θ}$
C $a = \frac{g}{cosec θ}$
D $a = g tan θ$
A block of mass $m$ is placed on a smooth inclined wedge ABC of inclination theta as shown in the figure. The wedge is given an acceleration $a$ towards the right. The relation between a and theta for the block to remain stationary on the wedge is.

A $a = g tan θ$
B $a = \frac{g}{cos e c θ}$
C $a = cos θ$
D $a = \frac{g}{sin θ}$
A smooth block of mass $m$ is held stationary on a smooth wedge of mass $M$ and inclination $θ$ as shown in figure .If the system is released from rest , then the normal reaction between the block and the wedge is

A $m g cos θ$
Bless than $m g cos θ$
Cgreater than $m g cos θ$
Dmay be less or greater than $m g cos θ$ depending upon whether $M$ is less or greater than $m$

MTG GUIDE-LAWS OF MOTION-AIPMT /NEET (MCQ)

Home
Ask Doubt
Profile