A body A mass m1 exerts a force on another body B of mass m2 . If the acceleration of B be a2 , then the acceleration (in magnitude) of A is
m2m1a2
m1m2a2
m1m2a2
(m1+m2)a2
The correct Answer is:A
To solve the problem, we will apply Newton's third law of motion, which states that for every action, there is an equal and opposite reaction. Let's break down the steps to find the acceleration of body A when body B is accelerating.
1. Identify the Forces:
- Body A (mass m1) exerts a force on body B (mass m2).
- According to Newton's third law, body B exerts an equal and opposite force on body A.
2. Write the Force Equation for Body B:
- The force exerted by body A on body B can be expressed using Newton's second law:
FAB=m2⋅a2
- Here, FAB is the force exerted by A on B, m2 is the mass of body B, and a2 is the acceleration of body B.
3. Write the Force Equation for Body A:
- The force exerted by body B on body A is equal in magnitude and opposite in direction to the force exerted by A on B:
FBA=m1⋅a1
- Here, FBA is the force exerted by B on A, m1 is the mass of body A, and a1 is the acceleration of body A.
4. Set the Forces Equal:
- According to Newton's third law:
FAB=FBA
- Therefore, we can equate the two expressions:
m2⋅a2=m1⋅a1
5. Solve for the Acceleration of Body A:
- Rearranging the equation to find a1:
a1=m2⋅a2m1
Final Answer:
The magnitude of the acceleration of body A is given by:
a1=m2⋅a2m1
- Body A (mass m1) exerts a force on body B (mass m2).
- According to Newton's third law, body B exerts an equal and opposite force on body A.
- The force exerted by body A on body B can be expressed using Newton's second law:
FAB=m2⋅a2
- Here, FAB is the force exerted by A on B, m2 is the mass of body B, and a2 is the acceleration of body B.
- The force exerted by body B on body A is equal in magnitude and opposite in direction to the force exerted by A on B:
FBA=m1⋅a1
- Here, FBA is the force exerted by B on A, m1 is the mass of body A, and a1 is the acceleration of body A.
- According to Newton's third law:
FAB=FBA
- Therefore, we can equate the two expressions:
m2⋅a2=m1⋅a1
- Rearranging the equation to find a1:
a1=m2⋅a2m1
The magnitude of the acceleration of body A is given by:
a1=m2⋅a2m1
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Linear Momentum And Impulse20videos
NEWTONS LAWS OF MOTION
- BOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Equilibrium Of A Particle19videos
NEWTONS LAWS OF MOTION
- BOOK - A2ZCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - Chapter Test29videos
MOTION IN TWO DIMENSION
- BOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Chapter Test29videos
OSCILLATION AND SIMPLE HARMONIC MOTION
Similar Questions
A constant force of 5 N can produce an acceleration of 1 m/s2 on the body of mass m1 and 2m/s2 on the body of mass m2 . If both the masses are tied together and same force is applied then what will be the acceleration of the system ?
View SolutionA constant force of 5N can produce an acceleration of 1 m/s2 on the body of mass m1and2m/s2 on the body of mass m2. If both the masses are tied together and same force is applied, then what will be the acceleration of the system?
View Solution
Knowledge Check
- Question 1 - Select One
A force produces an acceleration of 4m/s2 in a body of m1kg in another body of mass m2kg If the same force is applied to (m1+m2) then the acceleration will be
A10m/s2B2m/s2C2.4m/s2D5.4m/s2 - Question 2 - Select One
A force produces an acceleration of 4ms−2 in a body of mass m1 and the same force produces an acceleration of 6ms−2 in another body oa mass m2. If the same force is applied to (m1+m2), then the acceleration will be
A1.6ma−2B2ms−2C2.4ms−2D3.2ms−2 - Question 3 - Select One
A force F appllied to a body(A)of mas m1 produces an acceleratioin of 4m/s2. If the same force F is applied to another body (B) of mass m2, then an acceleratiionof 10m/s2, produced in the body. A and B are the tied together and the same force is applied to the combined body. What is the acceleration of the system?
A107m/s2B207m/s2C53m/s2D720m/s2
Figure shows a block of mass m1 sliding on a block of mass m2, with m1>m2. Find the
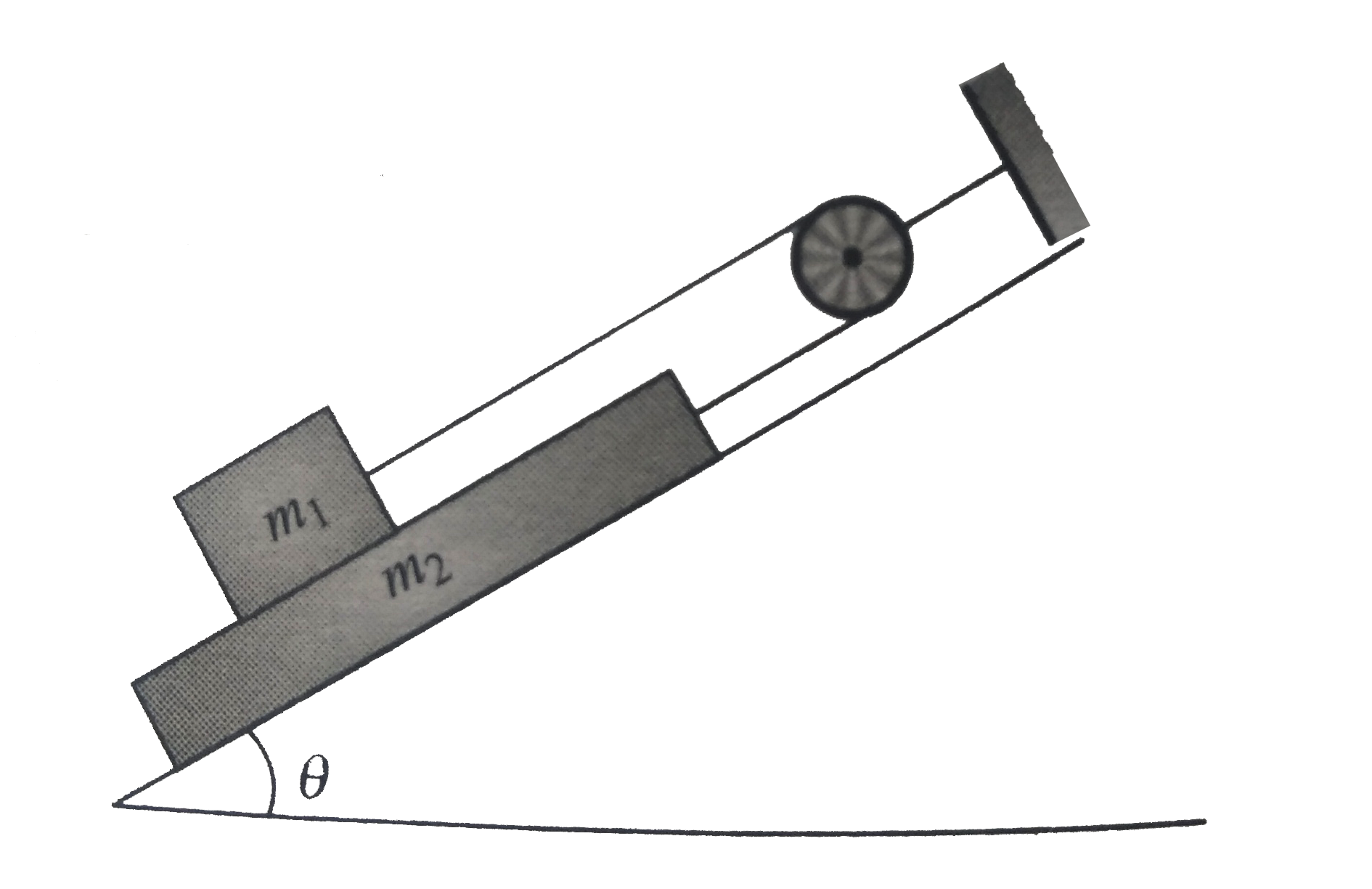
(a) acceleration of each block
(b) tension in the string
(c ) force exerted by m1 on m2. ltbr> (d) force exerted by m2 on the incline.View SolutionA force of 10N gives a mass m1 an acceleration of 10m/s2 and a mass m2 an acceleration of 20m/s2 What acceleration would it give if both the masses are tid together ?
View SolutionTwo bodies of masses 4 kg and 5kg are acted upon by the same force. If the acceleration of lighter body is 2m/sec2, then the acceleration of the heavier body is:-
View SolutionA body of mass m1 collides elastically with another body of mass m2 at rest. If the velocity of m1 after collision is 23 times its initial velocity, the ratio of their masses is :
View SolutionA force of 5N gives a mass m1, an acceleration of m/s2, and a mass m2, an acceleration of 24m/s2. What acceleration would it give if both the masses are tied together?
View Solution

