A body is initially at rest. It undergoes one dimensional motion with constant acceleration. The power delivered to it at time t is proportional to
t1/2
t
t3/2
t2
The correct Answer is:B
To solve the problem, we need to analyze the motion of a body that starts from rest and undergoes one-dimensional motion with constant acceleration. We will derive the expression for power delivered to the body at time t.
1. Identify Initial Conditions:
- The body is initially at rest, so the initial velocity u=0.
2. Use the Equation of Motion:
- The equation for velocity under constant acceleration is given by:
v=u+at
- Substituting u=0:
v=at
3. Define Power:
- Power P is defined as the rate of doing work, which can be expressed as:
P=dWdt
- Where W is the work done.
4. Relate Work Done to Force and Displacement:
- The work done W can also be expressed as:
W=F⋅x
- Where F is the force and x is the displacement.
5. Differentiate Work Done:
- To find power, differentiate the work done with respect to time:
P=dWdt=F⋅dxdt
- Since dxdt=v (velocity), we have:
P=F⋅v
6. Substitute Force and Velocity:
- The force F acting on the body can be expressed using Newton's second law:
F=ma
- Substituting v=at into the power equation:
P=F⋅v=ma⋅(at)=ma2t
7. Analyze the Proportionality:
- In the expression P=ma2t, m (mass) and a (acceleration) are constants. Therefore, the power P is directly proportional to time t:
P∝t
Conclusion:
The power delivered to the body at time t is proportional to t.
- The body is initially at rest, so the initial velocity u=0.
- The equation for velocity under constant acceleration is given by:
v=u+at
- Substituting u=0:
v=at
- Power P is defined as the rate of doing work, which can be expressed as:
P=dWdt
- Where W is the work done.
- The work done W can also be expressed as:
W=F⋅x
- Where F is the force and x is the displacement.
- To find power, differentiate the work done with respect to time:
P=dWdt=F⋅dxdt
- Since dxdt=v (velocity), we have:
P=F⋅v
- The force F acting on the body can be expressed using Newton's second law:
F=ma
- Substituting v=at into the power equation:
P=F⋅v=ma⋅(at)=ma2t
- In the expression P=ma2t, m (mass) and a (acceleration) are constants. Therefore, the power P is directly proportional to time t:
P∝t
The power delivered to the body at time t is proportional to t.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MATCH THE COLUMNS
7 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions
11 Videos
Similar Questions
Projectile motion is a two dimensional motion with constant acceleration. Is this statement true or false?
View SolutionA body initially at rest travels a distance 100 m in 5 s with a constant acceleration. Calculate : (i) the acceleration, and (ii) the final velocity at the end of 5 S.
View Solution
Knowledge Check
For the one dimensional motion, described by x=t−sint
Ax(t)>0 for all t gt 0Bν(t)>0 for all t>0Ca(t)>0 for all t>0Dall of these
A body starts from rest and acquires velocity V in time T. The instantaneous power delivered to the body in time 't' proportional to
View SolutionA body, initially at rest, starts moving with a constant acceleration 2 ms−2. Calculate : (i) the velocity acquired and (ii) the distance travelled in 5 s.
View SolutionA particle starting from rest undergoes a rectilinear motion with acceleration a. The variation of a with time t is shown below. The maximum velocity attained by the particle during its motion is
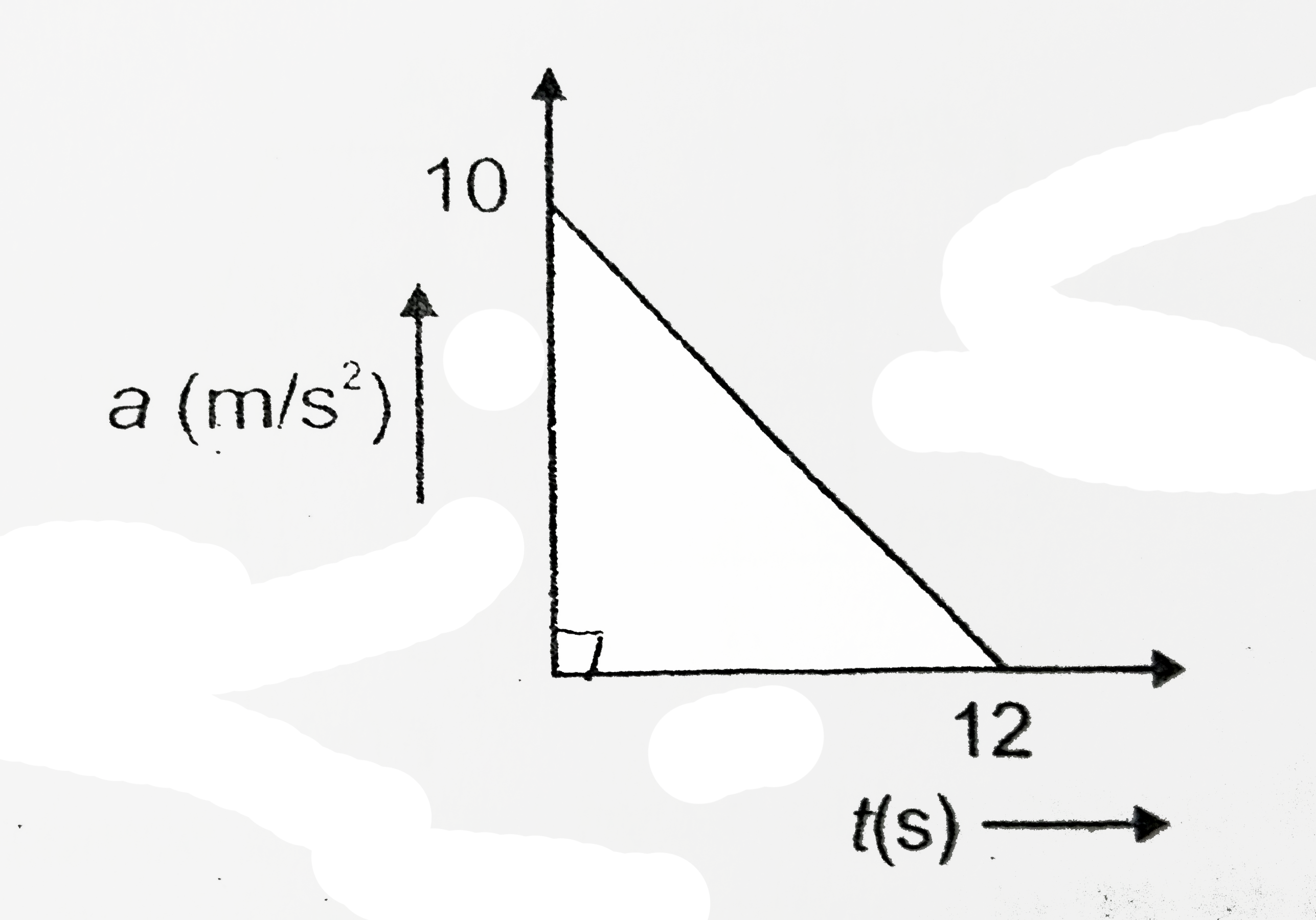
View SolutionAssertion : Simple harmonic motion is an example on one dimensional motion with non-uniform acceleration .
Reason : In simple harmonic motion, acceleration varies with displacement linearly.
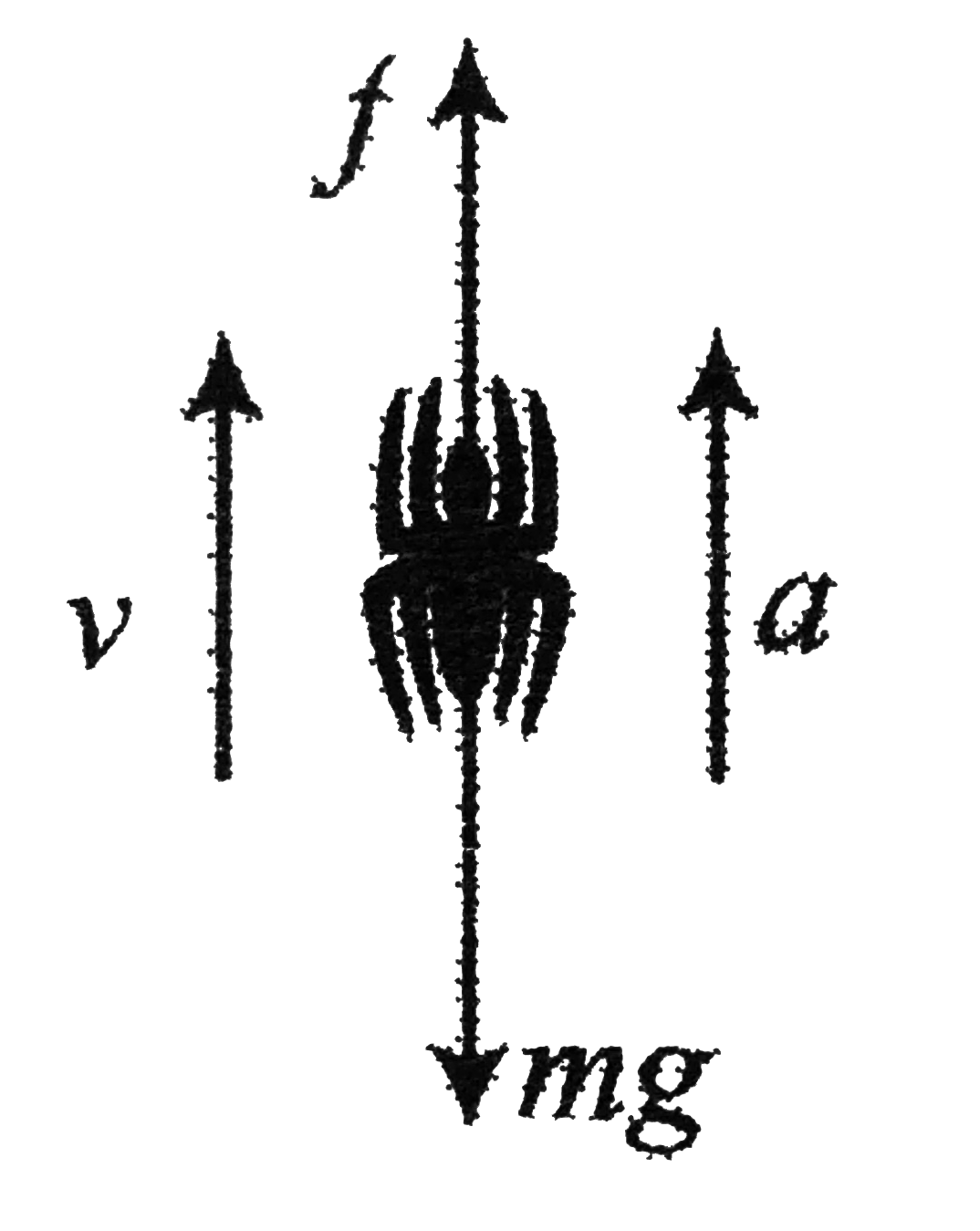
View SolutionAn insect of mass m moves up along a hanging stationary thread, with acceleration a. Find the power delivered by the gravity after a time t.
View SolutionA motor drives a body along a straight line with a constant force. The power P developed by the motor must vary with time t as
View SolutionA variable force F acts on a body which is free to move. The displacement of the body is proportional to t3, where t = time. The power delivered by F to the body will be proportional to
View Solution
