A body of mass m slides down a plane inclined at an angle α. The coefficient of friction is μ. Find the rate at which kinetic plus gravitational potential is dissipated at any time t.
The correct Answer is:A, B, C
To solve the problem of finding the rate at which kinetic plus gravitational potential energy is dissipated when a body of mass m slides down an inclined plane at an angle α with a coefficient of friction μ, we can follow these steps:
Step 1: Identify the Forces Acting on the Body
The forces acting on the body include:
- The gravitational force mg acting vertically downward.
- The normal force N acting perpendicular to the inclined plane.
- The frictional force Ff acting opposite to the direction of motion along the plane.
Step 2: Resolve the Gravitational Force
The gravitational force can be resolved into two components:
- Parallel to the incline: F∥=mgsinα
- Perpendicular to the incline: F_{\perpendicular} = mg \cos \alpha
Step 3: Calculate the Normal Force
The normal force N is equal to the perpendicular component of the gravitational force:
N=mgcosα
Step 4: Calculate the Frictional Force
The frictional force Ff can be expressed as:
Ff=μN=μ(mgcosα)=μmgcosα
Step 5: Write the Net Force Acting on the Body
The net force Fnet acting on the body along the incline is given by:
Fnet=F∥−Ff=mgsinα−μmgcosα
Step 6: Calculate the Acceleration of the Body
Using Newton's second law, we can find the acceleration a:
ma=mgsinα−μmgcosα
a=gsinα−μgcosα
Step 7: Determine the Rate of Work Done by Friction
The power P (rate of work done) due to the frictional force is given by:
P=Ff⋅v
where v is the velocity of the body down the incline.
Step 8: Relate Velocity to Acceleration
Using the kinematic equation, we can express the velocity v in terms of acceleration and time:
v=a⋅t=(gsinα−μgcosα)t
Step 9: Substitute into the Power Equation
Now substituting v into the power equation:
P=μmgcosα⋅v
P=μmgcosα⋅(gsinα−μgcosα)t
Step 10: Final Expression for Power
Thus, the rate at which kinetic plus gravitational potential energy is dissipated is:
P=μmgcosα(gsinα−μgcosα)t
Summary
The rate at which kinetic plus gravitational potential energy is dissipated due to friction while sliding down the incline is given by the expression derived above.
---
The forces acting on the body include:
- The gravitational force mg acting vertically downward.
- The normal force N acting perpendicular to the inclined plane.
- The frictional force Ff acting opposite to the direction of motion along the plane.
The gravitational force can be resolved into two components:
- Parallel to the incline: F∥=mgsinα
- Perpendicular to the incline: F_{\perpendicular} = mg \cos \alpha
The normal force N is equal to the perpendicular component of the gravitational force:
N=mgcosα
The frictional force Ff can be expressed as:
Ff=μN=μ(mgcosα)=μmgcosα
The net force Fnet acting on the body along the incline is given by:
Fnet=F∥−Ff=mgsinα−μmgcosα
Using Newton's second law, we can find the acceleration a:
ma=mgsinα−μmgcosα
a=gsinα−μgcosα
The power P (rate of work done) due to the frictional force is given by:
P=Ff⋅v
where v is the velocity of the body down the incline.
Using the kinematic equation, we can express the velocity v in terms of acceleration and time:
v=a⋅t=(gsinα−μgcosα)t
Now substituting v into the power equation:
P=μmgcosα⋅v
P=μmgcosα⋅(gsinα−μgcosα)t
Thus, the rate at which kinetic plus gravitational potential energy is dissipated is:
P=μmgcosα(gsinα−μgcosα)t
The rate at which kinetic plus gravitational potential energy is dissipated due to friction while sliding down the incline is given by the expression derived above.
Topper's Solved these Questions
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 More Than One Correct
9 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question
11 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
When a body slides down an inclined plane with coefficient of friction as μk, then its acceleration is given by .
View SolutionWhen a body of mass M slides down an inclined plane of inclination θ, having coefficient of friction μ through a distance s, the work done against friction is :
View Solution
Knowledge Check
A block of mass m is at rest on an inclined plane. The coefficient of static friction is μ. The maximum angle of incline before the block begins to slide down is :
Atan−1(μ/m)Btan−1(μ)Ctan−1(μ/2)Dcos−1(μ)
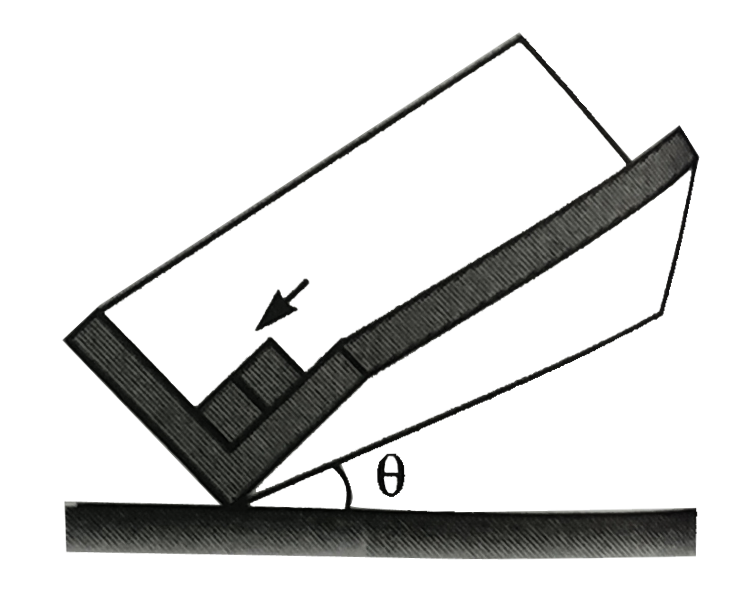
A block of mass m sides down an inclined right angled trough .If the coefficient of friction between block and the trough is μk acceleration of the block down the plane is
View SolutionA block slides down an inclined plane that makes an angle θ with the horizontal. If the coefficient of kinetic friction is μ, find the acceleration of the block.
View SolutionA brick of mass 2kg begins to slide down on a plane inclined at an angle of 45∘ with the horizontal. The force of friction will be
View SolutionA block of mass m is placed on a rough plane inclined at an angle θ with the horizontal. The coefficient of friction between the block and inclined plane is μ . Ifθ<tan−1(μ) , then net contact force exerted by the plane on the block is :
View SolutionA body of mass m is placed on a rough surface with coefficient of friction μ inclined at θ. If the mass is in equilibrium , then
View SolutionA body of mass m is placed on a rough surface with coefficient of friction μ inclined at θ. If the mass is in equilibrium , then
View SolutionA block of mass m slides down an inclined plane which makes an angle θ with the horizontal. The coefficient of friction between the block and the plane is μ. The force exerted by the block on the plane is
View Solution
