A boy throws a ball with initial velocity u at an angle of projection θ from a tower of height H.
Neglecting air resistance, find
a. How high above the building the ball rises
b. Its speed just before it hits the ground.
Step by step video & image solution for A boy throws a ball with initial velocity u at an angle of projection theta from a tower of height H. Neglecting air resistance, find a. How high above the building the ball rises b. Its speed just before it hits the ground. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WORK AND ENERGYBOOK - ANURAG MISHRACHAPTER - WORK AND ENERGYEXERCISE - Level 1
53 Videos
- WORK AND ENERGYBOOK - ANURAG MISHRACHAPTER - WORK AND ENERGYEXERCISE - Level 2
18 Videos
- WORK AND ENERGYBOOK - ANURAG MISHRACHAPTER - WORK AND ENERGYEXERCISE - Matching types problems
14 Videos
- UNIT AND DIMENSIONSBOOK - ANURAG MISHRACHAPTER - UNIT AND DIMENSIONSEXERCISE - Matching Type Problem
4 Videos
Similar Questions
A ball is dropped from a height h above ground,Neglect the air resistance,its velocity (v) varies with its height y above the ground as
View SolutionThree identical balls are thrown from the top of a building, all with the same initial speed. As shown in figure., the first ball is thrown horizontally, second above horizontal level, and third at an angle below the horizontal. Neglecting air resistance, rank the speeds of the balls at the instant each hits the ground.

View Solution
Knowledge Check
A ball is thrown from a point in level with velocity u and at a horizontal distance r from the top of a tower of height h.
At what horizontal distance x from the foot of the tower does the ball hit the ground ? Aucosθg{(u2sin2θ+gh)1/2−usinθ}Bucosθg{(u2cos2θ+2gh)1/2−ucosθ}Cucosθg{(u2cos2θ+gh)1/2−ucosθ}Ducosθg{(u2cos2θ+gh)1/2−ucosθ}
Aucosθg{(u2sin2θ+gh)1/2−usinθ}Bucosθg{(u2cos2θ+2gh)1/2−ucosθ}Cucosθg{(u2cos2θ+gh)1/2−ucosθ}Ducosθg{(u2cos2θ+gh)1/2−ucosθ}A ball is dropped from a height h above ground. Neglect the air resistance, its velocity (v) varies with its height (y) above the ground as :-
A√2g(h−y)B√2ghC√2gyD√2g(h+y)A boy throws a ball with a velocity u at an angle θ with the horizontal. At the same instant he starts running with uniform velocity to catch the ball before it hits the ground. To achieve this he should run with a velocity of
AucosθBusinθCutanθDusecθ
A ball is projected from top of the table with initial speed u at an angle of inclination θ, motion of image of ball w.r.t ball
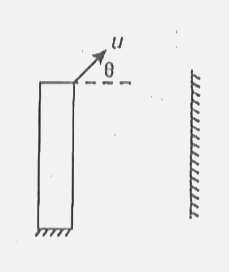
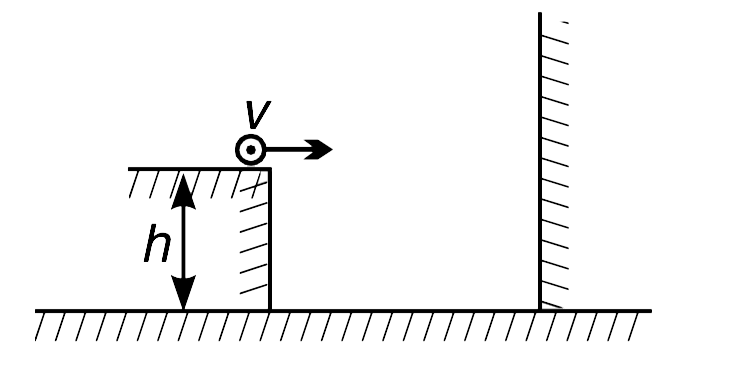
View Solution(a) A particle is thrown from a height h horizontally towards a vertical wall with a speed v as shown in the figure. If the particle returns to the point of projection after suffering two elastic collisions, one with the wall and another with the ground, find the total time of flight. [Elastic collision means the velocity component perpendicular to the surface gets reversed during collision.]
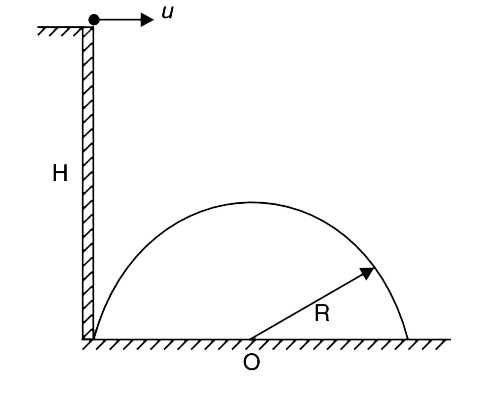
(b) Touching a hemispherical dome of radius R there is a vertical tower of height H=4R. A boy projects a ball horizontally at speed u from the top of the tower. The ball strikes the dome at a height R2 from ground and rebounds. After rebounding the ball retraces back its path into the hands of the body. Find u.
View SolutionA ball is thrown from a point in level with velocity u and at a horizontal distance r from the top os a tower of height h.
How must the speed and angle of the projection of the ball be related to r in order that the ball may just go grazing the top edge of the tower ?
View SolutionA ball is thrown upwards with a speed u from a height h above the ground.The time taken by the ball to hit the ground is
View SolutionA ball is projected with initial velocity u at an angle θ to the horizontal. Then horizontal displacement covered by ball as it collides third time to the ground would be, if coefficient of restitution is e :

View Solution
