A bullet is fired horizontally and gets embedded in a block kept on a table. If table is frictionless, then
kinetic energy is conserved
momentum is conserved
both a and b
none of these
The correct Answer is:C
To solve the problem of a bullet being fired horizontally and embedding itself in a block on a frictionless table, we can follow these steps:
Step 1: Understand the Scenario
A bullet is fired horizontally towards a block resting on a frictionless table. When the bullet embeds itself in the block, they move together as a single system.
Hint: Visualize the scenario by drawing a diagram of the bullet and block on a frictionless table.
Step 2: Identify the Type of Collision
Since the bullet becomes embedded in the block, this is an example of a perfectly inelastic collision. In such collisions, the two objects stick together after the impact.
Hint: Remember that inelastic collisions involve objects sticking together, while elastic collisions do not.
Step 3: Apply the Law of Conservation of Momentum
In the absence of external forces (which is the case here since the table is frictionless), the total momentum before the collision is equal to the total momentum after the collision.
- Let the mass of the bullet be mb and its initial velocity be U.
- Let the mass of the block be mblock and its initial velocity be 0 (since it is at rest).
The momentum before the collision is:
Pinitial=mb⋅U+mblock⋅0=mb⋅U
After the collision, the bullet and block move together with a common velocity V:
Pfinal=(mb+mblock)⋅V
According to the conservation of momentum:
mb⋅U=(mb+mblock)⋅V
Hint: Make sure to set up the momentum equation correctly, considering the masses and velocities before and after the collision.
Step 4: Solve for the Common Velocity V
From the momentum conservation equation, we can solve for V:
V=mb⋅Umb+mblock
Hint: This equation shows how the initial momentum of the bullet is distributed between the bullet and block after the collision.
Step 5: Analyze Kinetic Energy
In a perfectly inelastic collision, kinetic energy is not conserved. Some kinetic energy is transformed into other forms of energy (like heat and sound).
- Initial kinetic energy of the bullet:
KEinitial=12mbU2
- Final kinetic energy after the collision:
KEfinal=12(mb+mblock)V2
Since V is less than U, it follows that:
KEfinal<KEinitial
Hint: Remember that kinetic energy is only conserved in elastic collisions.
Conclusion
- Momentum is conserved in this scenario.
- Kinetic energy is not conserved due to the nature of the collision.
Thus, the correct answer to the question is that momentum is conserved, while kinetic energy is not conserved.
Final Answer: Momentum is conserved; kinetic energy is not conserved.
A bullet is fired horizontally towards a block resting on a frictionless table. When the bullet embeds itself in the block, they move together as a single system.
Since the bullet becomes embedded in the block, this is an example of a perfectly inelastic collision. In such collisions, the two objects stick together after the impact.
In the absence of external forces (which is the case here since the table is frictionless), the total momentum before the collision is equal to the total momentum after the collision.
- Let the mass of the block be mblock and its initial velocity be 0 (since it is at rest).
Pinitial=mb⋅U+mblock⋅0=mb⋅U
Pfinal=(mb+mblock)⋅V
mb⋅U=(mb+mblock)⋅V
From the momentum conservation equation, we can solve for V:
V=mb⋅Umb+mblock
In a perfectly inelastic collision, kinetic energy is not conserved. Some kinetic energy is transformed into other forms of energy (like heat and sound).
KEinitial=12mbU2
KEfinal=12(mb+mblock)V2
KEfinal<KEinitial
- Momentum is conserved in this scenario.
- Kinetic energy is not conserved due to the nature of the collision.
Topper's Solved these Questions
- FORCE AND LAWS OF MOTIONBOOK - MTG IIT JEE FOUNDATIONCHAPTER - FORCE AND LAWS OF MOTIONEXERCISE - EXERCISE (MATCH THE FOLLOWING )
2 Videos
- FORCE AND LAWS OF MOTIONBOOK - MTG IIT JEE FOUNDATIONCHAPTER - FORCE AND LAWS OF MOTIONEXERCISE - EXERCISE (ASSERTION AND REASON )
10 Videos
- FORCE AND LAWS OF MOTIONBOOK - MTG IIT JEE FOUNDATIONCHAPTER - FORCE AND LAWS OF MOTIONEXERCISE - EXERCISE MULTIPLE CHOICE QUESTIONS
1 Videos
- FOOTSTEPS TOWARDS NEETBOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS NEETEXERCISE - Multiple Choice Question
45 Videos
- GRAVITATION BOOK - MTG IIT JEE FOUNDATIONCHAPTER - GRAVITATION EXERCISE - Olympiad/HOTS Corner
20 Videos
Similar Questions
A bullet is fired and gets embedded in a block kept on table. If table is frictionless, then
View SolutionA block of mass m rests on a rough horizontal surface (Coefficient of friction is µ). When a bullet of mass m/2 strikes horizontally, and get embedded in it, the block moves a distance d before coming to rest. The initial velocity of the bullet is k√2μgd, then the value of k is
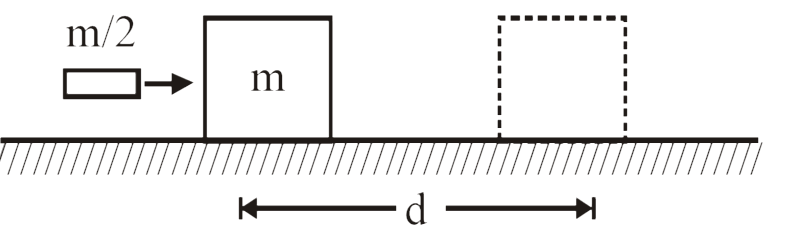
View Solution
Knowledge Check
A bullet in motion hits and gets embedded in a solid block resting on a frictionless table. What is conserved ?
AMomentum and KEBKinetic energy aloneCNeither KE nor momentumDMomentum aloneA bullet hits and gets embedded in a sloid block resting on a horizontal firctionless table . What is conserved ?
Amomentum and KEBmomentumCKEDneither momentum nor KEA bullet hits and gets embedded in a solid block resting on a frictionless surface. In this process, which of the following is correct ?
AOnly momentum is conservedBOnly kinetic energy is conservedCNeither momentum nor kinetic energy is conservedDBoth, momentum and kinetic energy are conserved
Two identical blocks A and B of mass M each are kept on each other on a smooth horizontal plane. There exists friction between A and B. A bullet of mass m hits the the lower block with a horizontal velocity v and goes embedded into it. Find the work done by friction between A and B.
View SolutionA bullet is fired horizontally aiming at an object which starts falling at the instant the bullet is fired. Show that the bullet will hit the object.
View SolutionA large block of wood of mass M=5.99 kg is hanging from two long massless cords. A bullet of mass m=10 g is fired into the block and gets embedded in it. The (block + bullet) then swing upwards, their centre of mass rising a vertical distance -9.8 cm before the (block+bullet) pendulum comes momentarily to rest at the end of its arc. The speed of the bullet just before collision is : (take g=9.8ms−2)

View SolutionA 15gm bullet is fired horizontally into a 3kg block of wood suspended by a string. The bullet sticks in the block, and the impact causes the block to swing 10cm above the initial level. The velocity of the bullet nearly is (in ms−1)
View SolutionAn 8gm bullet is fired horizontally into a 9kg block of wood and sticks in it. The block which is free to move, has a velocity of 40cm/s after impact. The initial velocity of the bullet is
View Solution
