A car travels with a uniform velocity of 20 m s−1 for 5 s. The brakes are then applied and the car is uniformly retarded. It comes to rest in further 8 s. Draw a graph of velocity against time. Use this graph to find :
(i) the distance travelled in first 5 s,
(ii) the distance travelled after the brakes are applied,
(iii) total distance travelled, and
(iv) acceleration during the first 5 s and last 8 s.
To solve the problem step by step, let's break it down according to the parts of the question.
Step 1: Draw the Velocity-Time Graph
1. Draw the axes: The vertical axis represents velocity (in m/s), and the horizontal axis represents time (in seconds).
2. Plot the first segment: From 0 to 5 seconds, the car travels with a uniform velocity of 20 m/s. This is represented as a horizontal line at 20 m/s from t = 0 to t = 5.
3. Plot the second segment: From 5 seconds to 13 seconds (5 + 8 seconds), the car comes to rest uniformly. The velocity decreases from 20 m/s to 0 m/s over 8 seconds. This is represented as a straight line sloping downwards from (5, 20) to (13, 0).
Step 2: Calculate the Distance Travelled in the First 5 Seconds
The distance travelled during the first 5 seconds can be calculated using the area of the rectangle formed in the graph.
- Area of rectangle = length × width
- Length (time) = 5 seconds
- Width (velocity) = 20 m/s
Distance = 5 s × 20 m/s = 100 m
Step 3: Calculate the Distance Travelled After the Brakes Are Applied
The distance travelled after the brakes are applied can be calculated using the area of the triangle formed in the graph.
- Area of triangle = 1/2 × base × height
- Base (time) = 8 seconds (from 5s to 13s)
- Height (initial velocity at B) = 20 m/s
Distance = 1/2 × 8 s × 20 m/s = 80 m
Step 4: Calculate the Total Distance Travelled
The total distance travelled is the sum of the distances from the first segment and the second segment.
Total Distance = Distance in first 5s + Distance after brakes
Total Distance = 100 m + 80 m = 180 m
Step 5: Calculate the Acceleration During the First 5 Seconds
During the first 5 seconds, the car travels with uniform velocity, so there is no change in velocity.
- Acceleration = Change in velocity / Time
- Change in velocity = 0 m/s (since velocity is constant)
- Time = 5 s
Acceleration = 0 m/s / 5 s = 0 m/s²
Step 6: Calculate the Acceleration During the Last 8 Seconds
During the last 8 seconds, the car is uniformly retarded.
- Initial velocity (at B) = 20 m/s
- Final velocity (at D) = 0 m/s
- Change in velocity = Final velocity - Initial velocity = 0 m/s - 20 m/s = -20 m/s
- Time = 8 s
Acceleration = Change in velocity / Time = -20 m/s / 8 s = -2.5 m/s²
Summary of Results
1. Distance travelled in the first 5 seconds: 100 m
2. Distance travelled after the brakes are applied: 80 m
3. Total distance travelled: 180 m
4. Acceleration during the first 5 seconds: 0 m/s²
5. Acceleration during the last 8 seconds: -2.5 m/s²
1. Draw the axes: The vertical axis represents velocity (in m/s), and the horizontal axis represents time (in seconds).
2. Plot the first segment: From 0 to 5 seconds, the car travels with a uniform velocity of 20 m/s. This is represented as a horizontal line at 20 m/s from t = 0 to t = 5.
3. Plot the second segment: From 5 seconds to 13 seconds (5 + 8 seconds), the car comes to rest uniformly. The velocity decreases from 20 m/s to 0 m/s over 8 seconds. This is represented as a straight line sloping downwards from (5, 20) to (13, 0).
The distance travelled during the first 5 seconds can be calculated using the area of the rectangle formed in the graph.
- Length (time) = 5 seconds
- Width (velocity) = 20 m/s
The distance travelled after the brakes are applied can be calculated using the area of the triangle formed in the graph.
- Base (time) = 8 seconds (from 5s to 13s)
- Height (initial velocity at B) = 20 m/s
The total distance travelled is the sum of the distances from the first segment and the second segment.
Total Distance = 100 m + 80 m = 180 m
During the first 5 seconds, the car travels with uniform velocity, so there is no change in velocity.
- Change in velocity = 0 m/s (since velocity is constant)
- Time = 5 s
During the last 8 seconds, the car is uniformly retarded.
- Final velocity (at D) = 0 m/s
- Change in velocity = Final velocity - Initial velocity = 0 m/s - 20 m/s = -20 m/s
- Time = 8 s
1. Distance travelled in the first 5 seconds: 100 m
2. Distance travelled after the brakes are applied: 80 m
3. Total distance travelled: 180 m
4. Acceleration during the first 5 seconds: 0 m/s²
5. Acceleration during the last 8 seconds: -2.5 m/s²
Topper's Solved these Questions
- MOTION IN ONE DIMENSION BOOK - ICSECHAPTER - MOTION IN ONE DIMENSION EXERCISE - EXERCISE -2 (A)
28 Videos
- MOTION IN ONE DIMENSION BOOK - ICSECHAPTER - MOTION IN ONE DIMENSION EXERCISE - EXERCISE -2 (A) ( Multiple choice type: )
5 Videos
- MEASUREMENTS AND EXPERIMENTATION BOOK - ICSECHAPTER - MEASUREMENTS AND EXPERIMENTATION EXERCISE - TOPIC 2 SIMPLE PENDULUM (4 Marks Questions )
1 Videos
- PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSUREBOOK - ICSECHAPTER - PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSUREEXERCISE - Exercise -4(B)Numericals
5 Videos
Similar Questions
A car travels with a uniform velocity of 25 m s−1 for 5 s. The brakes are then applied and the car is uniformly retarded and comes to rest in further 10 s. Find : (i) the distance which the car travels before the brakes are applied, (ii) the retardation,and (iii) the distance travelled by the car after applying the brakes.
View SolutionA train starts from rest and accelerates uniformly at 100 m minute−2 for 10 minutes. Find the velocity acquired by the train. It then maintains a constant velocity for 20 minutes. The brakes are then applied and the train is uniformly retarded. It comes to rest in 5 minutes. Draw a velocity-time graph and use it to find :
(i) the retardation in the last 5 minutes,
(ii) total distance travelled, and
(iii) the average velocity of the train.
View SolutionA car travelling with a velocity of 80 km/h slowed down to 44 km/h in 15 s. The retardation is
View SolutionA car travelling with a velocity of 80 km/h slowed down to 44 km/h in 15 s. The retardation is
View SolutionThe distance travelled by a motor car in t second after the brakes are applied is s feet, where s = 22t −12t2. The distance travelled by the car before it stops, is
View SolutionA car travelling with a velocity of 80 km/h slowed down to 40 km/h in 10s. The retardation is
View SolutionThe speed of a car increases uniformly from zero to 10ms−1 in 2s and then remains constant (figure)
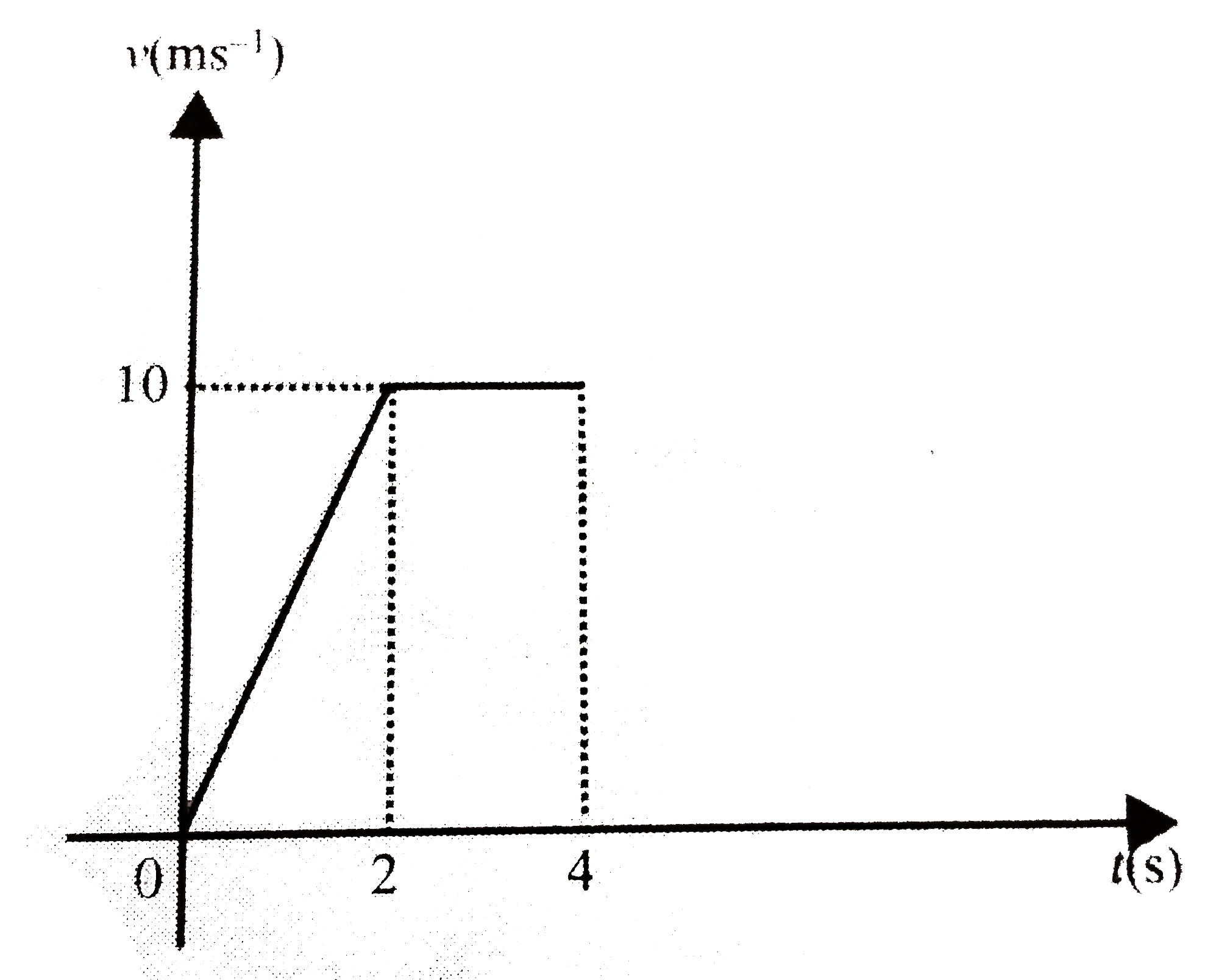
a. Find the distance travelled by the car in the first two seconds.
b. Find the distance travelled by the car in the next two seconds.
c. Find the total distance travelled in 4s.
View SolutionA train starts from rest and accelerates uniformly at a rate of 2 m s−2 for 10 s. It then maintains a constant speed for 200 s. The brakes are then applied and the train is uniformly retarded and comes to rest in 50 s. Find : (i) the maximum velocity reached, (ii) the retardation in the last 50 S, (iii) the total distance travelled, and (iv) the average velocity of the train.
View SolutionA car is moving with a velocity 20m s−1. The brakes are applied to retard it at a rate of 2m s2. What will be the velocity after 5 s of applying the brakes?
View SolutionA car is moving with a velocity 20 m s−1. The brakes are applied to retard it at a rate of 2 m s−2. What will be the velocity after 5 s of applying the brakes ?.
View Solution
