A chain is lying on a smooth table with half its length hanging over the edge of the table [fig(i)]. If the chain is released it slips off the table in time t1. Now, two identical small balls are attached to the two ends of the chain and the system is released [fig(ii)]. This time the chain took t2 time to slip off the table. Which time is larger, t1 or t2?
![A chain is lying on a smooth table with half its length hanging over the edge of the table [fig(i)]. If the chain is released it slips off the table in time t1. Now, two identical small balls are attached to the two ends of the chain and the system is released [fig(ii)]. This time the chain took t(2) time to slip off the table. Which time is larger, t(1) or t(2)?](https://d10lpgp6xz60nq.cloudfront.net/physics_images/IJA_PHY_V01_C03_E01_043_Q01.png)
The correct Answer is:t2
Step by step video, text & image solution for A chain is lying on a smooth table with half its length hanging over the edge of the table [fig(i)]. If the chain is released it slips off the table in time t1. Now, two identical small balls are attached to the two ends of the chain and the system is released [fig(ii)]. This time the chain took t_(2) time to slip off the table. Which time is larger, t_(1) or t_(2)? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

What is the speed of the chain, when it just loses contact with the table?
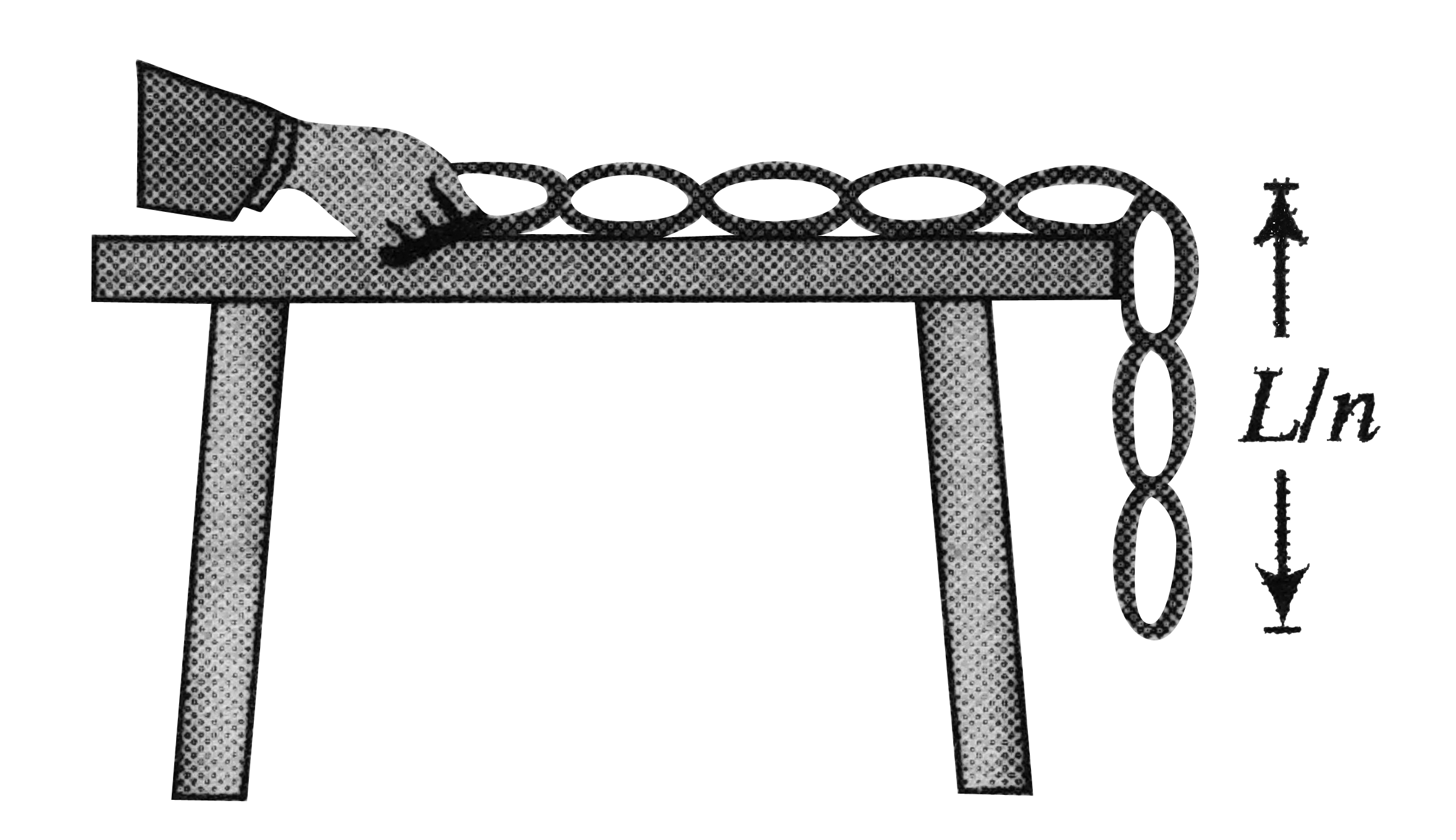
View SolutionA chain of length L and mass M is held on a frictionless table with (1/n)th of its length hanging over the edge. When the chain is released, find the velocity of chain while leaving the table.
View Solution
Knowledge Check
A chain is lying on a rough table with a fraction 1/n of its length hanging down from the edge of the table. if it is just on the point of sliding down from the table, then the coefficient of friction between the table and the chain is -
A1nB1(n−1)C1(n+1)Dn−1(n+1)A chain of mass M is placed on a smooth table with 1/n of its length L hanging over the edge. The work done in pulling the hanging portion of the chain back to the surface of the table is:
AMgL/nBMgL/2nCMgL/n2DMgL/2n2A uniform chain of length l is placed on rough table with length l/n (where n>1) , hanging over the edge. If the chain just begins to slide off the table by itself from this position, the coefficient of friction between the chain and the table is
A1/nB1/(n−1)C1/(n+1)D(n−1)/(n+1)
A uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

If the chain is limiting equlibrium, what is the coefficient of friction for the contact between table and chain?
View SolutionA uniform chain of length l is placed on a rough table, with length l/3 hanging over the edge. If the chain just begins to slide off the table by itself from this portion, the coefficient of friction between the chain and the table is 1/K. Then find the value of K.
View SolutionA chain is held on a frictionless table with 1/n th of its length hanging over the edge. If the chain has a length L and a mass M, how much work is required to pull the hanging part back on the table?
View SolutionA uniform chain of length l and mass m overhangs a smooth table with its tow third pat lying on the tabl. Find the kinetic energy of the chain s it completely slips off the table.
View SolutionA uniform chain of length L lies on a smooth horizontal table with its length perpendicular to the edge of the table and a small portion of the chain is hanging over the edge. The chain starts sliding due to the weight of the hanging part
View Solution
