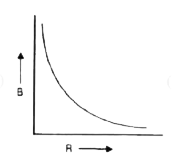
A charge Q is uniformaly distributed over the surface of non-conducting disc of radius R . The disc rotates about an axis perpendicular to its plane and passing through its centre with an angular velocity $ω$ , As a result of this rotation a magnetic field of induction B is obrained at the centre of the disc . If we keep both the amount of charge placed on the disc and its angular velocity to be constant and vary the radius of the disc then the variation fo the magnetic induction at the centre of the disc will be represented by the figure :

ocr_image

Text Solution

Verified by Experts

The correct Answer is:B

Updated on:21/07/2023

A charge $Q$ is uniformly distributed over the surface of non - conducting disc of radius $R$ . The disc rotates about an axis perpendicular to its plane and passing through its centre with an angular to its plane and passing through its centre of the disc. If we keep both the amount of charge placed on the disc and its angular velocity to be constant and vary the radius of the disc then the variation of the magnetic induction at the centre of the disc will br represented by the figure:
A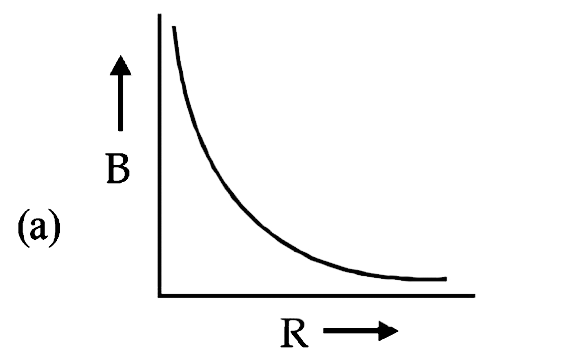
B
C
D
Radius of gyration of disc rotating about an axis perpendicular to its plane passing through through its centre is (If R is the radius of disc)
A $\frac{R}{2}$
B $\frac{R}{\sqrt{2}}$
C $\frac{R}{\sqrt{3}}$
D $\frac{R}{3}$
A non conducting disc of radius $R$ , charge $q$ is rotating about an axis passing through its centre and perpendicular to its plane with an angular velocity $ω$ charge $q$ is uniformly distributed over its surface. The magnetic moment of the disc is
A $\frac{1}{4} q ω R^{2}$
B $\frac{1}{2} q ω R$
C $q ω R$
D $\frac{1}{2} q ω R^{2}$