A charged particle with a velocity 2×103ms−1 passes undeflected through electric field and magnetic fields in mutually perpendicular directions. The magnetic field is 1.5 T. The magnitude of electric field will beSolution in Kannada
1.5×103NC−1
2×103NC−1
3×103NC−1
1.33×103NC−1
The correct Answer is:C
The charged particle goes undeflected through both the fields, therefore, force experienced by charged particle due magnetic field must be equal to the fore experienced by the charge particle due to electric field, i.e, Fm=Fe
or evBsinθ=eE
Given v=2×103ms−1
B=1.5T
and θ=90∘
Hence E=vBsinθ
=2×103×1.5sin90∘
=3×103V/m
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - MULTIPLE CHOICE QUESTIONS (LEVEL-III)9videos
ELECTRONS & PHOTONS
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - RECENT COMPETITIVE QUESTIONS4videos
ELECTROMAGNETIC WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS39videos
ELECTROSTATICS
Similar Questions
Energy required for the electron excitation in Li++ from the first to the third Bohr orbit is :
03:48View SolutionThe speed of electromagnetic wave in vacuum depends upon the source of radiation
02:57View SolutionTwo small spheres of masses M1andM2 are suspended by weightless insulating threads of lengths L1andL2 The spheres carry charges Q1andq2 respectively . The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of θ1andθ2 as shown . Which one o fthe following conditions , if θ1=θ2 ?
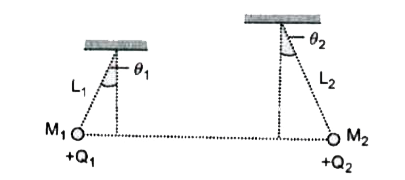 01:11View Solution
01:11View SolutionA particle of charge q and velocity v passes undeflected through a space with non-zero electric field E and magnetic field B. The undeflecting conditions will hold, if
02:49View SolutionA charged particle with a velocity 2xx10^(3) ms^(-1) passes undeflected through electric field and magnetic fields in mutually perpendicular directions. The magnetic field is 1.5 t. the magnitude of electric field will be
03:11View SolutionIn a velocity selector , the intensity of the electric field is 3 xx 10^(4) V/m and the strength of the magnetic field is 0.015 Wb//m^2 . The speed of the charged particles which pass undeflected through the velocity selector is
04:04View SolutionA charged particle moving with velocity vis subjected to electric field E and magnetic field B. The particle will go undeflected if
02:53View SolutionAn the electron undeflected when passing perpen to mutually perpendicular electric and magnetic fields magnetic field is 8 Gauss and the electric field Vm^(-1) the velocity of the electron is
04:52View SolutionA beam of electron posses undeflected through mutually perpendicular electric and magnetic fields. If the electric field is switched off and the same magnetic field is maintained, the electrons move,
05:20View SolutionA charged particle with a velocity 2 xx 10^(3) m s^(-1) passes undeflected through electric field and magnetic fields in the mutually perpendicular directions. The magnetic field is 1.5 T. The magnitude of electric field will be
05:19View SolutionA beam of electron passes undeflected through mutually perpendicular electric and magnetic fields. If the electric field is switched off, and the same magnetic field is maintained, the electrons move
03:37View SolutionA charged particle moving with velocity vis subjected to electric field E and magnetic field B. The particle will go undeflected if
02:04View SolutionA particle of charge q and velocity v passes undeflected through a space with non-zero electric field E and magnetic field B. The undeflecting conditions will hold, if
03:13View SolutionA charged particle with a velocity 2xx10^(3) ms^(-1) passes undeflected through electric field and magnetic fields in mutually perpendicular directions. The magnetic field is 1.5 T. the magnitude of electric field will be
View SolutionA charged particle with a velocity 2×103ms−1 passes undeflected through electric field and magnetic fields in mutually perpendicular directions. The magnetic field is 1.5 T. The magnitude of electric field will be
01:34View Solution

