λ1 and λ2 are used to illuminated the slits. β1 and β2 are the corresponding fringe widths. The wavelength λ1 can produce photoelectric effect when incident on a metal. But the wavelength λ2 cannot produce photoelectric effect. The correct relation between β1 and β2 isSolution in Kannada
β1<β2
β1=β2
β1>β2
β1≠β2
The correct Answer is:A
Fring width β∝λ. AS given that λ1 produced photo electric effect, so λ1<λ2
Hence, β1<β2
Topper's Solved these Questions
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - MULTIPLE CHOICE QUESTIONS (LEVEL-III)
9 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - RECENT COMPETITIVE QUESTIONS
4 Videos
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS
39 Videos
Similar Questions
Energy required for the electron excitation in Li++ from the first to the third Bohr orbit is :
View SolutionThe speed of electromagnetic wave in vacuum depends upon the source of radiation
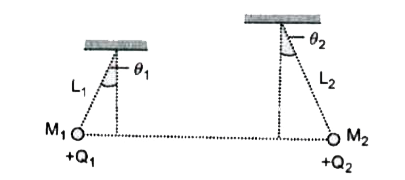
View SolutionTwo small spheres of masses M1andM2 are suspended by weightless insulating threads of lengths L1andL2 The spheres carry charges Q1andq2 respectively . The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of θ1andθ2 as shown . Which one o fthe following conditions , if θ1=θ2 ?
View SolutionA light source, which emits two wavelength λ1=400nm and λ2=600nm, is used in a Young's double slit experiment. If recorded fringe width for λ1 and λ2 are β1 and β2 and the number of fringes for them within a distance y on one side of the central maximum are m1 and m2 respectively, then
View SolutionIn the fraunhaufer differaction from a single slit illuminated by polychromatic light, the first minimum with wavelength lamda_(1) is found to be coincident with the third minimum at lamda_(2) . Then find the value of (lamda_(1))/(lamda_(2))
View SolutionA dye absorbs a photon of wavelength lamda and re-emits the same energy into two photns of wavelength lamda_(1) and lamda_(2) respectively. The wavelength lamda is related to lamda_(1) and lamda_(2) as :
View Solutionlambda_(1) and lambda_(2) are used to illuminated the slits. beta_(1) and beta_(2) are the corresponding fringe widths. The wavelength lambda_(1) can produce photoelectric effect when incident on a metal But the wavelength lambda_(2) cannot produce photoelectric effect. The correct relation between beta_(1) and beta_(2) is
View SolutionThe matrix A=⎡⎢ ⎢⎣λ21λ1λ2λ1λ3λ2λ1λ22λ2λ3λ3λ1λ3λ2λ23⎤⎥ ⎥⎦ is idempotent if λ21+λ22+λ23=k where λ1,λ2,λ3 are non-zero real numbers. Then the value of (10+k)2 is . . .
View SolutionIn a single slit diffraction pattern, the first minima for a wavelength λ1=6000 Å coincides with the first maxima for a wavelength λ2. Then the value of λ2 is
View SolutionIf λ1andλ2 are the wavelengths of the first members of the Lyman and Paschen series respectively, then λ1:λ2 is
View SolutionFor Balmer series, wavelength of first line is lamda_(1) and for Brackett series, wavelength of first line is lamda_(2) then (lamda_(1))/(lamda_(2))
View Solutionlamda_(1) and lamda_(2) are used to illuminated the slits. beta_(1) and beta_(2) are the corresponding fringe widths. The wavelength lamda_(1) can produce photoelectric effect when incident on a metal. But the wavelength lamda_(2) cannot produce photoelectric effect. The correct relation between beta_(1) and beta_(2) is
View SolutionTwo wavelengths λ1 and λ2 are used in Young's double slit expt. λ1=430nm. What is the value of λ2 if 4th bright band of one coincides with 6th bright band of the other?
View SolutionLet α=3i+j and β=2i−j+3k. If β=β1−β2, where β1 is parallel to α and β2 is perpendicular to α, then β1×β2 is equal to
View Solutionlambda_(1) and lambda_(2) are used to illuminated the slits. beta_(1) and beta_(2) are the corresponding fringe widths. The wavelength lambda_(1) can produce photoelectric effect when incident on a metal But the wavelength lambda_(2) cannot produce photoelectric effect. The correct relation between beta_(1) and beta_(2) is
View Solution
