A charged particle would continue to move with a constant velocity in a region wherein,
E=0,B≠0
E≠0,B≠0
E≠0,B=0
E=0,B=0
The correct Answer is:A, B, D
To determine the conditions under which a charged particle continues to move with a constant velocity, we need to analyze the forces acting on the particle in different scenarios involving electric (E) and magnetic (B) fields.
1. Understanding Constant Velocity:
A charged particle moves with constant velocity when the net force acting on it is zero. According to Newton's first law of motion, if no net force acts on an object, it will maintain its state of motion.
2. Analyzing the First Option (E = 0, B ≠ 0):
- If the electric field (E) is zero, the electric force (F_e = qE) is also zero.
- The magnetic force (F_m = qvBsinθ) depends on the velocity (v) and the magnetic field (B). If θ = 0 (the angle between velocity and magnetic field), then sin(0) = 0, resulting in F_m = 0.
- Since both forces are zero, the net force is zero, and the particle continues to move with constant velocity.
- Conclusion: This option is valid.
3. Analyzing the Second Option (E ≠ 0, B ≠ 0):
- Here, both electric and magnetic fields are present, leading to non-zero forces.
- The electric force is F_e = qE, and the magnetic force is F_m = qvBsinθ.
- For the particle to move with constant velocity, these forces must balance each other: F_e = F_m. This can happen if v = E/B, which is a condition known as a velocity selector.
- Conclusion: This option is also valid.
4. Analyzing the Third Option (E ≠ 0, B = 0):
- With B = 0, the magnetic force F_m = 0.
- However, since E ≠ 0, the electric force F_e = qE is non-zero.
- A non-zero force implies that the particle will experience an acceleration, changing its velocity.
- Conclusion: This option is not valid.
5. Analyzing the Fourth Option (E = 0, B = 0):
- In this scenario, both electric and magnetic forces are zero (F_e = 0 and F_m = 0).
- With no net force acting on the particle, it will maintain its constant velocity.
- Conclusion: This option is valid.
Final Answer:
The charged particle would continue to move with a constant velocity in regions described by options 1, 2, and 4 (E = 0, B ≠ 0; E ≠ 0, B ≠ 0; E = 0, B = 0).
---
A charged particle moves with constant velocity when the net force acting on it is zero. According to Newton's first law of motion, if no net force acts on an object, it will maintain its state of motion.
- If the electric field (E) is zero, the electric force (F_e = qE) is also zero.
- The magnetic force (F_m = qvBsinθ) depends on the velocity (v) and the magnetic field (B). If θ = 0 (the angle between velocity and magnetic field), then sin(0) = 0, resulting in F_m = 0.
- Since both forces are zero, the net force is zero, and the particle continues to move with constant velocity.
- Conclusion: This option is valid.
- Here, both electric and magnetic fields are present, leading to non-zero forces.
- The electric force is F_e = qE, and the magnetic force is F_m = qvBsinθ.
- For the particle to move with constant velocity, these forces must balance each other: F_e = F_m. This can happen if v = E/B, which is a condition known as a velocity selector.
- Conclusion: This option is also valid.
- With B = 0, the magnetic force F_m = 0.
- However, since E ≠ 0, the electric force F_e = qE is non-zero.
- A non-zero force implies that the particle will experience an acceleration, changing its velocity.
- Conclusion: This option is not valid.
- In this scenario, both electric and magnetic forces are zero (F_e = 0 and F_m = 0).
- With no net force acting on the particle, it will maintain its constant velocity.
- Conclusion: This option is valid.
The charged particle would continue to move with a constant velocity in regions described by options 1, 2, and 4 (E = 0, B ≠ 0; E ≠ 0, B ≠ 0; E = 0, B = 0).
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Comphension Based
2 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Subjective type
3 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Subjective
11 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Concept Based
8 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False
3 Videos
Similar Questions
A charged particle moving along +ve x-direction with a velocity v enters a region where there is a uniform magnetic field B0(−ˆk),omx=0→x=d. The particle gets deflected at an angle θ from its initial path. The specific charge of the particle is

View SolutionA particle moves with constant angular velocity in a circle. During the motion its
View Solution
Knowledge Check
A particle moves in a plane with a constant acceleration in a direction different from the initial velocity. The path of the particle is
Astraight lineBarc of circleCparabolaDellipseA charged particle is moving on circular path with velocityv in a uniform magnetic field B, if the velocity of the charged particle is doubled and strength of magnetic field is halved, then radius becomes
A8 timesB4 timesC2 timesD16 times
A force ¯¯¯F=¯¯¯Vׯ¯¯A is exerted on a particle in addition to the force of gravity, where ¯¯¯V is the velocity of the particle and ¯¯¯A is a constant vector in the horizontal direction. The minimum speed of a particle of mass 3kg be projected, so that it continues to move undeflected with a constant velocity is xgA. Then 'x' is (consider the plane of the ground as xy plane)
View SolutionAssertion: In successive time intervals if the average velocities of a particle are equal then the particle must be moving with constant velocity.
Reason: When a particle moves with uniform velocity, its displacement always increases with time.
View SolutionShow that the angular momentum about any point of a single particle moving with constant velocity remains constant throughout the motion.
View SolutionTo keep a particle moving with constant velocity on a frictionless horizontal surface an external force
View SolutionAssertion : If a particle moves with a constant velocity, then angular momentum of this particle about any point remains constant.
Reason : Angular momentum has the units of Plank's constant.
View SolutionA particle having charge of 20μC and mass 20μg moves along as circle of radius 5cm under the action of a magnetif field B=0.1 tesla. When the particle is at P, uniform transverse electric field is switched on an it is found that the particle continues along the tangent with a uniform velocity. Find the electric field
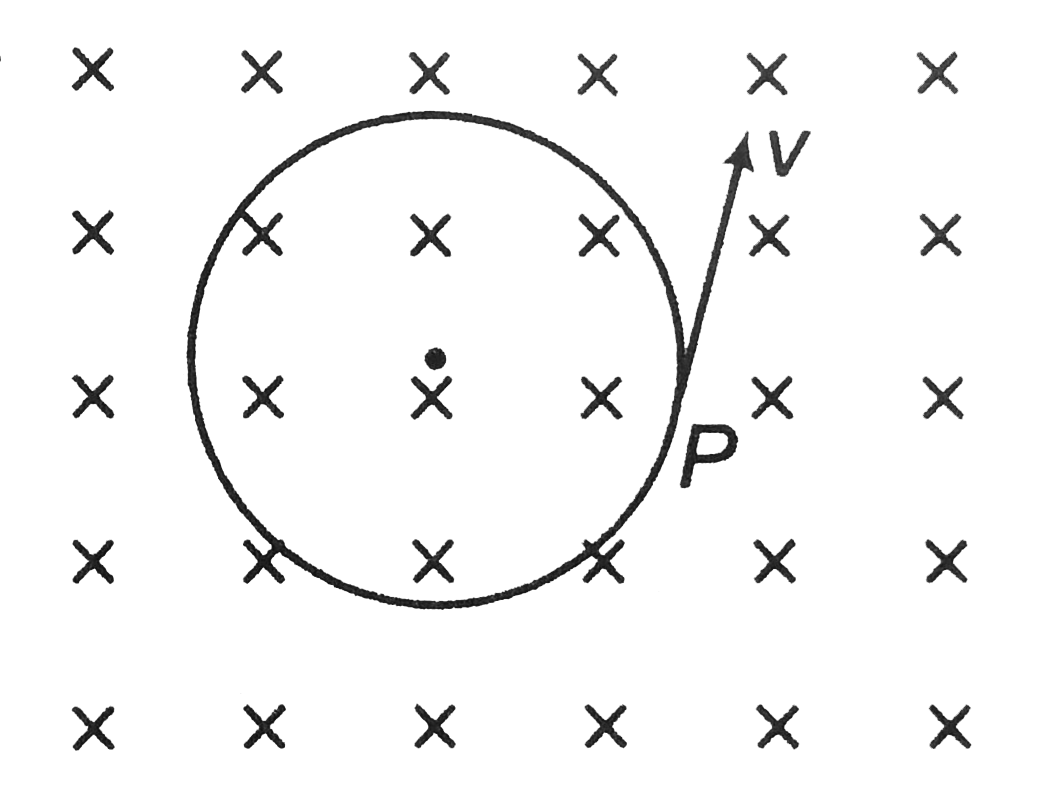
View Solution
