A force ¯¯¯F=¯¯¯Vׯ¯¯A is exerted on a particle in addition to the force of gravity, where ¯¯¯V is the velocity of the particle and ¯¯¯A is a constant vector in the horizontal direction. The minimum speed of a particle of mass 3kg be projected, so that it continues to move undeflected with a constant velocity is xgA. Then 'x' is (consider the plane of the ground as xy plane)
The correct Answer is:3
To solve the problem, we need to analyze the forces acting on the particle and derive the minimum speed required for it to move undeflected with constant velocity.
1. Identify the Forces Acting on the Particle:
- The gravitational force acting downward on the particle is given by ¯Fg=m¯g, where m=3kg is the mass of the particle and ¯g is the acceleration due to gravity.
- An additional force ¯F=¯VׯA is acting on the particle, where ¯V is the velocity of the particle and ¯A is a constant vector in the horizontal direction.
2. Set Up the Equation for Constant Velocity:
- For the particle to move with constant velocity, the net force acting on it must be zero. This means that the upward component of the force ¯F must balance the downward gravitational force ¯Fg.
- The upward component of the force can be expressed as Fup=|¯V||¯A|sinθ, where θ is the angle between the velocity vector ¯V and the vector ¯A.
3. Equate the Forces:
- Since the net force is zero, we can write:
|¯V||¯A|sinθ=mg
- Rearranging gives us:
|¯V|=mg|¯A|sinθ
4. Determine Minimum Speed:
- To find the minimum speed |¯V|, we need to maximize sinθ. The maximum value of sinθ is 1 (when θ=90∘).
- Thus, the minimum speed becomes:
|¯V|min=mg|¯A|
5. Substitute Known Values:
- Given m=3kg and substituting g (approximately 9.81m/s2):
|¯V|min=3×9.81|¯A|=29.43|¯A|
6. Compare with Given Expression:
- The problem states that the minimum speed is given by xgA.
- We can equate this with our derived expression:
29.43|¯A|=xgA
- Since g is a constant, we can compare coefficients:
x=3
Final Answer:
Thus, the value of x is 3.
---
- The gravitational force acting downward on the particle is given by ¯Fg=m¯g, where m=3kg is the mass of the particle and ¯g is the acceleration due to gravity.
- An additional force ¯F=¯VׯA is acting on the particle, where ¯V is the velocity of the particle and ¯A is a constant vector in the horizontal direction.
- For the particle to move with constant velocity, the net force acting on it must be zero. This means that the upward component of the force ¯F must balance the downward gravitational force ¯Fg.
- The upward component of the force can be expressed as Fup=|¯V||¯A|sinθ, where θ is the angle between the velocity vector ¯V and the vector ¯A.
- Since the net force is zero, we can write:
|¯V||¯A|sinθ=mg
- Rearranging gives us:
|¯V|=mg|¯A|sinθ
- To find the minimum speed |¯V|, we need to maximize sinθ. The maximum value of sinθ is 1 (when θ=90∘).
- Thus, the minimum speed becomes:
|¯V|min=mg|¯A|
- Given m=3kg and substituting g (approximately 9.81m/s2):
|¯V|min=3×9.81|¯A|=29.43|¯A|
- The problem states that the minimum speed is given by xgA.
- We can equate this with our derived expression:
29.43|¯A|=xgA
- Since g is a constant, we can compare coefficients:
x=3
Thus, the value of x is 3.
Similar Questions
Knowledge Check
A particle moves in a plane with a constant acceleration in a direction different from the initial velocity. The path of the particle is
Astraight lineBarc of circleCparabolaDellipse
Force acting on a particle of mass m moving in straight line varies with the velocity of the particle as F=K/V K is constant then speed of the particle in time t
View SolutionVelocity of a particle is in negative direction with constant acceleration in positive direction. Then match the following:

View SolutionForces acting on a particle moving in a straight line varies with the velocity of the particle as F=αυ where α is constant. The work done by this force in time interval Δt is :
View SolutionA charged particle enters into a space and continues to move undeflected. Considering gravitational force also on the particle, in the space
View SolutionA particle of mass m initially moving with speed v.A force acts on the particle f=kx where x is the distance travelled by the particle and k is constant. Find the speed of the particle when the work done by the force equals W.
View SolutionA particle of mass m initially at rest. A variabl force acts on the particle f=kx2 where k is a constant and x is the displacment. Find the work done by the force f when the speed of particles is v.
View SolutionA particle of mass 5kg is moving on rough fixed inclined plane with constant velocity of 5m/s as shown in the figure. Find the friction force acting on a body by plane.
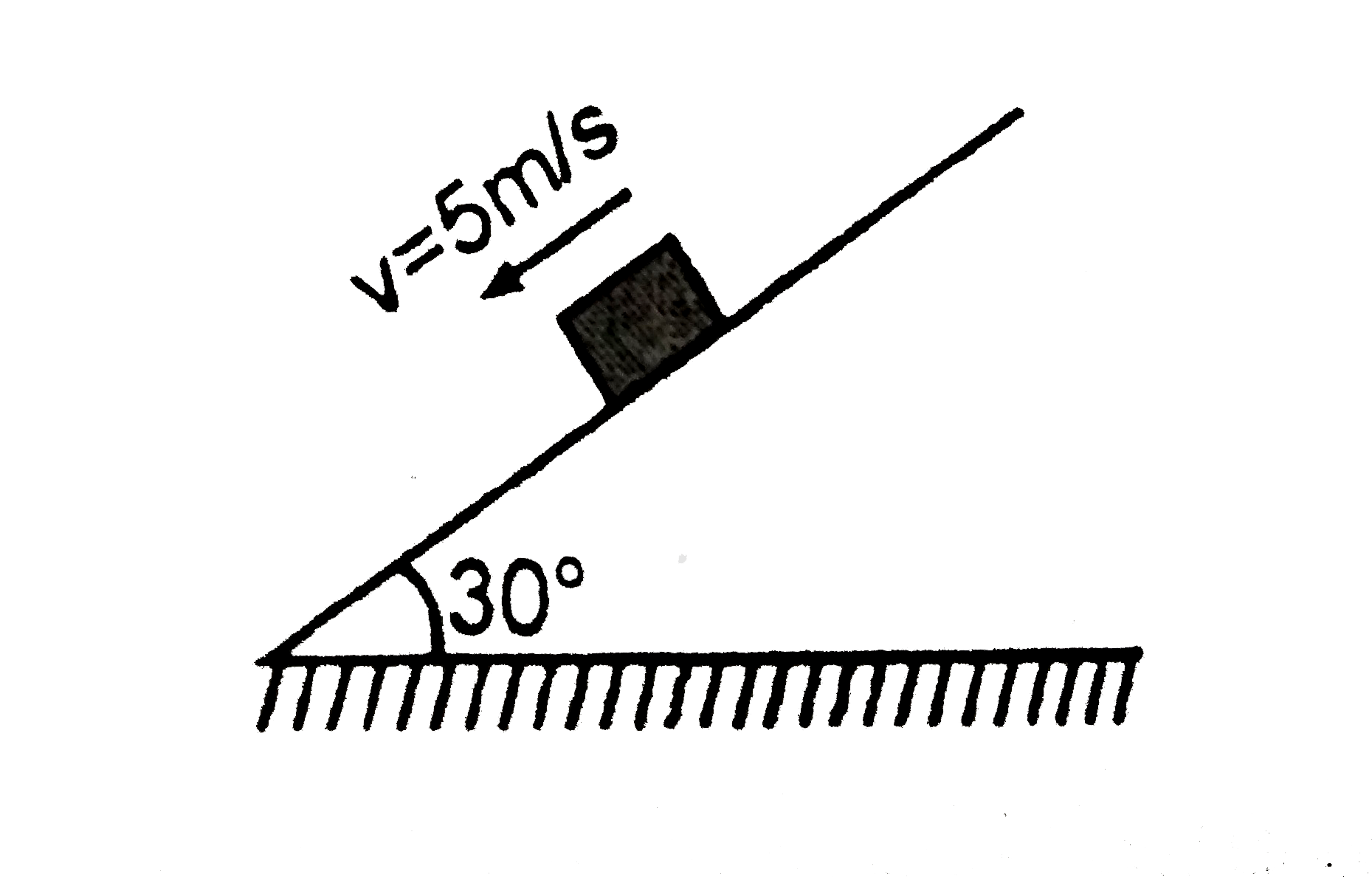
View Solution
