A circular copper ring of radius r translates in its plane with a constant velocity v. A uniform magnetic field b exists in the space in a direction perpendicular ot the plane of the ring. Consider different pairs of diametrically opposite points on the ring. (a) Between which pair of points is the emf? (b) Between which pair of points is the emf minimum? What is the value of this minimum emf?
(a) The emf is highest between the diameter perpendicular to the velocity because here length perpendicular to velocity is highest.E_(max)-vB 2R(b) The length perpendicular to velocity is lowest as the diameter is parallel to the velocity E=_(min)=0.
Topper's Solved these Questions
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE9videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective 210videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Exercises75videos
ELECTRIC FIELD AND POTENTIAL
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercises9videos
ELECTROMAGNETIC WAVES
Similar Questions
A circular copper ring of radius r translates in its plane with a constant velocity v. A uniform magnetic field b exists in the space in a direction perpendicular ot the plane of the ring. Consider different pairs of diametrically opposite points on the ring. (a) Between which pair of points is the emf? (b) Between which pair of points is the emf minimum? What is the value of this minimum emf?
View SolutionA righ of radius 2m translates in its plane with a constant velocity 5m/s. A uniform magnetic field 0.1 T exists in the space in a direction perpendicualr to the plane of the ring. Consider different pairs of diametrically opposite points on the ring.
(i) Between which pair of points is emf maximum? What is the value of this maximum emf?
(ii) Between which pair of points is the emf maximum? What is the value of this minimum emf?View Solution
Knowledge Check
- Question 1 - Select One
A ring of radius R is rolling on a horizontal plane with constant velocity v. There is a constant and uniform magnetic field B which is perpendicular to the plane of the ring. Emf across the lowest point A and right-most point C as shown in the figure will
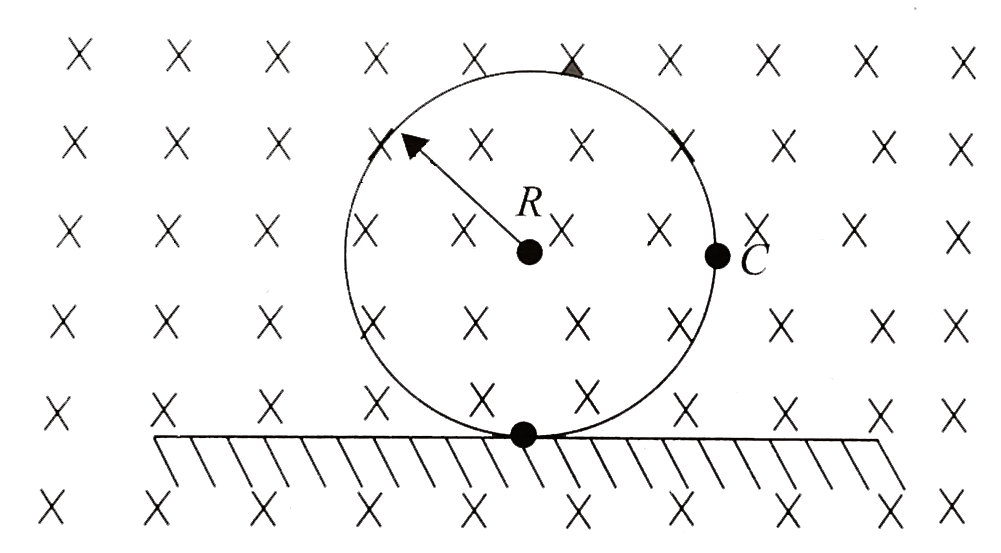 AIncrease with timeBdecrease with timeCremains constant and equal to √2BvRDremains constant and equal to BvR
AIncrease with timeBdecrease with timeCremains constant and equal to √2BvRDremains constant and equal to BvR - Question 2 - Select One
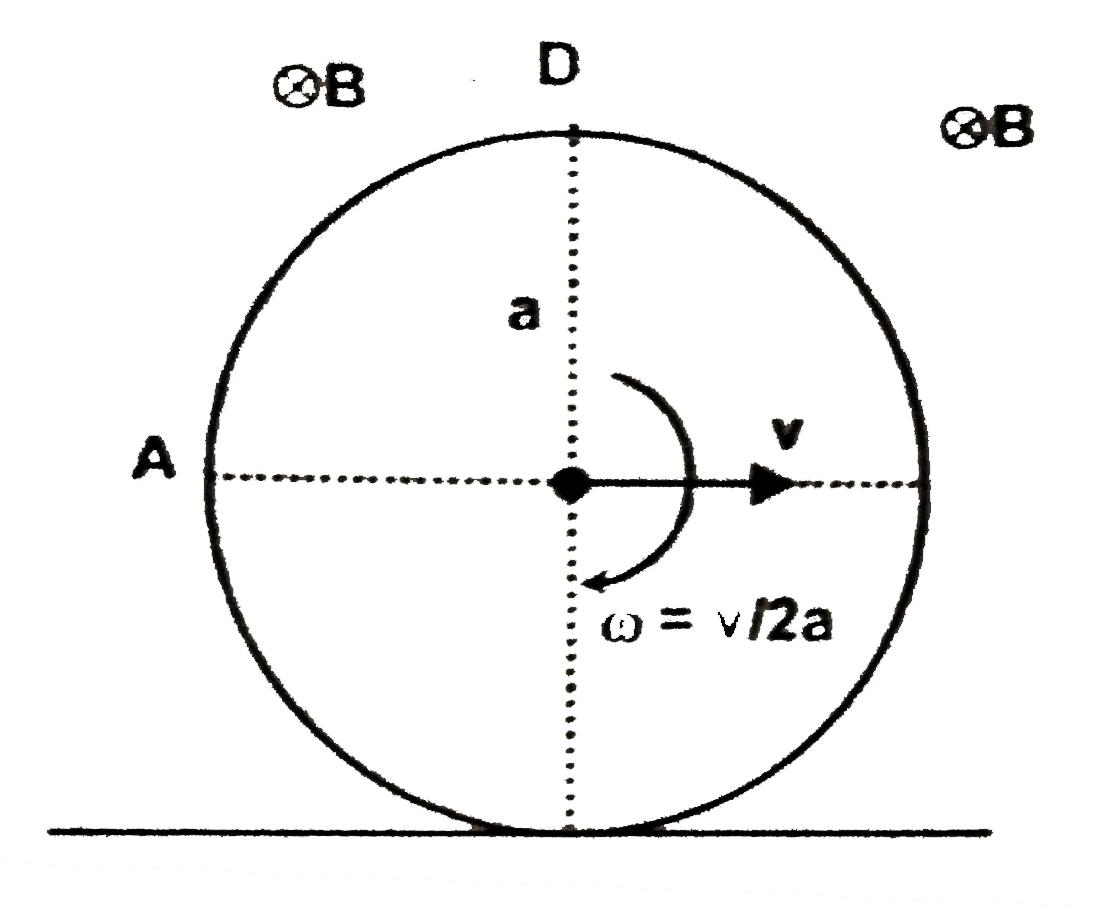
A circular conducting ring of radius 'a' is rolling with slipping on a horizontal surface as shown. A uniform magnetic field B is existing perpendicular to the plane of motion of the ring. The emf iniduced between the points A and D of the ring isABωa2B2Bωa2C4Bωa2D6Bωa2 - Question 3 - Select One or More
A conducting ring is placed in a uniform magnetic field with its plane perpendicular to the field . An emf is induced in the ring if
AIt is rotated about it axisBit is translatedCit is rotated about a diameterDit is deformed
A copper wire bent in the shape of a semicircle of radius r translates in its plane with a constant velocity v. A uniform magnetic field B exists in the direction perpendicular to the plane of the wire. Find the emf induced between the ends of the wire if (a) the velocity is perpendicular ot the diameter joining free ends, (b) the velocity is parallel to this diameter.
View SolutionA conducting ring of radius r and resistance R rolls on a horizintal surface with constant velocity v. The magnetic field B is uniform and is normal to the plane of the loop. Choose the correct option.
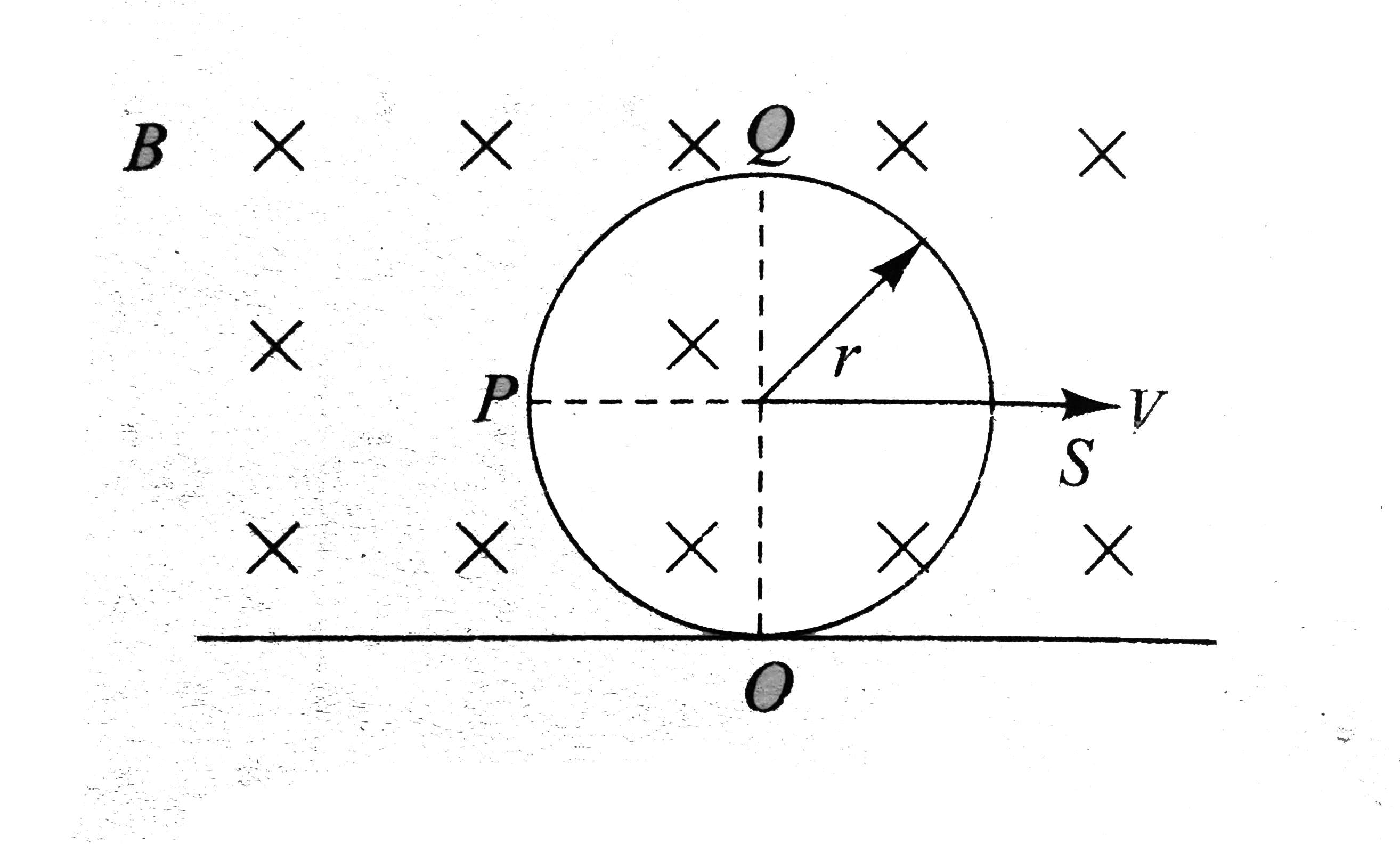 View Solution
View SolutionA vertical conducting ring of radius R falls vertically with a speed V in a horizontal uniform magnetic field B which is perpendicular to the plane of the ring. Which of the following statements is correct?
 View Solution
View SolutionA semicircle conducting ring of radius R is placed in the xy plane, as shown in Fig. A uniform magnetic field is set up along the x-axis. No emf, will be induced in the ring if
 View Solution
View SolutionA horizontal ring of radius r spins about its axis with an angular velocity ω in a uniform vertical magnetic field of magnitude B. The emf induced in the ring is
View Solution

