A coil having a resistance of 5 Ω and an inductance of 0.02H is arranged in parallel with another coil having a resistance of 1Ω and an inductance of 0.08H. Calculate the power absorbed when a voltage of 100V at 50Hz is applied.
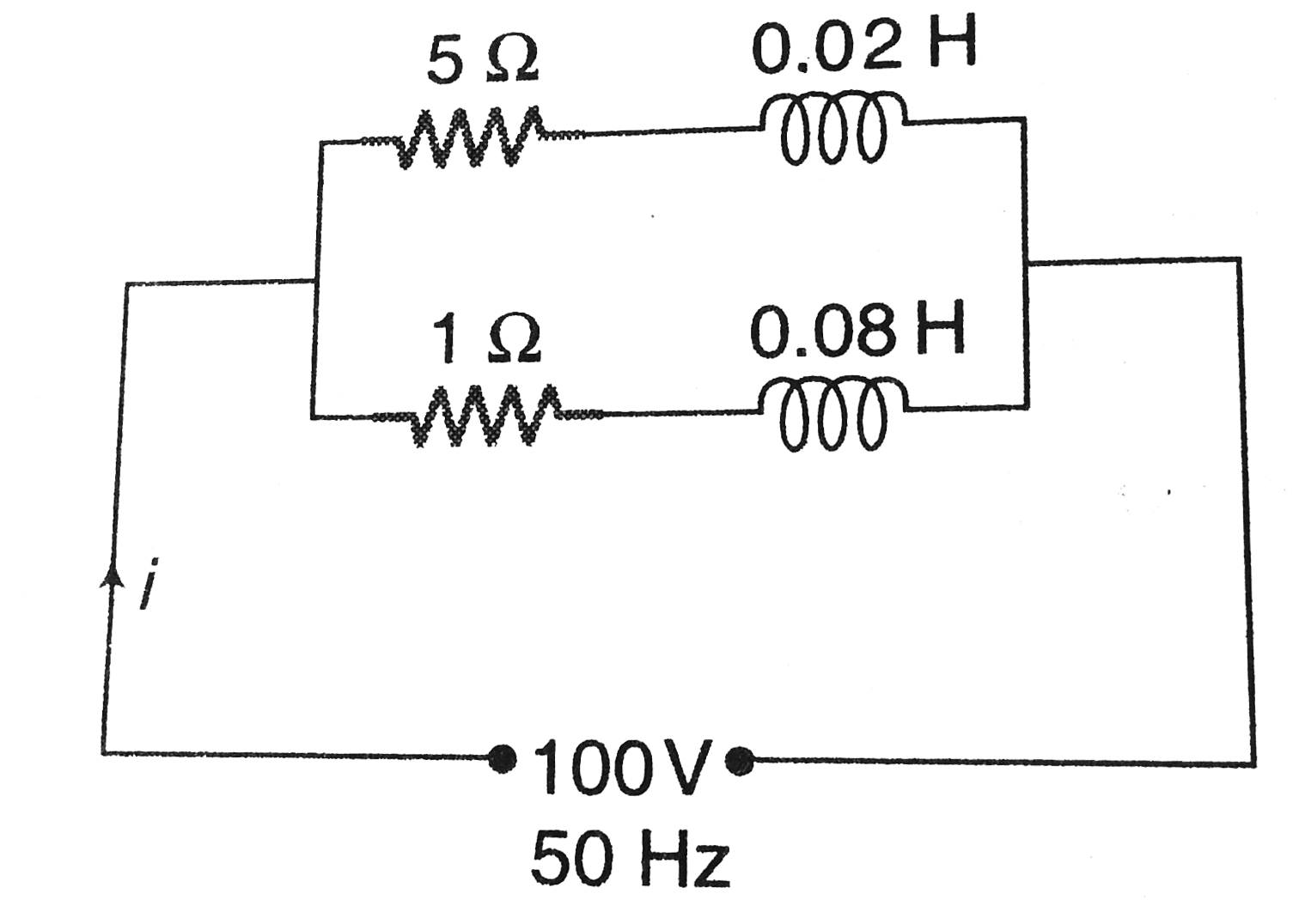 .
.
XL_1=ωL1=(2π×50)(0.02)=6.25Ω
∴Z1=√R21+X2L1
=√(5)2+(6.28)2=8.0Ω
P1=(Irms)1Vrmscosϕ1=(1008)(100)(58)
=781.25W
XL2=ωL2=(2π×50)(0.08)
=25.13Ω
∴Z2=√R22+X2L2
=25.15Ω
=(10025.15)(100)(125.15)
=15.8W
∴PTotal=P1+P2=797W.
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - JEE MAIN
62 Videos
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Level 2 Comprehension
6 Videos
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MEDICAL ENTRANCES GALLERY
42 Videos
Similar Questions
An alternating emf of 200V, 50Hz is applied to an L-R circuit, having a resistances R of 10Ω and an inductance L of 0.05H connected in series. Calculate:
Impedance
View SolutionA coil of resistance 10Ω and an inductance 5H is connected to a 100 volt battery. Then energy stored in the coil is
View SolutionAn alternating emf of 200V, 50Hz is applied to an L-R circuit, having a resistances R of 10Ω and an inductance L of 0.05H connected in series. Calculate:
Current flowing in the circuit
View SolutionA coil having a resistance of 50.0Ω and an inductance of 0.500 henry is connected to an AC source of 110 volts, 50.0 cycle/s. Find the rms value of the current in the circuit.
View SolutionAn rms voltage of 110 V is applied across a series circuit having a resistance 11Ω and an impedance 22Ω. The power consumed is
View SolutionA circuit has a resistance of 11Ω, an inductive reactance of 25Ω, and a capacitive resistance of 18Ω. It is connected to an AC source of 260V and 50Hz. The current through the circuit (in amperes) is
View SolutionA capacitor of capacitance 250 pF is connected in parallel with a choke coil having inductance of 1.6×10−2H and resistance 20Ω. Calculate
(a) the resonance frequency and
(b) the circuit impedance at resonance.
View SolutionIn LC oscillation resistance is 100 Ω and inductance and capacitance is 1 H and 10μF . Find the half power of frequency .
View SolutionThe resistance of a heater coil is 110Ω. A resistance R is connected in parallel with it and the combination is joined in series with a resistance of 11Ω to a 220V main line. The heater operates with a power of 110W. The value of R in Ω is
View SolutionA choke coil of resistance 5Ω and inductance 0.6 H is in series with a capacitance of 10(μ)F. If a voltage of 200 V is applied and the frequency is adjusted to resonance, the current and voltage across the inductance and capacitance are (I0),(V0)and(V1) respectively. we have
View Solution
