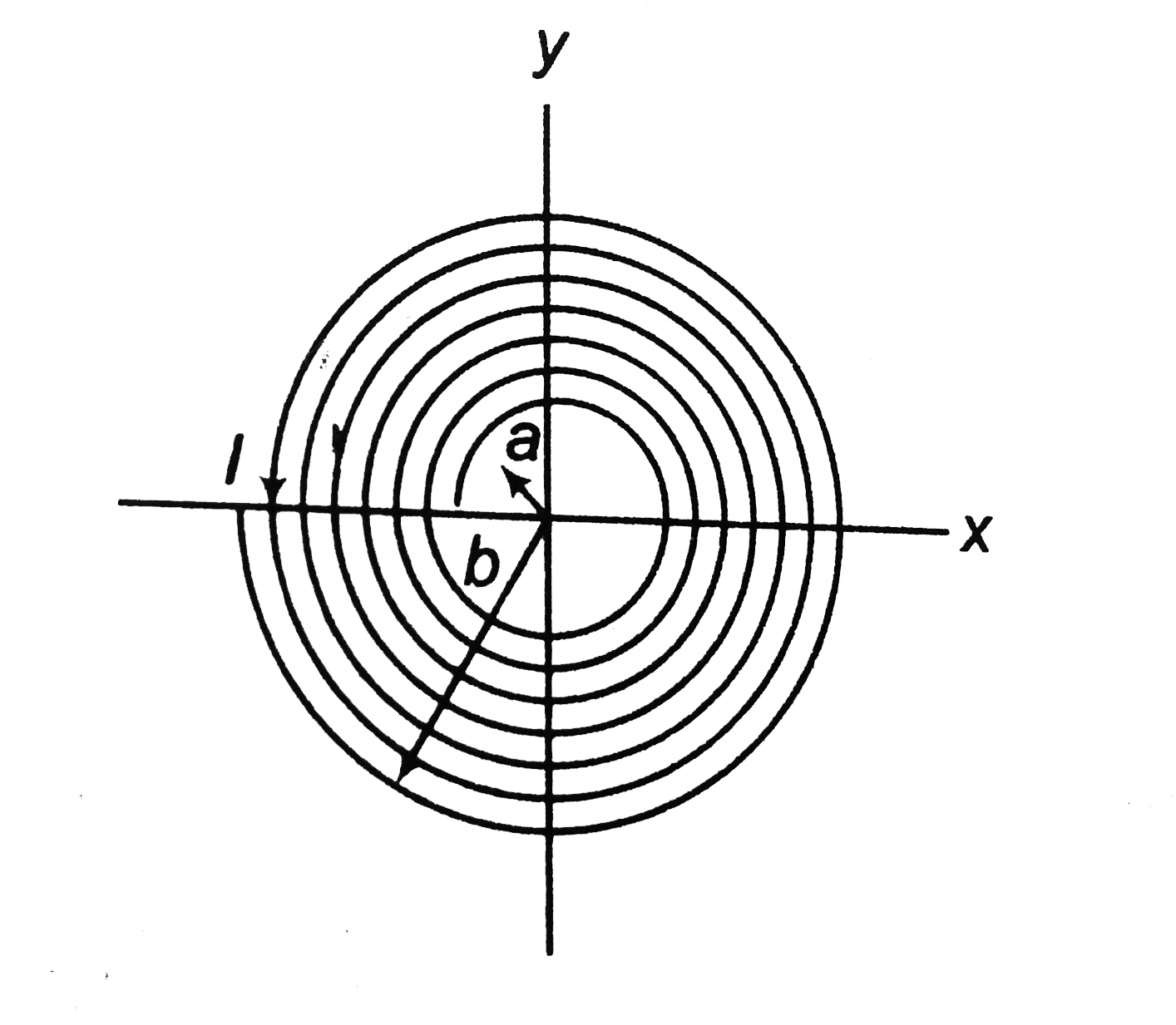
A coil having $N$ turns is would tightly in the form of a spiral with inner and outer radii a and $b$ respectively. When a current $I$ passes through the coil, the magnetic field at the centre is.

$\frac{μ_{0} N I}{b}$

$\frac{2 μ_{0} N I}{a}$

$\frac{μ_{0} N I}{2 (b - a)} ln \frac{b}{a}$

$\frac{μ_{0} I N}{(b - a)} ln \frac{b}{a}$ .

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

A coil having $N$ turns is would tightly in the form of a spiral with inner and outer radii a and $b$ respectively. When a current $I$ passes through the coil, the magnetic field at the centre is.
A $\frac{μ_{0} N I}{b - a}$
B $\frac{μ_{0} N I}{2 π (b - a)}$
C $\frac{μ_{0} N I}{2 (b - a)} In \frac{b}{a}$
D $\frac{μ_{0} N I}{b - a} In \frac{b}{a}$
A coil having N turns is wound tightly in the form of a spiral with inner and outer radii a and b respectively. A current I passes through the coil. The magnetic moment of the spriral is
A $M = \frac{μ_{0} N I}{3} \frac{b^{3} - a^{3}}{{(b - a)}^{3}}$
B $M = \frac{π N I}{3} \frac{{(b - a)}^{3}}{b^{3} - a^{3}}$
C $M = \frac{π N I}{3} \frac{(b^{3} - a^{3})}{{(b - a)}^{3}}$
D $M = \frac{μ_{0} N I}{3} \frac{{(b - a)}^{3}}{b^{3} - a^{3}}$
A coil of n number of tums is wound tightly in the form of a spiral with inner and outer radii a and b respectively. When a current of strength / is passed through the coil, the magnetic field at its centre is
A $\frac{μ_{0} n l}{(b - a)} {log}_{e} \frac{b}{a}$
B $\frac{μ_{0} n l}{2 (b - a)}$
C $\frac{2 μ_{0} n l}{b}$
D $\frac{μ_{0} n l}{2 (b - a)} {log}_{e} \frac{b}{a}$