A current- carrying straight wire is kept along the axis of a circular loop carrying a current. The straight wire
will exert an inward force on the circular loop
will exert an outward force on the circular loop
will not exert any force on the circular loop
will exert a force on the circular loop parallel to itself.
The correct Answer is:C
To solve the problem of a current-carrying straight wire placed along the axis of a circular loop carrying a current, we can follow these steps:
Step 1: Understand the Configuration
We have a circular loop with a current flowing through it, and a straight wire also carrying a current is positioned along the axis of the loop.
Step 2: Determine the Magnetic Field Due to the Straight Wire
The magnetic field (B) created by a long straight wire carrying current (I) at a distance (r) from the wire is given by the formula:
B=μ0I2πr
where μ0 is the permeability of free space.
Step 3: Analyze the Magnetic Field Direction
Using the right-hand rule, we can determine the direction of the magnetic field produced by the straight wire. If the current in the wire flows in a certain direction, the magnetic field will circulate around the wire in a direction determined by the right-hand rule.
Step 4: Calculate the Force on the Circular Loop
The force (F) on a segment of the circular loop due to the magnetic field created by the straight wire can be calculated using the formula:
F=BILsinθ
where:
- B is the magnetic field due to the straight wire,
- I is the current in the circular loop,
- L is the length of the segment of the loop in the magnetic field,
- θ is the angle between the direction of the current in the loop and the magnetic field.
Step 5: Determine the Angle θ
In this case, since the straight wire is along the axis of the circular loop, the angle θ between the magnetic field produced by the straight wire and the current in the loop is 0 degrees. Therefore:
sinθ=sin0=0
Step 6: Calculate the Force
Substituting sinθ=0 into the force equation:
F=BIL⋅0=0
This indicates that there is no force exerted on the circular loop by the straight wire.
Conclusion
The straight wire will not exert any force on the circular loop carrying current.
Final Answer
The answer to the question is option C: The straight wire will not exert any force on the circular loop.
---
We have a circular loop with a current flowing through it, and a straight wire also carrying a current is positioned along the axis of the loop.
The magnetic field (B) created by a long straight wire carrying current (I) at a distance (r) from the wire is given by the formula:
B=μ0I2πr
where μ0 is the permeability of free space.
Using the right-hand rule, we can determine the direction of the magnetic field produced by the straight wire. If the current in the wire flows in a certain direction, the magnetic field will circulate around the wire in a direction determined by the right-hand rule.
The force (F) on a segment of the circular loop due to the magnetic field created by the straight wire can be calculated using the formula:
F=BILsinθ
where:
- B is the magnetic field due to the straight wire,
- I is the current in the circular loop,
- L is the length of the segment of the loop in the magnetic field,
- θ is the angle between the direction of the current in the loop and the magnetic field.
In this case, since the straight wire is along the axis of the circular loop, the angle θ between the magnetic field produced by the straight wire and the current in the loop is 0 degrees. Therefore:
sinθ=sin0=0
Substituting sinθ=0 into the force equation:
F=BIL⋅0=0
This indicates that there is no force exerted on the circular loop by the straight wire.
The straight wire will not exert any force on the circular loop carrying current.
The answer to the question is option C: The straight wire will not exert any force on the circular loop.
Topper's Solved these Questions
- MAGNETIC FIELD DUE TO CURRENTBOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Objective 2
7 Videos
- MAGNETIC FIELD DUE TO CURRENTBOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - exercise
60 Videos
- MAGNETIC FIELD DUE TO CURRENTBOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - worked out examples
15 Videos
- MAGNETIC FIELDBOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELDEXERCISE - objective2
9 Videos
- MAGNETIC PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC PROPERTIES OF MATTEREXERCISE - Short Answer
6 Videos
Similar Questions
A current carrying straight wire passing inside a triangular loop as shown in Figure. The current in the wire is perpendicular to paper inwards. Find the direction of the induced current in the loop if current in the wire is increased.

View SolutionThe figure shows a long straigh wire carryhing a current I1 along the axis of a circular ring carrying a current I_2. Identify the correct statement.
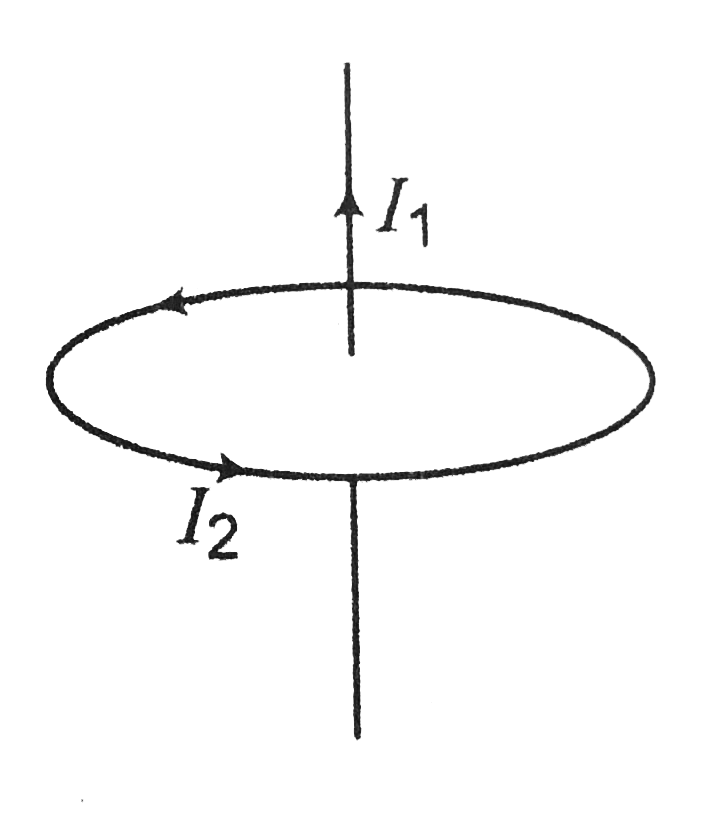
View SolutionA current carrying infinitely long wire is kept along the diameter of a circular wire loop, without touching it, the correct statements(s) is(are)
View SolutionA current carrying infinitely long wire is kept along the diameter of a circular wire loop, without touching it, the correct statements(s) is(are)
View SolutionA current carrying infinitely long wire is kept along the diameter of a circular wire loop, without touching it, the correct statements(s) is(are)
View SolutionA long straight current carrying wire passes normally through the centre of circular loop. If the current through the wire increases, will there be an induced emf in the loop ? Justify.
View SolutionAn infinite current-carrying conductor is placed along the z-axis and a wire loop is kept in the x−y plane. The current in the conductor is increasing with time. Then the
View SolutionA straight wire of diametre 0.5 mm carrying a current of 1 A is replaced by another wire of 1mm diametre carrying same current. The strenth of magnetic field far away is
View SolutionA long straight wire is carrying current I in + z direction .The x-y plane contains a closed circular loop carrying current I2 and not encircling the straight wire.The force on the loop will be
View SolutionA rectangular loop is placed near a current carrying straight wire as shown in the figure. If the loop is rotated about an axis passing throught one of its sides find the direction of induced current in the loop.
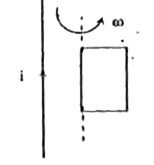
View Solution
