A current- carrying straight wire is kept along the axis of a circular loop carrying a current. The straight wire
will exert an inward force on the circular loop
will exert an outward force on the circular loop
will not exert any force on the circular loop
will exert a force on the circular loop parallel to itself.
The correct Answer is:C
To solve the problem of a current-carrying straight wire kept along the axis of a circular loop carrying a current, we will analyze the magnetic fields and forces involved.
1. Identify the Setup:
- We have a circular loop carrying a current (let's denote this current as I1).
- A straight wire carrying a current (denote this as I2) is placed along the axis of the circular loop.
2. Determine the Direction of Current:
- Assume the current in the circular loop flows in a clockwise direction when viewed from one end.
- The straight wire also carries a current, which we will assume flows in a specific direction (let's say upward for this explanation).
3. Use the Right-Hand Rule:
- For the straight wire, apply the right-hand rule to determine the direction of the magnetic field it produces.
- Point your thumb in the direction of the current in the straight wire; your fingers will curl in the direction of the magnetic field lines.
- The magnetic field due to the straight wire will form concentric circles around the wire.
4. Magnetic Field at the Loop:
- At the center of the circular loop, the magnetic field B due to the straight wire can be calculated using the formula:
B=μ0I22πr
where r is the distance from the wire to the center of the loop.
5. Force on the Circular Loop:
- The force F on a segment of the circular loop due to the magnetic field from the straight wire can be calculated using the formula:
F=I1dl×B
- Here, dl is the differential length element of the loop.
6. Direction of Force:
- Since the magnetic field B and the current I1 in the loop are perpendicular to each other at the center of the loop, the angle between dl and B is 0 degrees.
- Therefore, F=I1dlBsin(0)=0.
- This indicates that there is no net force acting on the circular loop due to the straight wire.
7. Conclusion:
- Since the force on the circular loop is zero, it will remain in equilibrium and will not experience any movement due to the presence of the straight wire.
Final Answer:
The correct option is that no force is exerted on the circular loop due to the straight wire.
- We have a circular loop carrying a current (let's denote this current as I1).
- A straight wire carrying a current (denote this as I2) is placed along the axis of the circular loop.
- Assume the current in the circular loop flows in a clockwise direction when viewed from one end.
- The straight wire also carries a current, which we will assume flows in a specific direction (let's say upward for this explanation).
- For the straight wire, apply the right-hand rule to determine the direction of the magnetic field it produces.
- Point your thumb in the direction of the current in the straight wire; your fingers will curl in the direction of the magnetic field lines.
- The magnetic field due to the straight wire will form concentric circles around the wire.
- At the center of the circular loop, the magnetic field B due to the straight wire can be calculated using the formula:
B=μ0I22πr
where r is the distance from the wire to the center of the loop.
- The force F on a segment of the circular loop due to the magnetic field from the straight wire can be calculated using the formula:
F=I1dl×B
- Here, dl is the differential length element of the loop.
- Since the magnetic field B and the current I1 in the loop are perpendicular to each other at the center of the loop, the angle between dl and B is 0 degrees.
- Therefore, F=I1dlBsin(0)=0.
- This indicates that there is no net force acting on the circular loop due to the straight wire.
- Since the force on the circular loop is zero, it will remain in equilibrium and will not experience any movement due to the presence of the straight wire.
The correct option is that no force is exerted on the circular loop due to the straight wire.
Topper's Solved these Questions
- MAGNETIC FIELD DUE TO CURRENTBook:HC VERMAChapter:MAGNETIC FIELD DUE TO CURRENTExercise:Objective 2
Explore 7 Videos
- MAGNETIC FIELD DUE TO CURRENTBook:HC VERMAChapter:MAGNETIC FIELD DUE TO CURRENTExercise:Exercises
Explore 61 Videos
- MAGNETIC FIELD DUE TO CURRENTBook:HC VERMAChapter:MAGNETIC FIELD DUE TO CURRENTExercise:Short Answer
Explore 12 Videos
- MAGNETIC FIELDBook:HC VERMAChapter:MAGNETIC FIELDExercise:Exercises
Explore 61 Videos
- MAGNETIC PROPERTIES OF MATTERBook:HC VERMAChapter:MAGNETIC PROPERTIES OF MATTERExercise:Exercises
Explore 9 Videos
Similar Questions
A current carrying straight wire passing inside a triangular loop as shown in Figure. The current in the wire is perpendicular to paper inwards. Find the direction of the induced current in the loop if current in the wire is increased.

View SolutionA long straight current carrying wire passes normally through the centre of circular loop. If the current through the wire increases, will there be an induced emf in the loop ? Justify.
View Solution
Knowledge Check
A current-carrying, straight wire is kept along the axis of a square loop carrying a current.The straight wire
Awill exert an inward force on the square loopBwill exert an outward force on the square loopCwill not exert any force on the square loopDwill exert a force on the square loop parallel to itself.A straight current carrying conductor is kept along the axis of circular loop canying current. The force exerted by the straight conductor on the loop is
Aperpendicular to the plane of the loopBin the plane of the loop, away from the centerCin the plane of the loop, towards the centerDzeroThe figure shows a long straight wire carrying a current I1 along the axis of a circular ring carrying a current I_2. Identify the correct statement.
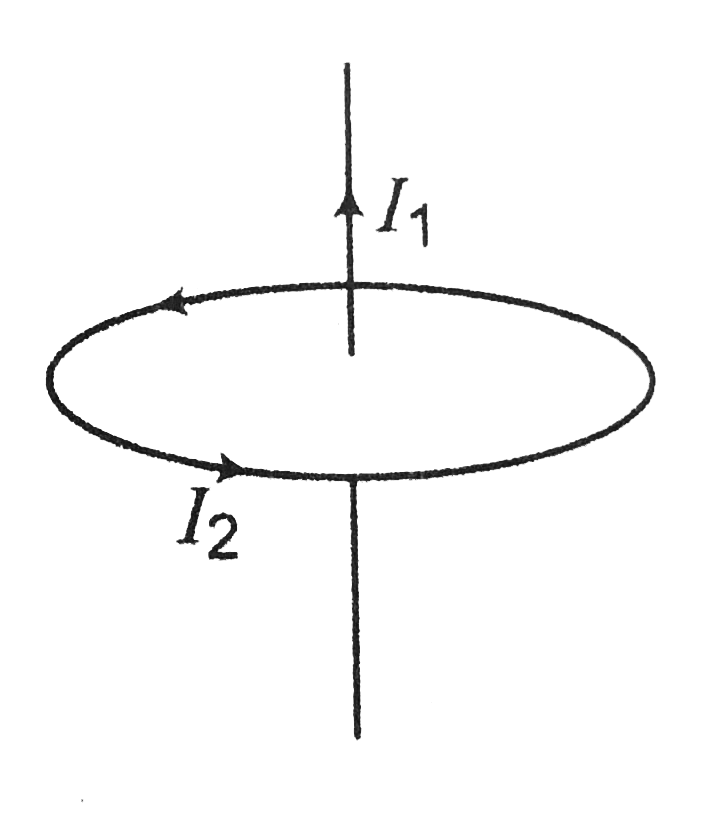 AStraight wire attracts the ringBStraight wire attracts a small element of the ringCStraight wire does not attract any small element of the ringDnone of these
AStraight wire attracts the ringBStraight wire attracts a small element of the ringCStraight wire does not attract any small element of the ringDnone of these
A long straight current carrying wire passes normally through the centre of circular loop. If the current through the wire increases, will there be an induced emf in the loop ? Justify.
View SolutionA current carrying wire is placed along the axis of a uniformly charged circular ring. If the ring starts rotating about its central axis what will be force on the current carrying wire due to the moving charges of ring.
View SolutionA current-carrying infinitely long wire is kept along the diameter of a circular wire loop, without touching it. The correct statement(s) is (are)
View SolutionA current-carrying infinitely long wire is kept along the diameter of a circular wire loop, without touching it. The correct statement(s) is (are)
View SolutionThere is one long straight conductor carrying current which is kept along the dimaeter of a circular loop without touching it.
View Solution