A current I flows through a thin wire shaped as regular polygon of n sides which can be inscribed in a circle of radius R. The magnetic fiedl induction at the center of polygon due to one side of the polygon is

Video Solution

Text Solution

Verified by Experts

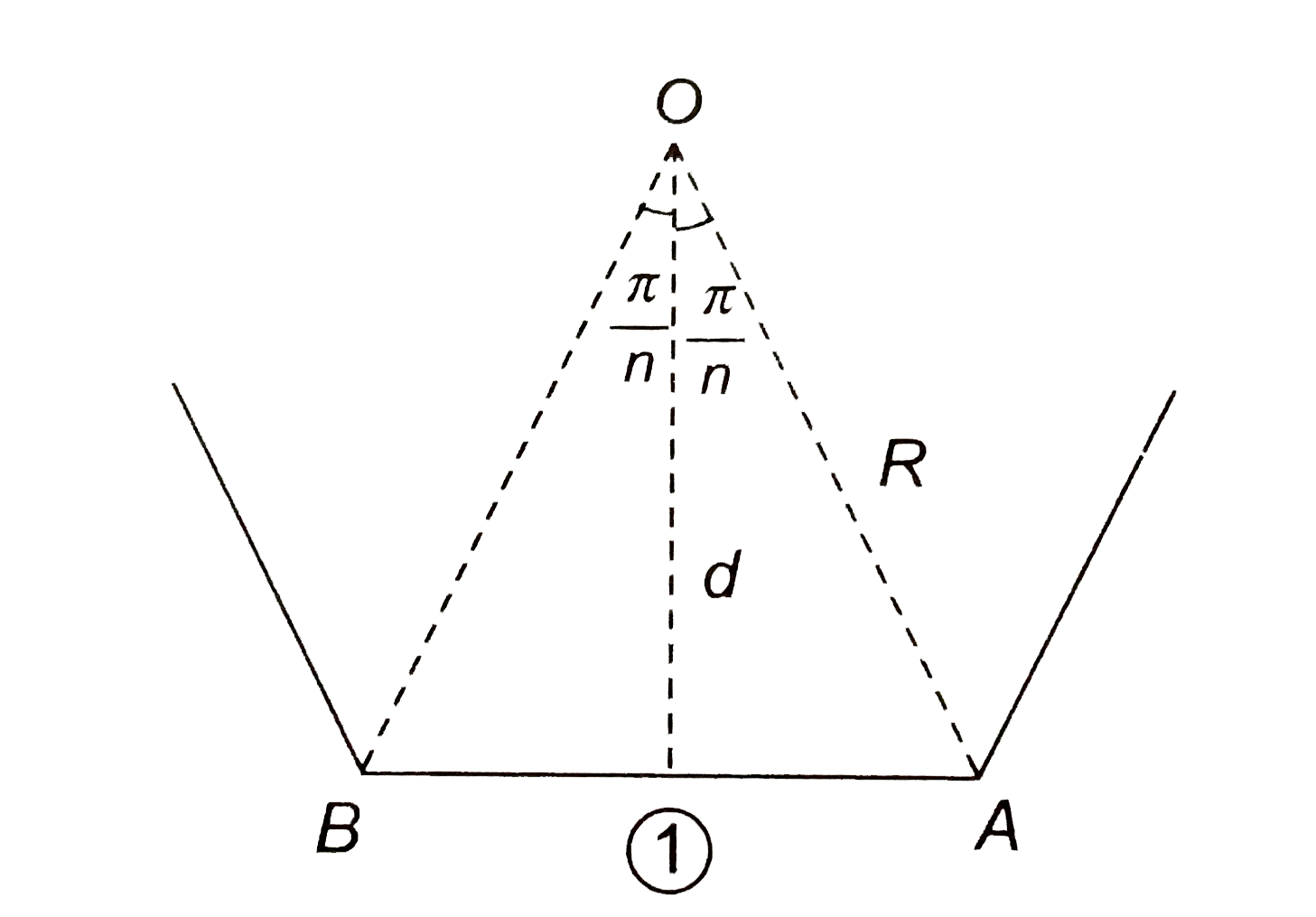
Angle subtended by one side at centre $= 2 π / n$
$d = R cos (π / n)$
Magnetic field at $O$ due to one side
$B_{1} = \frac{μ_{0} i}{4 π d} (sin . \frac{π}{n} + sin . \frac{π}{n})$
$= \frac{μ_{0} i}{4 π . R . cos (\frac{π}{n})} .2 sin (\frac{π}{n})$
$= \frac{μ_{0} tan (π / n)}{2 π R}$
Magnetic field due to polygon
$B_{O} = n B_{1} = \frac{μ_{0} n i tan (π / n)}{2 π R}$
when $n \to \infty$ , polygon becomes circle
$B_{O} = \frac{μ_{0} i}{2 R}$

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
In the following figure a wire bent in the form of a regular polygon of $n$ sides is inscribed in a circle of radius $a$ . Net magnetic field at centre will be

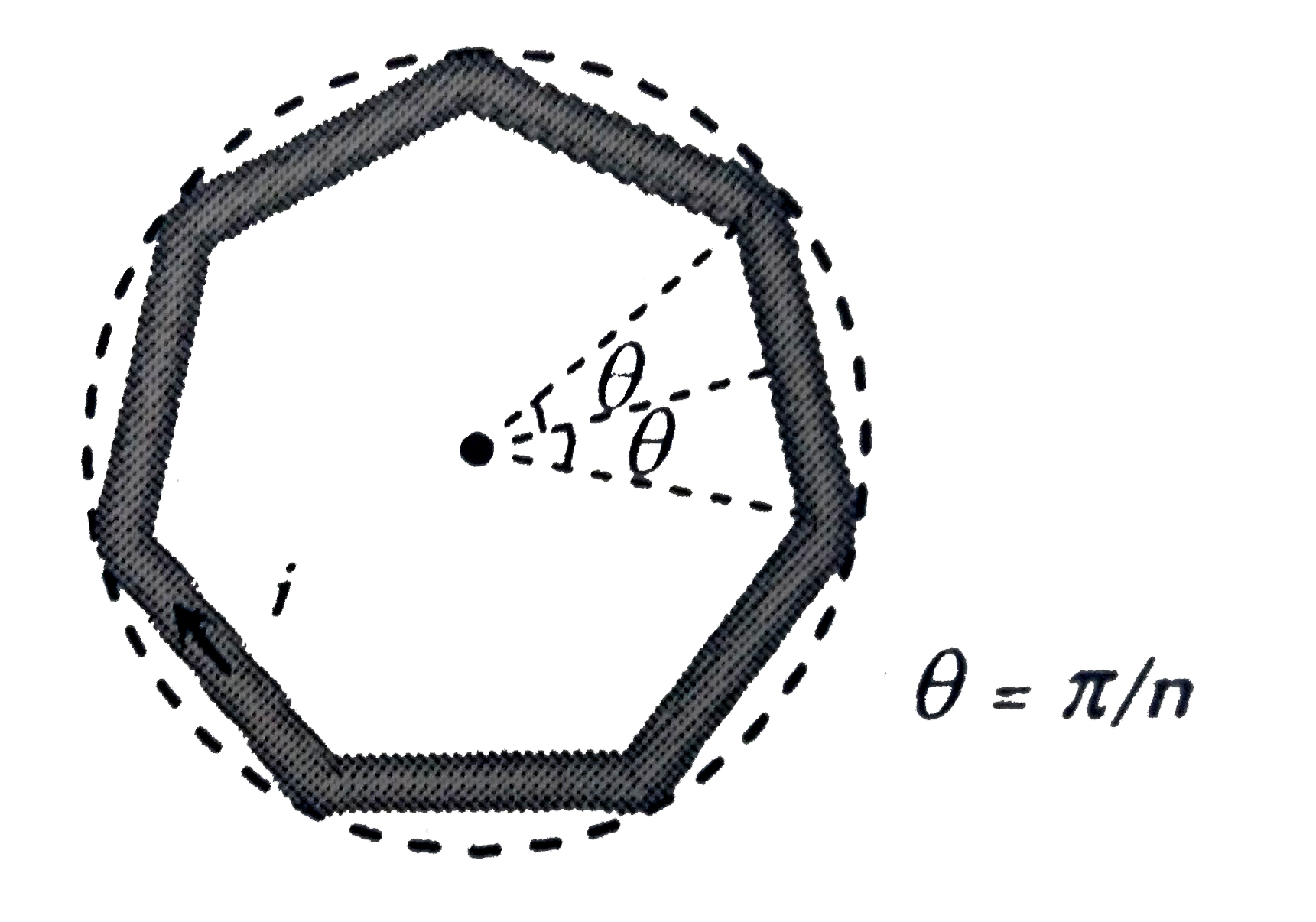A $\frac{μ_{0} i}{2 π a} tan \frac{π}{n}$
B $\frac{μ_{0} n i}{2 π a} tan \frac{π}{n}$
C $\frac{2}{π} \frac{n i}{a} μ_{0} tan \frac{π}{n}$
D $\frac{n i}{2 a} μ_{0} tan \frac{π}{n}$
Question 2 - Select One
A current I flows in a thin wire in the shape of a regular polygon n sides. The magnetic induction at the centre of the polygon when $n \to \infty$ is (R is the radius of its circumcircle)
A $\frac{μ_{0} n i}{2 π R} tan \frac{π}{6}$
B $\frac{μ_{0} n i}{2 π R} tan \frac{π}{R}$
C $\frac{μ_{0} i}{2 R}$
Dzero
Question 3 - Select One
The limit of the interior angle of a regular polygon of n sides as $n \to \infty$ is
A $π$
B $\frac{π}{3}$
C $\frac{3 π}{2}$
D $\frac{2 π}{3}$

CP SINGH-MAGNETIC FIELD-EXERCISE

Home
Ask Doubt
Profile