A current I is passing through a wire having two sections P and O of uniform diameters d and d/2 respectively. If the mean drift velocity of electrons in section P and Q is denoted by vP and vQ respectively, then
vP=vQ
vP=12vQ
vp=(14)vQ
vP=2vQ
The correct Answer is:C
To solve the problem, we will follow these steps:
Step 1: Understand the relationship between current, drift velocity, and cross-sectional area.
The current I flowing through a conductor is given by the formula:
I=neAvd
where:
- n = number of charge carriers per unit volume,
- e = charge of an electron,
- A = cross-sectional area of the wire,
- vd = drift velocity of the charge carriers.
Step 2: Calculate the cross-sectional areas of sections P and Q.
For section P with diameter d:
AP=π(d2)2=πd24
For section Q with diameter d2:
AQ=π(d/22)2=π(d4)2=πd216
Step 3: Write the expressions for current in both sections.
For section P:
I=neAPvP⟹I=ne(πd24)vP
For section Q:
I=neAQvQ⟹I=ne(πd216)vQ
Step 4: Set the two expressions for current equal to each other.
Since the current I is the same in both sections:
ne(πd24)vP=ne(πd216)vQ
Step 5: Cancel out common terms.
We can cancel ne and πd2 from both sides:
d24vP=d216vQ
Step 6: Simplify the equation.
Dividing both sides by d2:
14vP=116vQ
Multiplying both sides by 16:
4vP=vQ
Step 7: Rearranging gives the relationship between vP and vQ.
Thus, we can express vP in terms of vQ:
vP=14vQ
or
vPvQ=14
Final Answer:
The relationship between the mean drift velocities is:
vPvQ=14
---
The current I flowing through a conductor is given by the formula:
I=neAvd
where:
- n = number of charge carriers per unit volume,
- e = charge of an electron,
- A = cross-sectional area of the wire,
- vd = drift velocity of the charge carriers.
For section P with diameter d:
AP=π(d2)2=πd24
AQ=π(d/22)2=π(d4)2=πd216
For section P:
I=neAPvP⟹I=ne(πd24)vP
I=neAQvQ⟹I=ne(πd216)vQ
Since the current I is the same in both sections:
ne(πd24)vP=ne(πd216)vQ
We can cancel ne and πd2 from both sides:
d24vP=d216vQ
Dividing both sides by d2:
14vP=116vQ
Multiplying both sides by 16:
4vP=vQ
Thus, we can express vP in terms of vQ:
vP=14vQ
or
vPvQ=14
The relationship between the mean drift velocities is:
vPvQ=14
Topper's Solved these Questions
- ELECTRIC CURRENT & CIRCUITSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Combination of Resistance 1
14 Videos
- ELECTRIC CURRENT & CIRCUITSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Combination of Resistance 2
14 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Single Answer Correct Type
22 Videos
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Interger
8 Videos
Similar Questions
The area of cross-section of a current carrying conductor is A∘ and A∘4 at section (1) and (2) respectively If V1.V2 and E1.E2 be the drift velocity and electric field at section 1 and 2 respectively then
View SolutionTwo points P and Q are maintained at the potentials of 10V and -4V, respectively. The work done in moving 100 electrons from P to Q is:
View SolutionThe area of cross section of a current carrying conductor is A0 and A04 at section (1) and (2) respectively. If vα1,vα2andE1,E2 be the drift velocity and electric field at sections 1 and 2 respectively then :
View SolutionElectric current is passing through a solid conductor PQ from P to Q. The electric current densities at p and Q are in the ratio.
View Solutionif E denotes electric field in a uniform conductor and vd the corresponding drift velocity of free electrons in the conductor, then which of the following graph is correct
View SolutionA current .I. flows through a metallic wire of radius .r. and the free electrons in it drift with a velocity vd. Calculate the drift velocity of the free electrons through the wire of the same material, having double the radius, when same current flows through it.
View SolutionFigure shows wavefront P Passing through two systems A and B, and emerging as Q and then as R. Then systems A and B could, respectively, be
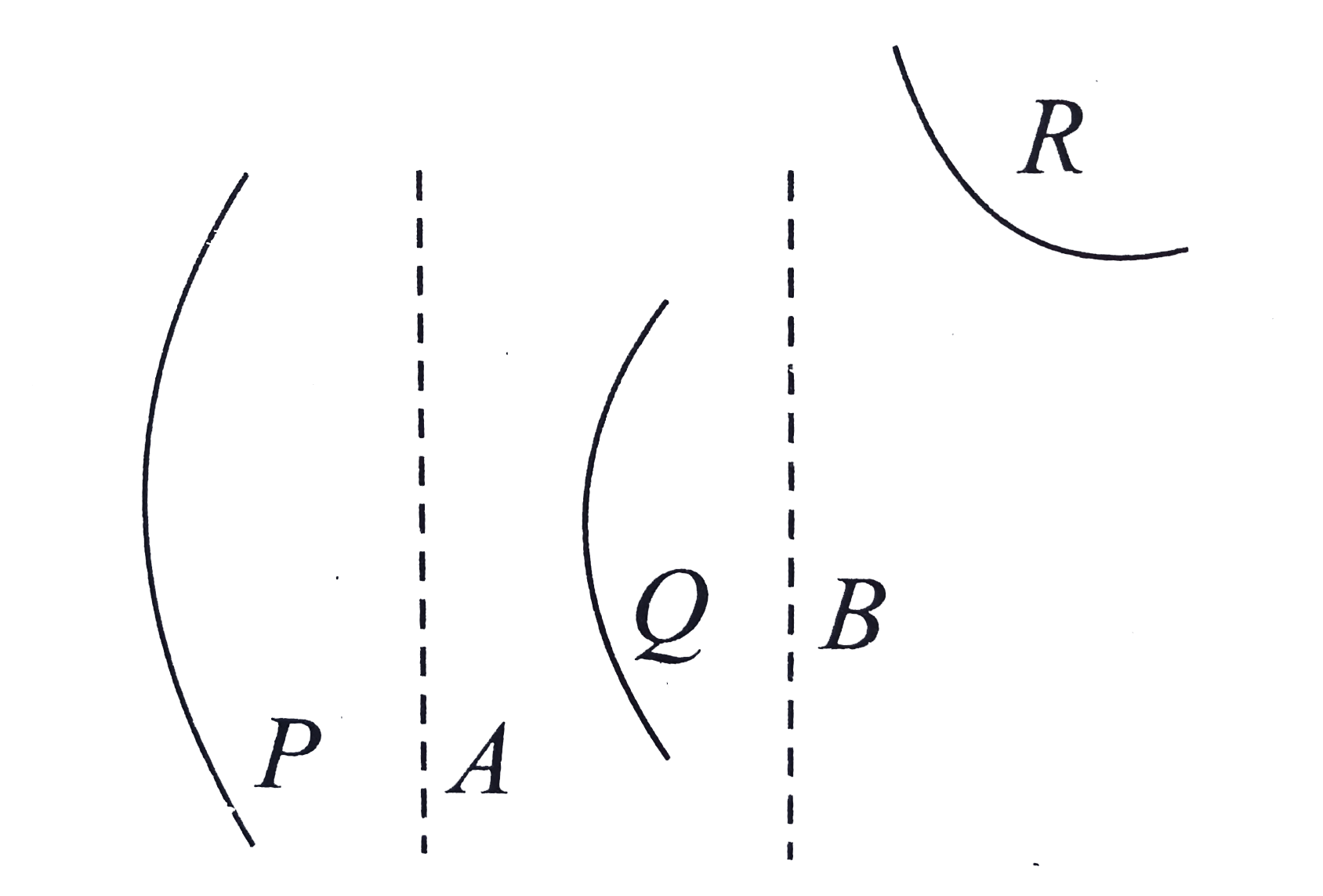
View SolutionP and Q are two distinct points on the parabola, y2=4x with parameters t and t1 respectively. If the normal at P passes through Q, then the minimum value of t21 is
View SolutionWhen the current i is flowing through a conductor, the drift velocity is v . If 2i current is flowed through the same metal but having double the area of cross-section, then the drift velocity will be
View SolutionWhen the current i is flowing through a conductor, the drift velocity is v . If 2i current is flowed through the same metal but having double the area of cross-section, then the drift velocity will be
View Solution

