A dielectric slab of thickness d is inserted in a parallel plate capacitor whose negative plate is at x=0 and positive plate is at x=3d. The slab is equidistant from the plates. The capacitor is given some charge. As one goes from 0 to 3d(1998).
the magnitude of the electric field remains the same
the direction of the electric remains the same
the electric potential increases continuously
the electric potential increases at first, then decreases and again increases
The correct Answer is:B, C
To solve the problem step by step, let's analyze the situation involving the parallel plate capacitor with a dielectric slab inserted between the plates.
Step 1: Understand the Configuration
We have a parallel plate capacitor with:
- Negative plate at x=0
- Positive plate at x=3d
- A dielectric slab of thickness d inserted equidistantly between the plates.
Step 2: Identify the Electric Field
The electric field E in a parallel plate capacitor is given by the formula:
E=Vd
where V is the potential difference between the plates and d is the separation between them. The direction of the electric field is from the positive plate to the negative plate.
Step 3: Determine the Effect of the Dielectric
When a dielectric slab is inserted, it affects the electric field. However, since the slab is equidistant from both plates, the electric field will still point from the positive plate to the negative plate, but its magnitude will change depending on the dielectric constant κ of the slab.
Step 4: Analyze the Electric Potential
The electric potential V at a distance x from the negative plate can be expressed as:
V(x)=−∫Edx
Since the electric field is directed towards the negative plate, the potential increases as we move from the negative plate to the positive plate.
Step 5: Evaluate the Options
1. Magnitude of the Electric Field: The magnitude of the electric field does not remain the same due to the presence of the dielectric. Thus, this option is incorrect.
2. Direction of the Electric Field: The direction of the electric field remains the same (from positive to negative). This option is correct.
3. Electric Potential: The electric potential increases continuously as we move from the negative plate to the positive plate. This option is correct.
4. Electric Potential Behavior: The electric potential does not increase and then decrease; it continuously increases. Thus, this option is incorrect.
Final Conclusion
The correct options are:
- B: The direction of the electric field remains the same.
- C: The electric potential increases continuously.
We have a parallel plate capacitor with:
- Negative plate at x=0
- Positive plate at x=3d
- A dielectric slab of thickness d inserted equidistantly between the plates.
The electric field E in a parallel plate capacitor is given by the formula:
E=Vd
where V is the potential difference between the plates and d is the separation between them. The direction of the electric field is from the positive plate to the negative plate.
When a dielectric slab is inserted, it affects the electric field. However, since the slab is equidistant from both plates, the electric field will still point from the positive plate to the negative plate, but its magnitude will change depending on the dielectric constant κ of the slab.
The electric potential V at a distance x from the negative plate can be expressed as:
V(x)=−∫Edx
Since the electric field is directed towards the negative plate, the potential increases as we move from the negative plate to the positive plate.
1. Magnitude of the Electric Field: The magnitude of the electric field does not remain the same due to the presence of the dielectric. Thus, this option is incorrect.
2. Direction of the Electric Field: The direction of the electric field remains the same (from positive to negative). This option is correct.
3. Electric Potential: The electric potential increases continuously as we move from the negative plate to the positive plate. This option is correct.
4. Electric Potential Behavior: The electric potential does not increase and then decrease; it continuously increases. Thus, this option is incorrect.
The correct options are:
- B: The direction of the electric field remains the same.
- C: The electric potential increases continuously.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (B) Chapter exercises
17 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.5
20 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
A dielectric slab is inserted between the plates of an isolated capacitor. The force between the plates will
View SolutionIn a parallel plate capacitor, the distance between the plates is d and potential difference across the plate is V. Energy stored per unit volume between the plates of capacitor is
View Solution
Knowledge Check
A copper plate of thickness b is placed inside a parallel plate capacitor of plate distance d and area A as shown in figure. The capacitance of capacitor is
 Aε0Ad+b2Bε0A2dCε0Ad−bD2ε0Ad+b2
Aε0Ad+b2Bε0A2dCε0Ad−bD2ε0Ad+b2
A parallel plate capacitor, with plate area A and plate separation d, is filled with a dielectric slabe as shown. What is the capacitance of the arrangement ?
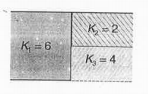
View SolutionA parallel plate capacitor of capacitance C (without dielectric) is filled by dielectric slabs as shown in figure. Then the new capacitance of the capacitor is
View SolutionA slab of copper of thickness b is inserted in between the plates of parallel plate capacitor as shown in figure. The separation of the plate is d. If b=d/2, then the ratio of capacities of the capacitor after and before inserting that slab will be

View SolutionA slab of copper of thickness y is inserted between the plates of parallel plate capacitor as shown in figure. The separation between the plates is d. If y=d4, then the ratio of capacitance of the capacitor after nd before inserting the slab is

View SolutionIn a parallel-plate capacitor, the region between the plates is filled by a dielectric slab. The capacitor is connected to a cell and the slab is taken out. Then
View SolutionA parallel plate capacitor is connected across a battery. Now, keeping the battery connected, a dielectric slab is inserted between the plates. In the process,
View SolutionA dielectric slab is placed between the plates of a parallel plate capacitor. Its capacitance
A. become zero
B. remains the same
C. decrease
D. increase
View Solution
