A diwali rocket moves vertically up with a constant acceleration a1=20//3ms−2. After sometives, its fuel gers exhausted ad then if falls freely with an acceleration a2=10ms−2, If themaximum height attained by the diwalin rocket is (h), using graphicalmerhod, find its speed when the fuel is just exausted. Assume h=50m.
To solve the problem step by step, we will analyze the motion of the Diwali rocket in two phases: the upward motion with fuel and the downward motion after the fuel is exhausted.
Step 1: Understanding the Motion Phases
1. Upward Motion: The rocket moves upward with a constant acceleration a1=203m/s2.
2. Downward Motion: After the fuel is exhausted, the rocket falls freely with an acceleration a2=10m/s2.
Step 2: Using Kinematic Equations
We need to find the speed of the rocket when the fuel is exhausted. Let's denote:
- v: speed of the rocket when the fuel is exhausted.
- t1: time taken to reach the maximum height during the upward motion.
- t2: time taken to fall back to the ground after the fuel is exhausted.
Step 3: Relating Speed and Time
Using the definition of acceleration, we can express the speed at the point when the fuel is exhausted:
- For upward motion:
v=a1t1
- For downward motion:
v=−a2t2
This gives us two equations:
1. v=a1t1 (1)
2. v=−a2t2 (2)
Step 4: Total Displacement
The total displacement h (maximum height) can be calculated using the area under the velocity-time (V-T) graph. The area under the graph consists of two parts:
1. The area during the upward motion (triangle).
2. The area during the downward motion (triangle).
The total displacement can be expressed as:
h=12vt1+12vt2
Step 5: Expressing Time in Terms of Velocity
From equations (1) and (2), we can express t1 and t2 in terms of v:
- From (1):
t1=va1
- From (2):
t2=va2
Step 6: Substitute Back into Displacement Equation
Substituting t1 and t2 into the displacement equation:
h=12v(va1)+12v(va2)
This simplifies to:
h=v22(1a1+1a2)
Step 7: Rearranging for Velocity
Rearranging the equation gives:
v2=2h(a1a2a1+a2)
Taking the square root:
v=√2h(a1a2a1+a2)
Step 8: Substituting Values
Now, substituting the given values:
- h=50m
- a1=203m/s2
- a2=10m/s2
Calculating:
v=
⎷2×50⎛⎝203×10203+10⎞⎠
Calculating the terms inside the square root:
=
⎷100⎛⎝2003203+303⎞⎠=
⎷100⎛⎝2003503⎞⎠=√100×4=√400=20m/s
Final Answer
The speed of the rocket when the fuel is just exhausted is v=20m/s.
---
1. Upward Motion: The rocket moves upward with a constant acceleration a1=203m/s2.
2. Downward Motion: After the fuel is exhausted, the rocket falls freely with an acceleration a2=10m/s2.
We need to find the speed of the rocket when the fuel is exhausted. Let's denote:
- v: speed of the rocket when the fuel is exhausted.
- t1: time taken to reach the maximum height during the upward motion.
- t2: time taken to fall back to the ground after the fuel is exhausted.
Using the definition of acceleration, we can express the speed at the point when the fuel is exhausted:
- For upward motion:
v=a1t1
- For downward motion:
v=−a2t2
This gives us two equations:
1. v=a1t1 (1)
2. v=−a2t2 (2)
The total displacement h (maximum height) can be calculated using the area under the velocity-time (V-T) graph. The area under the graph consists of two parts:
1. The area during the upward motion (triangle).
2. The area during the downward motion (triangle).
h=12vt1+12vt2
From equations (1) and (2), we can express t1 and t2 in terms of v:
- From (1):
t1=va1
- From (2):
t2=va2
Substituting t1 and t2 into the displacement equation:
h=12v(va1)+12v(va2)
This simplifies to:
h=v22(1a1+1a2)
Rearranging the equation gives:
v2=2h(a1a2a1+a2)
Taking the square root:
v=√2h(a1a2a1+a2)
Now, substituting the given values:
- h=50m
- a1=203m/s2
- a2=10m/s2
v=
⎷2×50⎛⎝203×10203+10⎞⎠
Calculating the terms inside the square root:
=
⎷100⎛⎝2003203+303⎞⎠=
⎷100⎛⎝2003503⎞⎠=√100×4=√400=20m/s
The speed of the rocket when the fuel is just exhausted is v=20m/s.
Topper's Solved these Questions
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.1
17 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.2
12 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer
9 Videos
Similar Questions
A rocket is fired vertically from the ground. It moves upwards with a constant acceleration 10m/s2 after 30 sec the fuel is finished. After what time from the instant of firing the rocket will attain the maximum height? g=10m/s2:-
View SolutionA rocket is fired upwards such that its engine takes 10 s to explods fully. Its velocity- time graph is as shown in the figure. Acceleration due to gravity is 10m/s2.
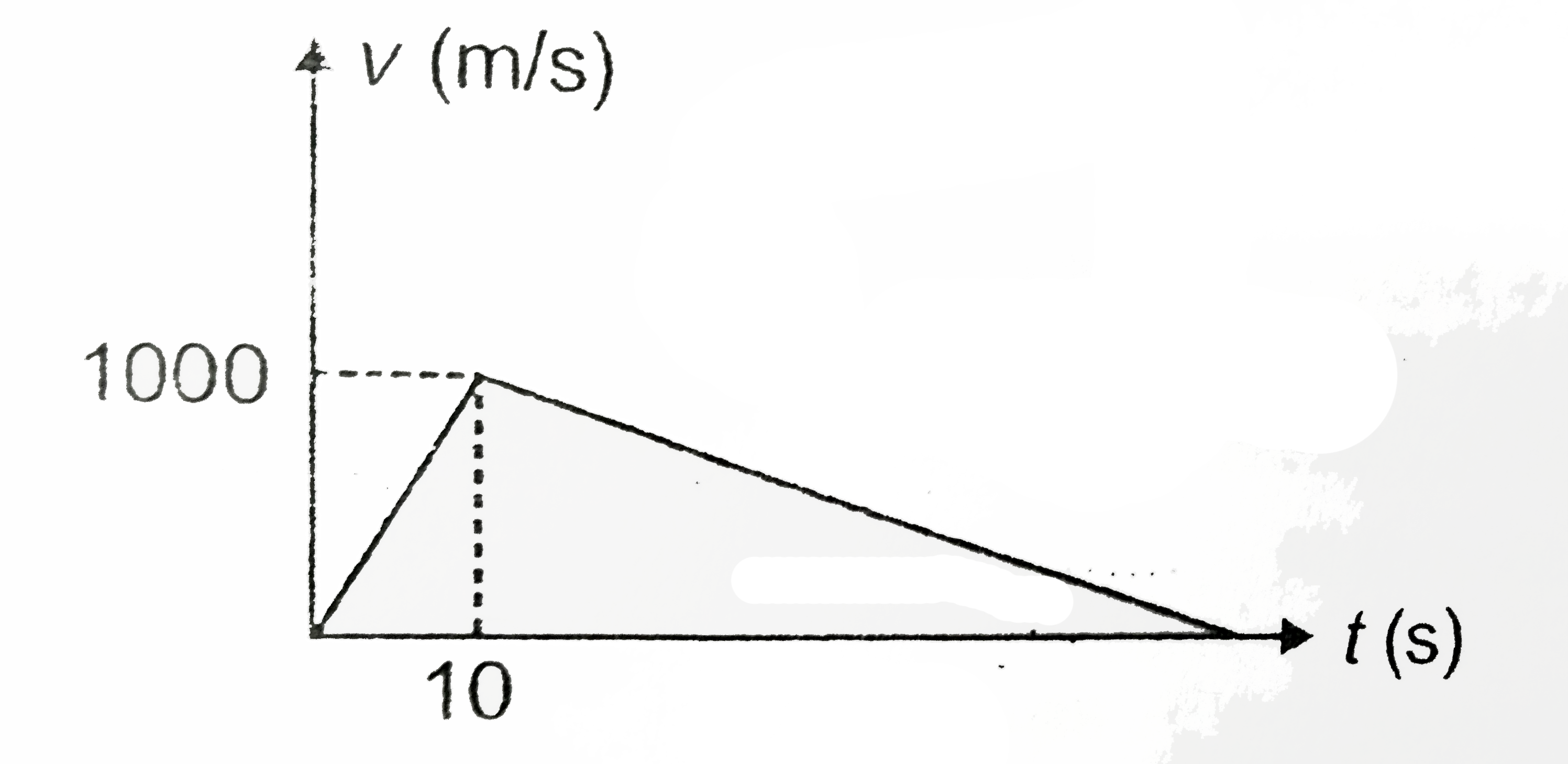
The maximum height attained by the rocket is
View SolutionA body is moving vertically upwards. Its velocity changes at a constant rate from 50m s−1 to 20m s−1 in 3 s. What is its acceleration ?
View SolutionA rocket is fired vertically up from the ground with an acceleration 10 m/s2. If its fuel is finished after 1 minute then calculate -
(a) Maximum velocity attained by rocket in ascending motion.
(b) Height attained by rocket fuel is finished.
(c ) Time taken by the rocket in the whole motion.
(d) Maximum height attained by rocket.
View SolutionThe exhaust velocity of gases with respect to a small rocket of mass 25 kg. is 28×102m/s. At what rate the fuel must burn so that it may rise up with an acceleration of 9.8m/s2?
View SolutionA particle is thrown vertically upward. Its velocity at half of the height is 10 m/s. Then the maximum height attained by it : -
(g=10m/s2)
View SolutionWhen a particle is thrown vertically upwards, its velocity at one third of its maximum height is 10√2m/s. The maximum height attained by it is
View SolutionA body starts from rest with an acceleration 2m/s2 till it attains the maximum velocity then retards to rest with 3m/s2. If total time taken is 10 second, then maximum speed attained is
View SolutionA rocket is fired vertically up from the ground with a resultant vertical acceleration of 10m/s2. The fuel is finished in 1 min and it continues to move up. (a) What is the maximum height reached? (b) Afte2r how much time from then will the maximum height be reached?(Take g=10m/s2)
View SolutionA rocket is fired vertically up from the ground with a resultant vertical acceleration of 10m/s2. The fuel is finished in 1 min and it continues to move up. (a) What is the maximum height reached? (b) Afte2r how much time from then will the maximum height be reached?(Take g=10m/s2)
View Solution
