A double-layer cylindrical capacitor consists of conductors of radii R1 and R3 and two layers of dielectrics of relative permittivtties ε1 and esπon2. The radius of the common surface of the dielectrics. Is R2 . The breakdown field strengths of the dielectrics are E1 and E2 respectively. What is the breakdown voltage of this capacitor if (a) ε1R1E1<ε2R2E2 (b)ε2R2E2>ε1E1R1?
[Hint: If E1=E1max and E2>,E2max dielectric 1 will break first and if E2=E2max'E1>E1max dielectric 2 will break first.]
The correct Answer is:(a)Vbreak=E1R1(InR2R1+ε1ε2InR3R2) (b)Vbreak=E2R2(ε2ε1InR2R1+InR3R2)
Topper's Solved these Questions
Similar Questions
There is a double-layer cylindrical capacitor whose parameters are shoen in Fig. The breakdown field strength values for these dielectrics are equal to E1 and E2 respectively. What is the breakdown voltage of this capacitor if ε1R1E1<ε2R2E2 ?
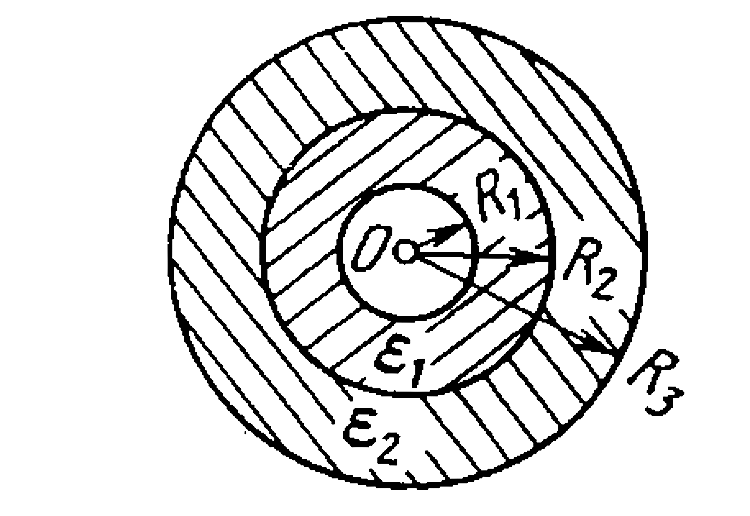
View SolutionFind the capacitance of an isolated ball-shaped conductor of radius R1 surrounded by an adjacent concentric layer of dielectric with permittivity ε and outside radius R2.
View Solution
Knowledge Check
The magnitudes of the gravitational field at distance r1 and r2 from the centre of a uniform sphere of radius R and mass M are E1 and E2 respectively. Then:
AE1E2=r1r2 if r1<R and r2<RBE1E2=r22r21 if r1<R and r2<RCE1E2=r22r32 if r1<R and r2<RDE1E2=r21r22 if r1<R and r2<RThe magnitudes of the gravitational field at distance r1 and r2 from the centre of a uniform sphere of radius R and mass M are E1 and E2 respectively. Then:
AE1E2=r1r2 ir r1<R and r2<RBE1E2=r22r21 if r1<R and r2<RCE1E2=r31r32 if r1<R and r2<RDE1E2=r21r22 ir r1<R and r2<RThe dimensions of 12ε0E2(ε0= permittivity of free space, E= electric field) is
A[MLT−1]B[ML−1T−2]C[MLT−2]D[ML2T−1]
A cylindrical capacitor is filled with two cyclindrical layers of dielectric with permittivity esπlon1 and esπlon2. The inside radii of the layers are equal to R1 and R2>R1. The maximum permissible values of electric field strength reaching teh breakdown value for both dielectrics simulttaneously ?
View SolutionThree dielectrics of relative permittivities εr1=1,εr2=2, and εr3=3
are introduced in a parallel plate capacitor of plate A and B.
 .
.
View SolutionThree dielectrics of relative permittivities εr1=1,εr2=2, and εr3=3
are introduced in a parallel plate capacitor of plate A and B. Find equivalent capacitance between A and B
 .
.
View SolutionThe dimensions of 1ε0e2hc are
View SolutionThe dimensions of 12ε0E2(ε0= permittivity of free space, E= electric field) is
View Solution
