A drunkard is walking along a straight road. He takes five steps forward and three steps backward and so on. Each step is 1m long and takes 1s. There is a pit on the road 11m, away from the starting point. The drunkard will fall into the pit after.
21 s
29 s
31 s
37 s
The correct Answer is:B
To solve the problem of the drunkard walking along a straight road and falling into a pit, we can break it down into steps:
1. Understand the Drunkard's Movement:
The drunkard takes 5 steps forward and then 3 steps backward. Each step is 1 meter long and takes 1 second.
2. Calculate the Net Movement in One Cycle:
- In one complete cycle (5 steps forward and 3 steps backward):
- Forward distance = 5 m
- Backward distance = 3 m
- Net distance covered in one cycle = Forward distance - Backward distance = 5 m - 3 m = 2 m.
3. Determine the Time Taken for One Cycle:
- Total steps in one cycle = 5 (forward) + 3 (backward) = 8 steps.
- Since each step takes 1 second, the total time for one cycle = 8 seconds.
4. Calculate the Number of Cycles to Reach the Pit:
- The pit is located 11 m away from the starting point.
- After each cycle, the drunkard moves 2 m forward.
- To find the number of complete cycles needed to reach or exceed 11 m:
- Distance after n cycles = 2n.
- We need to find n such that 2n ≥ 11.
- Solving for n: n = 11/2 = 5.5 cycles.
- Since the drunkard cannot complete half a cycle, he will complete 5 cycles.
5. Calculate the Distance After 5 Cycles:
- After 5 cycles, the distance covered = 2 * 5 = 10 m.
- Time taken for 5 cycles = 8 seconds/cycle * 5 cycles = 40 seconds.
6. Determine the Remaining Distance to the Pit:
- After 5 cycles, the drunkard is at 10 m. The pit is at 11 m, so he has 1 m left to walk.
7. Final Step to the Pit:
- In the next cycle, he will take 5 steps forward (1 m each), reaching 15 m, and then take 3 steps backward (1 m each), ending up at 12 m.
- However, since he only needs to walk 1 more meter to reach the pit, he will fall into the pit after taking 1 more step forward.
8. Calculate the Total Time Until He Falls:
- He has already taken 40 seconds for the first 5 cycles.
- He will take 1 more second to take that final step forward into the pit.
- Total time = 40 seconds + 1 second = 41 seconds.
Conclusion:
The drunkard will fall into the pit after 41 seconds.
The drunkard takes 5 steps forward and then 3 steps backward. Each step is 1 meter long and takes 1 second.
- In one complete cycle (5 steps forward and 3 steps backward):
- Forward distance = 5 m
- Backward distance = 3 m
- Net distance covered in one cycle = Forward distance - Backward distance = 5 m - 3 m = 2 m.
- Total steps in one cycle = 5 (forward) + 3 (backward) = 8 steps.
- Since each step takes 1 second, the total time for one cycle = 8 seconds.
- The pit is located 11 m away from the starting point.
- After each cycle, the drunkard moves 2 m forward.
- To find the number of complete cycles needed to reach or exceed 11 m:
- Distance after n cycles = 2n.
- We need to find n such that 2n ≥ 11.
- Solving for n: n = 11/2 = 5.5 cycles.
- Since the drunkard cannot complete half a cycle, he will complete 5 cycles.
- After 5 cycles, the distance covered = 2 * 5 = 10 m.
- Time taken for 5 cycles = 8 seconds/cycle * 5 cycles = 40 seconds.
- After 5 cycles, the drunkard is at 10 m. The pit is at 11 m, so he has 1 m left to walk.
- In the next cycle, he will take 5 steps forward (1 m each), reaching 15 m, and then take 3 steps backward (1 m each), ending up at 12 m.
- However, since he only needs to walk 1 more meter to reach the pit, he will fall into the pit after taking 1 more step forward.
- He has already taken 40 seconds for the first 5 cycles.
- He will take 1 more second to take that final step forward into the pit.
- Total time = 40 seconds + 1 second = 41 seconds.
The drunkard will fall into the pit after 41 seconds.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINEBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - AVERAGE VELOCITY AND AVERAGE SPEED
8 Videos
- MOTION IN A STRAIGHT LINEBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - INSTANTANEOUS VELOCITY AND SPEED
16 Videos
- MOTION IN A STRAIGHT LINEBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - NCERT Exemplar
6 Videos
- MOTION IN A PLANE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A PLANE EXERCISE - Assertion And Reason
15 Videos
- OSCILLATIONS BOOK - NCERT FINGERTPS ENGLISHCHAPTER - OSCILLATIONS EXERCISE - Assertion And Reason
15 Videos
Similar Questions
A drunkard walking in a narrow lane takes 5 steps forward and 3 steps backward, followed again by 5 steps forward and 3 steps backward, and so on. Each step is 1 m long and requires 1 s. Plot the x-t graph of his motion. Determine graphically and otherwise how long the drunkard takes to fall in a pit 13 m away from the start.
View SolutionA drunkard walking in a narrow lane takes 5 steps forward and 3 steps backward, followed again by 5 steps forward and 3 steps backward, and so on. Each step is 1 m long and requires 1 s. Plot the x−t graph of his motion. Determine graphically and otherwise how long the drunkard takes to fall in a pit 13 m away from the start
View Solution
Knowledge Check
A car moving along a straight road with speed of 144kmh−1 is brought to a stop within a distance of 200 m. How long does it take for the car to stop ?
A5 sB10 sC15 sD20 sThe rear side of a truck is open A box of 40kg mass is placed 5m away from the open end as shown in The coefficient of friction between the box and the surface is 0.15. On a straight road, the truck starts from rest and accel erating with 2m/s2. At what dis tance from the starting point does the box dis-tance from the starting point does the box fall from the truck? (Ignore the size of the box)
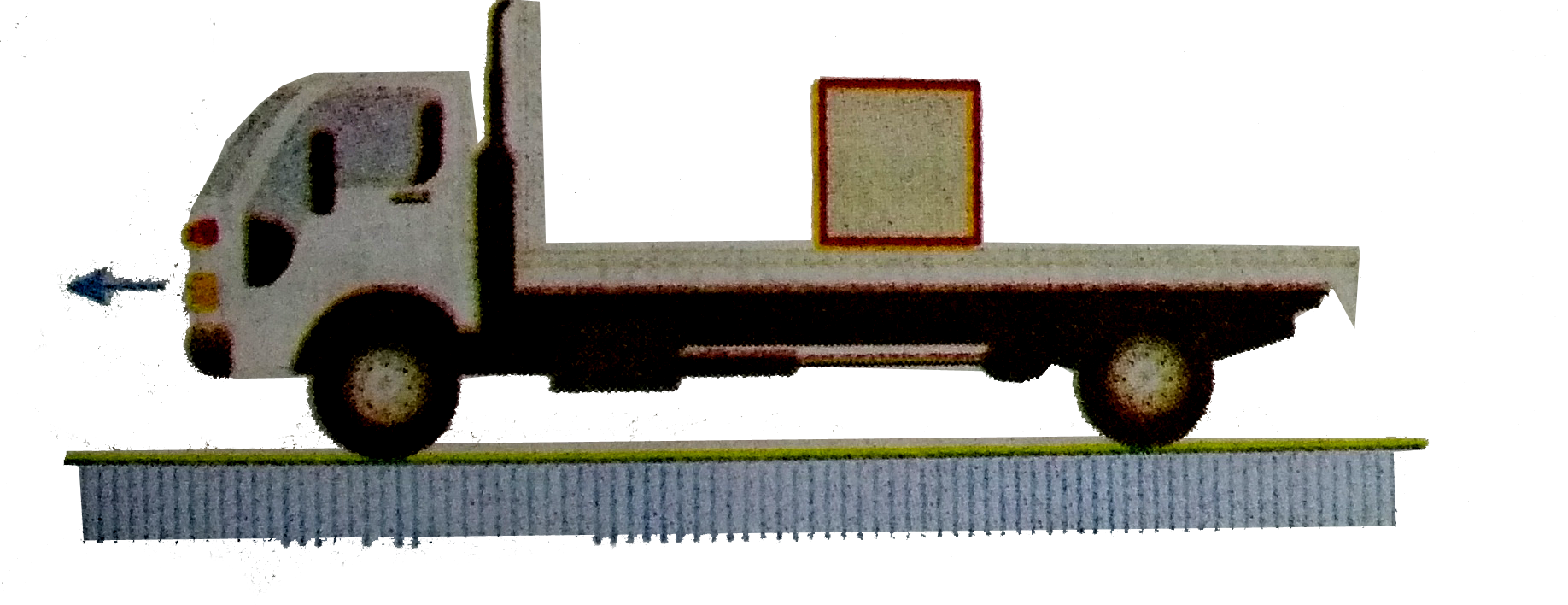 .A20mB30mC40mD50m
.A20mB30mC40mD50mAn auto travelling along a straight road increases its speed from 30.0ms−1 to 50.0ms−1 in a distance of 180 m. If the acceleration is constant, how much time elapse while the auto moves this distance?
A6.0 sB4.5 sC3.6 sD7.0 s
A man takes a step forward with probability 0.4 and backward with probability 0.6. Find the probability that at the end of 5 steps, he is one step away from the starting point.
View SolutionA man takes a step forward with probability 0.4 and backward with probability 0.6. The probability that at the end of eleven steps he is just one step away from the starting point, is
View SolutionA man takes a step forward with probability 0.4 and backward with probability 0.6. The probability that at the end of eleven steps he is just one step away from the starting point, is
View SolutionA drunked person is 10 step away from a manhole. Suddenly he begins to walk towards the manhole. Suddenly he begins to walk towards the manhole. He walks two steps forward and one step beckward in one second. Find out
(i) The time after which the person will fall into the manhole?
View SolutionA car starts moving on a straight road. It makes the entire journey in three parts. The total time of journey is 25 s. For first part it accelerates at a constant rate 5m/s2. For second part it moves with a constant speed. For the third part it retards at constant rate 5m/s2 to come to rest.
Let t1 be the time for first part, t2 be the time for 2nd part then correct relation is
View Solution
