A gas (γ=1.5) undergoes a cycle of adiabatic, isobaric and isochoric processes in an order. If the volume of the gas is doubled in the adiabatic process then the efficiency of the cycle is approximately,
18%
46.4%
38.5%
9.25%
The correct Answer is:A
According to the question, we drawn the following situation,
In isochoric process, pATA=pBTB
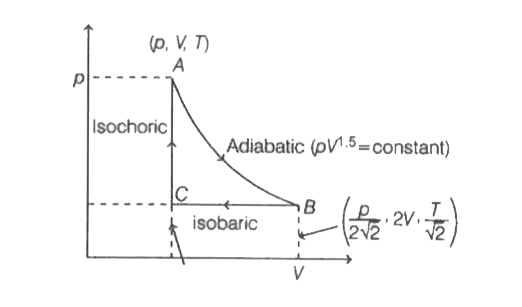
As work done in adiabatic process,
W=nR(T1−T2)y−1=R(T−T√2)(1.5−1) (Taking, n=1)
V2=2V1
⇒ T2=T1√2 or T2=T√2
Wt=RT2(1−1√2)=0.58 (∵r=i Given )
Work done in isobaric process,
W2=pΔV=nRΔT
⇒W=nR(T2√2−T√2)=−RT(1√2−12√2)=−0.35
Now, heat given to system
Q1=nCpΔT=72R(1√2−12√2)=−1.23J
Negative, Q1 shows that heat is given to the system.
Efficiency of the cycle,
η=net work doneheat input=W1+W2Q1
=0.58−0.351.23
⇒ η=0.231.23×100=18.6%=18%
Hence, the efficiency of cycle is 18%.
Topper's Solved these Questions
Similar Questions
An ideal gas with the adiabatic exponent γ goes through a direct (clockwise) cycle conisting of adiabatic, isobaric, and isochoric lines. Find the efficiency of the cycle if in the adiabatic process the volume of the ideal gas.
(a) increases n-fold ,
(b) decreases n-fold.
View SolutionIn an isobaric process (λ is adiabatic exponent of the gas)
View Solution
Knowledge Check
Two moles of an ideal gas goes under the process shown in figure. AB and CD are reversible adiabatic process.

The efficiency of the cycle isA1/4B1/2C3/4D3/5In an isobaric process (λ is adiabatic exponent of the gas)
AThe heat given to gas is λλ−1 times the work done by gas.BThe work done by gas is (λ−1) time the change in internal energy.CThe temperature of gas in increased.DThe temperature of gas is decreased.Helium gas goes through a cycle ABCDA (consisting of two isochoric and isobaric lines) as shown in figure Efficiency of this cycle is nearly: (Assume the gas to be close to ideal gas)
 A15.4%B9.1%C10.5%D12.5%
A15.4%B9.1%C10.5%D12.5%
What is the molar specific heat capacity of a gas undergoing an adiabatic process ?
View SolutionCalculate the efficiency of a cycle consisting of isothermal, isobaric, and isochoric line, if in the isothermal process the volume of the ideal gas with the adiabatic exponent γ.
(a) increased n−fold ,
(b) decreases n−fold.
View SolutionAn ideal gas goes through a cycle consisting of
(a) isochoric, adiabatic, and isothermal lines ,
(b) isobaric, adiabatic, and isothermal lines,
with the isothermal process proceeding at the minimum temperature of the whole cycle. Find the efficiency of each cycle if the absolute temperature varies n−fold within the cycle.
View SolutionWhat is the molar specific heat capacity of a gas undergoing an adiabatic process ?
View SolutionWhat is the molar specific heat capacity of a gas undergoing an adiabatic process ?
View Solution
