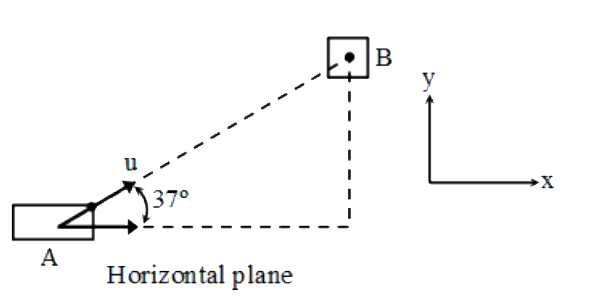
A helicopter on flood relief mission, flying horizontally with a speed u at an altitude H, has to driop a food packet for a victim standing on the ground. At what distance from the victim should the packet be dropped? The victim stands in the vertical plane of the helicopters's motion.
To solve the problem of determining at what distance from the victim the food packet should be dropped from a helicopter flying horizontally, we can follow these steps:
Step 1: Understand the scenario
The helicopter is flying horizontally at an altitude H with a speed u. When the food packet is dropped, it will take some time T to reach the ground. During this time, the packet will also move horizontally due to the helicopter's speed.
Step 2: Calculate the time of flight T
The time T taken for the packet to fall from height H can be calculated using the second equation of motion for free fall:
H=12gT2
Rearranging this equation to solve for T:
T2=2Hg
T=√2Hg
Step 3: Calculate the horizontal distance d
During the time T, the horizontal distance d that the packet travels can be calculated using the formula:
d=u⋅T
Substituting the expression for T from Step 2:
d=u⋅√2Hg
Step 4: Determine the distance from the victim
Since the victim is directly below the helicopter's flight path, the distance from the helicopter to the point where the packet should be dropped can be found using the Pythagorean theorem:
Distance=√H2+d2
Substituting d from Step 3:
Distance=
⎷H2+(u⋅√2Hg)2
This simplifies to:
Distance=√H2+2Hu2g
Final Answer
The distance from the victim at which the food packet should be dropped is:
Distance=√H2+2Hu2g
The helicopter is flying horizontally at an altitude H with a speed u. When the food packet is dropped, it will take some time T to reach the ground. During this time, the packet will also move horizontally due to the helicopter's speed.
The time T taken for the packet to fall from height H can be calculated using the second equation of motion for free fall:
H=12gT2
Rearranging this equation to solve for T:
T2=2Hg
T=√2Hg
During the time T, the horizontal distance d that the packet travels can be calculated using the formula:
d=u⋅T
Substituting the expression for T from Step 2:
d=u⋅√2Hg
Since the victim is directly below the helicopter's flight path, the distance from the helicopter to the point where the packet should be dropped can be found using the Pythagorean theorem:
Distance=√H2+d2
Substituting d from Step 3:
Distance=
⎷H2+(u⋅√2Hg)2
This simplifies to:
Distance=√H2+2Hu2g
The distance from the victim at which the food packet should be dropped is:
Distance=√H2+2Hu2g
Topper's Solved these Questions
- REST AND MOTION : KINEMATICSBOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Objective -1
13 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Objective -2
9 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer
13 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Question for short Answer
14 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Questions for short Answer
21 Videos
Similar Questions
A helicopter is flying horizontally at an altitude of 2km with a speed of 100ms−1 . A packet is dropped from it. The horizontal distance between the point where the packet is dropped and the point where it hits the ground is (g=10ms−2)
View SolutionA fighter plane flying horizontally at an altitude of 1.5 km with speed 720 km h−1 passes diretly overhead an anti-aircraft gun. At what angle from the vertical should the gun he fired for the shell with muzzle speed 600 m s−1 to hit the plane. (Take g = 10 m s−1)
View Solution
Knowledge Check
A fighter plane flying horizontally at an altitude of 1.5km with speed 720kmh−1 passes directly over head an anticraft gun.
At what angle from the vertical should the gun be fired from the shell with muzzle speed 600ms−1 to hit plane.
At what minimum altitude should the pilot fly the plane to avoid being hit ? ( Take g= 10 ms−2).Asin−1(13) , 16kmBsin−1(23) ,16 kmCcos−1(13) 16mDcos−1(23) ,16m
A helicopter rises from rest on the ground vertically upwards with a constant acceleration g. A food packet is dropped from the helicopter when is at a height h. The time taken by the packet to reach the ground is close to [ g is the acceeration due to gravity] :
View SolutionA level flight olane flying at an altitude of 1024 ft with a speed of 240 ft/s is overtaking a motor boat travelling at 80 ft/s in the same direction as the plane. At what horizontal distance before the boat should a bag be dropped from the plane in order to hit the boat ? [g=32 ft/s2 ]
View SolutionA food packed is dropped, from a height of 500 m, from a plane flying horizontally with speed 100km/h. The packet reaches to the ground after travelling a horizontal distance. [ Take g =10ms−2]
View SolutionA food packet is dropped from a plane going at an altitude of 100 m. What is the path of the packet as seen from the plane? What is the path as seen from the ground? If someone asks what is the actual path, what will you answer?
View SolutionA food package was dropped from an aircraft flying horizontally. 6 s before it hit the ground, it was at a height of 780m, and had travelled a distance of 1 km horizontally. Find the speed and the altitude of the aircraft.
View SolutionAn enemy plane is flying horizontally at an altitude of 2 km with a speed of 300ms−1 An army man with an anti-aircraft gun on the ground sights hit enemy plane when it is directly overhead and fires a shell with a muzzle speed of 600ms−1. At what angle with the vertical should the gun be fired so as to hit the plane ?
View SolutionA man throws a ball of mass m from a moving plank (A). The ball has a horizontal component of velocity u=3√34ms−1 with respect to ground towards another man standing on stationary plank (B), as shown in the figure. The combined mass of plank A with man is 2m each. The x-y plane represents the horizontal plane which is frictionless. If initially, plank A was moving with speed uˆi with respect to ground, then the relative velocity of plank B (in m s−1) with respect to plank A (after the man on plank B catches the ball) minus 10ms−1 is
View Solution
